Short circuit behavior in very complex circuits (First Order RL and RC circuit problems)

Question

I do know that a resistor is by passed when parallel to a short circuit or wire with no branches. However what if it was within a complex circuit and not just in the outer loop how does that by-passed resistor affect the other multiple resistors connected in series/parallel with it.

I end up with having 0 results in finding my i(t), it must be because i considered the entire right part to the current source as short thus shorting everything else. I lack knowledge in short circuits and how they affect COMPLEX CIRCUITS. i know how it affects it in a simple basic way but not when its already so complicated and connecte dwith varieous branches.

which of the following happens? 1, 2, 3 those are the possibilities ive come up with, im not sure which is the right one, is it 2?

Answer 1

Habitually redraw schematics

The first thing I do before attempting to analyze a circuit is to redraw that circuit. The process of just doing it helps me think and gather up a few details that I may not notice so easily otherwise, just staring at someone's depiction. But it can often help readability. And that, too, improves understanding and reduces chances for mistakes, later. (And helps others who may look at what you produce.)

It does take lots of practice to accumulate a good sense about it. But that practice is well worth your time.

For more, see the Addendum at the end, below.

Use the schematic editor

There's another reason in this case for redrawing your schematic. There are no part numbers in your pasted picture. And since you are asking for communication with others, it helps a lot if things are uniquely identified so that the communication can be specific and more terse, saving space and reading and interpretation time for all. Please also get into the habit of using the schematic editor here -- especially if your picture doesn't have unique part numbering.

It just saves time for all and it is the considerate thing to do when asking others for their help.

Your schematic, redrawn

Switch closed:

^{simulate this circuit – Schematic created using CircuitLab}

Can you now work out your answer from this? Would Thevenizing the Norton source, \$I_1\$ and \$R_1\$, help? Do you also see that \$R_1\$ and \$R_4\$ are in parallel and can be combined right away? What happens after a long time with \$L_1\$? The current would be constant, yes? And if so, then no time-dependent change anymore and therefore no voltage across \$L_1\$? What does that imply for \$R_3\$?

The above helps figure out the initial conditions for \$L_1\$.

Switch open:

^{simulate this circuit}

Once open, the subsection on left is no longer connected and isn't involved in further analysis. But given that you now know the initial conditions of \$L_1\$, you can readily work out the time-dependent behavior after the switch opens up. I would imagine now that \$R_3\$ appears to be in parallel with the series combination of \$R_2\$ and \$R_4\$. Not so?

Redrawing Schematic Addendum

Rules to live by are:

• Arrange the schematic so that conventional current appears to flow from the top towards the bottom of the schematic sheet. I like to imagine this as a kind of curtain (if you prefer a more static concept) or waterfall (if you prefer a more dynamic concept) of charges moving from the top edge down to the bottom edge. This is a kind of flow of energy that doesn't do any useful work by itself, but provides the environment for useful work to get done.
• Arrange the schematic so that signals of interest flow from the left side of the schematic to the right side. Inputs will then generally be on the left, outputs generally will be on the right.
• Do not "bus" power around. In short, if a lead of a component goes to ground or some other voltage rail, do not use a wire to connect it to other component leads that also go to the same rail/ground. Instead, simply show a node name like "Vcc" and stop. Busing power around on a schematic is almost guaranteed to make the schematic less understandable, not more. (There are times when professionals need to communicate something unique about a voltage rail bus to other professionals. So there are exceptions at times to this rule. But when trying to understand a confusing schematic, the situation isn't that one and such an argument "by professionals, to professionals" still fails here. So just don't do it.) This one takes a moment to grasp fully. There is a strong tendency to want to show all of the wires that are involved in soldering up a circuit. Resist that tendency. The idea here is that wires needed to make a circuit can be distracting. And while they may be needed to make the circuit work, they do NOT help you understand the circuit. In fact, they do the exact opposite. So remove such wires and just show connections to the rails and stop.
• Try to organize the schematic around cohesion. It is almost always possible to "tease apart" a schematic so that there are knots of components that are tightly connected, each to another, separated then by only a few wires going to other knots. If you can find these, emphasize them by isolating the knots and focusing on drawing each one in some meaningful way, first. Don't even think about the whole schematic. Just focus on getting each cohesive section "looking right" by itself. Then add in the spare wiring or few components separating these "natural divisions" in the schematic. This will often tend to almost magically find distinct functions that are easier to understand, which then "communicate" with each other via relatively easier to understand connections between them.
• You get to choose exactly one node and call it "ground." If the purpose of redrawing the schematic is for understanding it, then choose a node that helps achieve that. When signals are single-ended, they share a common node and you should select this common node as "ground." If the purpose is for analysis, then you can select this for the purpose of reducing the equation complexity. Often, this will mean the node that is "busiest" (has the most terminals attached to it.) Either way, make this choice wisely and it will help a great deal.

The above rules aren't hard and fast. But if you struggle to follow them, you'll find that it does help a lot.

You can read a snippet of my own education by those schematic draftsmen at Tektronix who trained me by reading here.

Answer 2

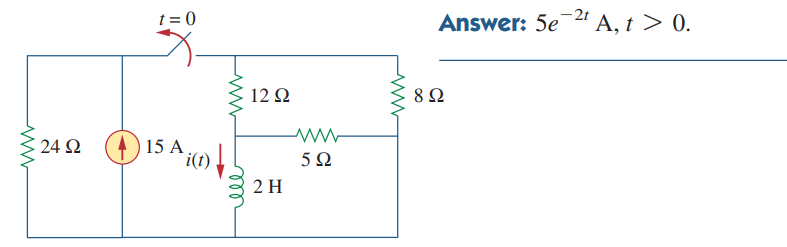
Regarding two-terminal devices in parallel with each other, you have this arrangement, where each impedance (even when the impedances are inductive or capacitive, resistive or any combination thereof) represented by \$Z\$:

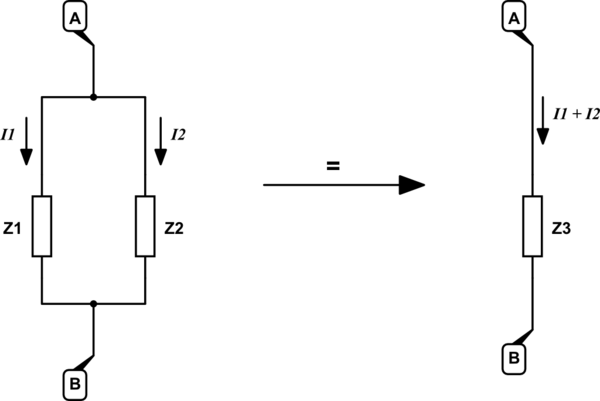
^{simulate this circuit – Schematic created using CircuitLab}

$$ \begin{aligned} \frac{1}{Z_3} &= \frac{1}{\frac{1}{Z_1} + \frac{1}{Z_2}} \\ \\ Z_3 &= \frac{Z_1 Z_2}{Z_1 + Z_2} \end{aligned} $$

Clearly, if either impedance is zero, or in other words is a short circuit across the other, then the combined impedance is also 0Ω, and the pair can be replaced by a simple zero-ohm path:

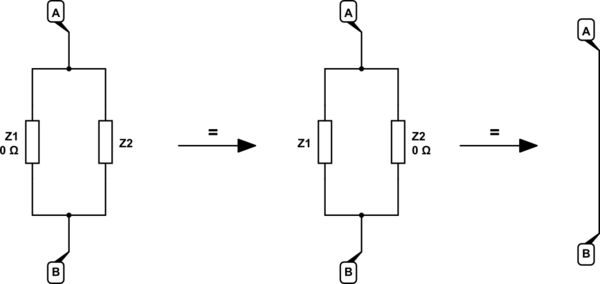
^{simulate this circuit}

This principle applies to any short-circuited two-terminal device, or compound circuit with only two externally accessible terminals:

^{simulate this circuit}

Theoretically (and in practice too, if you can somehow produce a true zero-impedance short-circuit), a zero-ohm path can have no voltage across it. If the short circuit impedance is \$Z_1=0\$:

$$ \begin{aligned} V_{AB} &= I \times Z_1 \\ \\ &= I \times 0 \\ \\ &= 0 \end{aligned} $$

Since both parallel elements (the short-circuit \$Z_1=0\$ and the element being shorted \$Z_2 \ne 0\$) have the same voltage across them, there can be no current passing through the non-zero impedance \$Z_2\$, by Ohm's law:

$$ \begin{aligned} I_2 &= \frac{V_{AB}}{Z_2} \\ \\ &= \frac{0}{Z_2} \\ \\ &= 0 \end{aligned} $$

Therefore the element being shorted circuited has:

• No voltage across it
• No current through it (all current passes via the short circuit)

Consequently, any application of Kirchhoff's voltage or current laws to that shorted device will yield a zero term, and disappear from the algebra entirely. In other words, the element can be removed completely (leaving just the short-circuit path) without affecting the state or behaviour of the entire circuit at all.

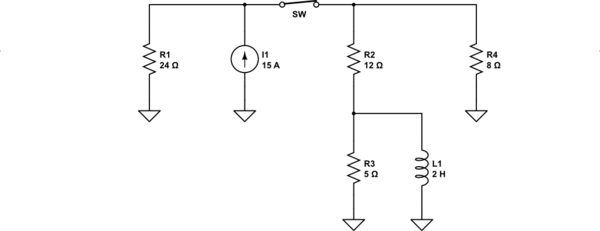
Knowing all that, your circuit can be reconstructed, in a step by step manner, removing any short-circuited components one by one. Start by shorting the inductor here:

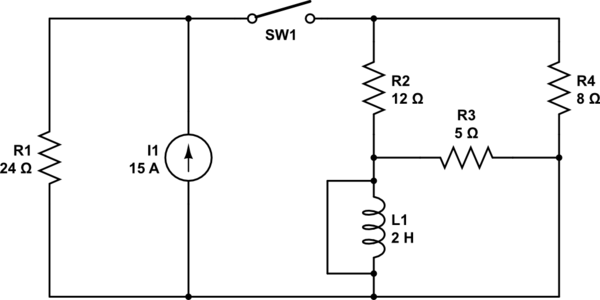
^{simulate this circuit}

Remove the inductor, leaving only the short circuit path:

^{simulate this circuit}

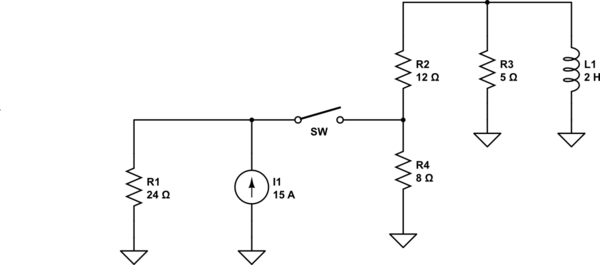
Notice that R3 is now also short-circuited, so remove that too, leaving only the short-circuit path in place:

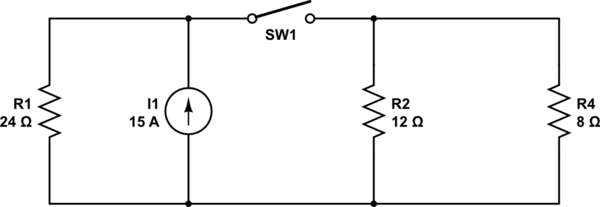
^{simulate this circuit}

Notice how R2 an R4 are now in parallel and can be replaced by a single resistance. I won't do that here, you get the idea.

Proceed like this until you have a minimal circuit, then perform your analysis.