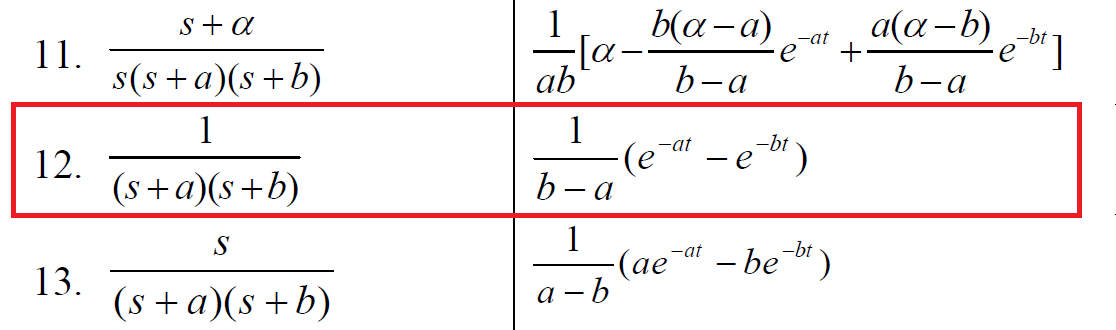Inverse Laplace transform not giving correct result

Question

How does one calculate the inverse Laplace transform of \$V(s) = \frac{1}{(s+α)(s+β)} \$? Laplace transform of function $$V(t) = \frac{1}{β-α}(e^{-αt} - e^{-βt})$$ is $$V(s) = \frac{1}{(s+α)(s+β)}$$If I try to do inverse Laplace transform of \$V(s) = \frac{1}{(s+α)(s+β)}\$ to get \$V(t) = \frac{1}{β-α}(e^{-αt} - e^{-βt})\$, I'm stuck at integral expansion where the integral doesn't evaluate to become the function V(t). I get the integral expansion with terms such as Ei which is called exponential integral I got with the help of Wolframalpha. Unless the terms as \$\frac{Ei}{2πj}\$ under limits σ - j∞ to σ + j∞ in integral expansion become unity, the result is not V(t).

I tried to calculate inverse Laplace transform by using the formula below:

$$V(t) = \frac{1}{2πj}\int_{σ-j∞}^{σ + j∞}e^{st}V(s) \mathrm{ds} $$

Answer 1

Well, we can use the convolution property of the Laplace transform:

\begin{equation} \begin{split} \mathscr{L}_\text{s}^{-1}\left[\text{F}_1\left(\text{s}\right)\cdot\text{F}_2\left(\text{s}\right)\right]_{\left(x\right)}&=\int\limits_0^x\mathscr{L}_\text{s}^{-1}\left[\text{F}_1\left(\text{s}\right)\right]_{\left(\sigma\right)}\cdot\mathscr{L}_\text{s}^{-1}\left[\text{F}_2\left(\text{s}\right)\right]_{\left(x-\sigma\right)}\space\text{d}\sigma\\ \\ &=\int\limits_0^x\text{f}_1\left(\sigma\right)\cdot\text{f}_2\left(x-\sigma\right)\space\text{d}\sigma \end{split}\tag1 \end{equation}

Now, notice that we want to find:

\begin{equation} \begin{split} \text{y}_{\left[\alpha\space,\space\beta\right]}\left(x\right)&=\mathscr{L}_\text{s}^{-1}\left[\frac{1}{\left(\text{s}+\alpha\right)\left(\text{s}+\beta\right)}\right]_{\left(x\right)}\\ \\ &=\int\limits_0^x\mathscr{L}_\text{s}^{-1}\left[\frac{1}{\text{s}+\alpha}\right]_{\left(\sigma\right)}\cdot\mathscr{L}_\text{s}^{-1}\left[\frac{1}{\text{s}+\beta}\right]_{\left(x-\sigma\right)}\space\text{d}\sigma\\ \\ &=\int\limits_0^x\exp\left(-\alpha\sigma\right)\cdot\exp\left(\beta\left(\sigma-x\right)\right)\space\text{d}\sigma\\ \\ &=\int\limits_0^x\exp\left(\beta\left(\sigma-x\right)-\alpha\sigma\right)\space\text{d}\sigma\\ \\ &=\int\limits_0^x\exp\left(\left(\beta-\alpha\right)\sigma-\beta x\right)\space\text{d}\sigma\\ \\ &=\frac{1}{\beta-\alpha}\int\limits_{-\beta x}^{-\alpha x}\exp\left(\text{u}\right)\space\text{du}\\ \\ &=\frac{1}{\beta-\alpha}\cdot\left[\exp\left(\text{u}\right)\right]_{-\beta x}^{-\alpha x}\\ \\ &=\frac{1}{\beta-\alpha}\cdot\left(\exp\left(-\alpha x\right)-\exp\left(-\beta x\right)\right)\\ \\ &=\frac{\exp\left(-\alpha x\right)-\exp\left(-\beta x\right)}{\beta-\alpha} \end{split}\tag2 \end{equation}

Where I used the table of selected Laplace transforms.

Answer 2

How does one calculate the inverse Laplace transform of \$V(s)=\frac{1}{(s+α)(s+β)}\$?

Engineers use Laplace transform tables such as this document and item 12 below: -

But, if you want to do it the hard way then convert to partial fractions: -

$$\dfrac{1}{(s+a)(s+b)} = \dfrac{p}{s+a}+\dfrac{q}{s+b} = \dfrac{p(s+b)+q(s+a)}{(s+a)(s+b)}$$

Solve for p and q: -

$$1 = p(s+b)+q(s+a)$$

By substituting firstly for \$s = -b\$ and then for \$s = -a\$ and you get: -

$$p = \dfrac{1}{b-a}\text{ and } q = \dfrac{-1}{b-a}$$

Hence...

$$\dfrac{1}{(s+a)(s+b)} = \dfrac{1}{b-a}\left[\dfrac{1}{s+a}-\dfrac{1}{s+b} \right] $$

Solving the inverse Laplace of \$\frac{1}{s+a}\$ is trivial and even the most basic inverse Laplace table will give you the answer of \$e^{-at}\$.

Answer 3

What is the problem?

It is "obvious" with Maple ...