The opamp here is ideal except for the bias currents. In order to calculate the maximum absolute voltage deviation at the output, I was thinking of sum the deviation of Vos and deviation of current, being that the deviation of current, for the maximum is R2*Ib (I think.)
My problem is for the deviation of Vos because in the exercise description, they give Ios (so I did the Ohm laws to get Vos) and then calculate the deviation for Vos with gain*Vos, but then I got the wrong answer in the end. What am I doing wrong?

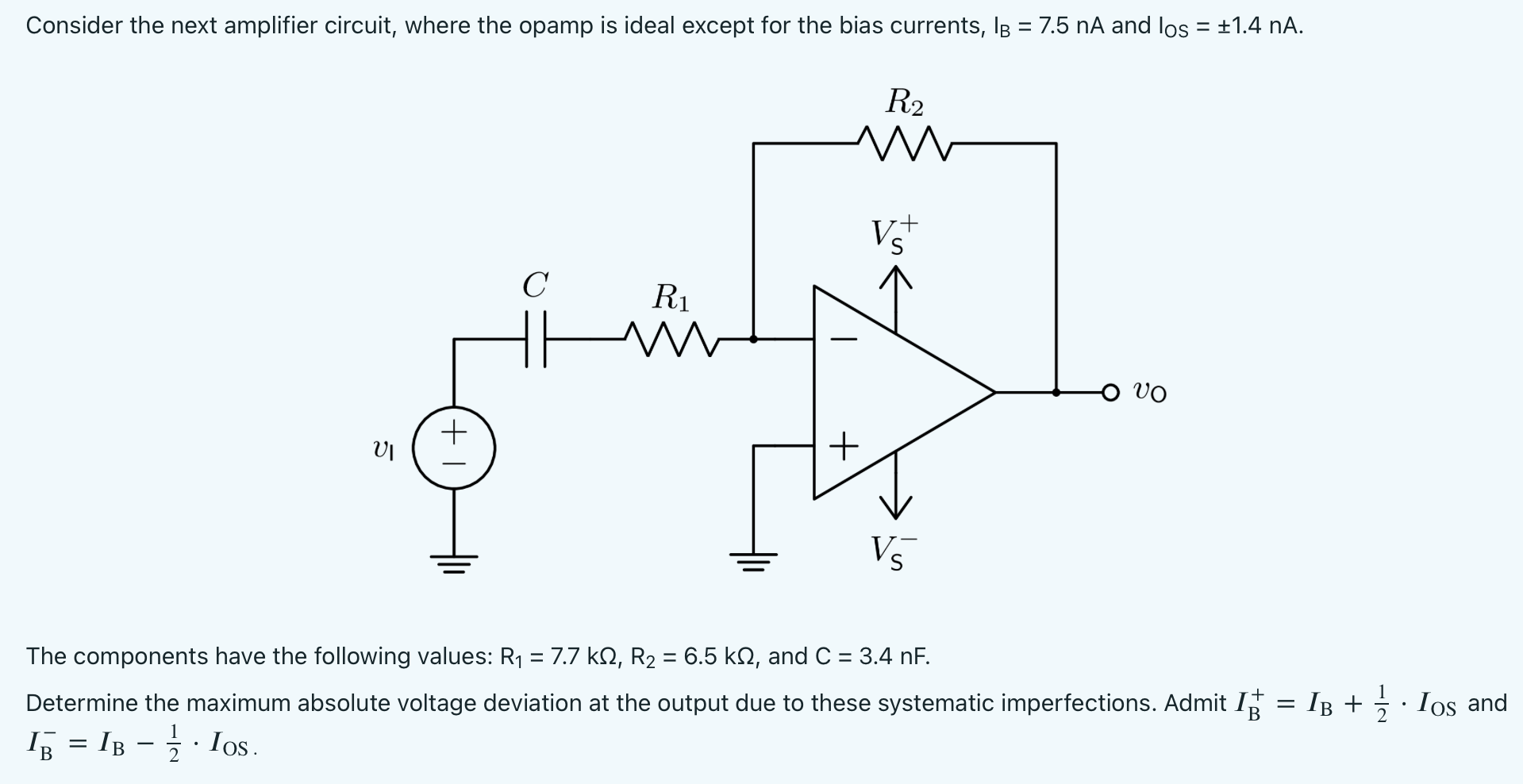
\$I_{b+} = 7.5\text{ nA}+\dfrac{1.4\text{ nA}}{2}\$\$\endgroup\$