Regarding a composition of casual system (cascading them)
The definition of a causal system is that:
A system \$F\$ is causal if for any signals \$x_1\$, \$x_2\$ and any time \$t_0\$ we have that \$x_1(t)=x_2(t)\$ for all time \$t\$ up to \$t_0\$ implies that \$F(x_1(t))=F(x_2(t))\$ for all time \$t\$ up to \$t_0\$.
Where the \$x\$'s are input signals to the system/circuit \$F\$ that generates the output signal \$F(x_1)(\cdot)\$.
Now, if you have two causal systems \$F\$ and \$G\$ and compose them you obtain another system \$H(\cdot) = F(G(\cdot))\$. This system will be causal, and to show it we start with:
- (1) for any signals \$x_1\$, \$x_2\$ and any time \$t_0\$ such that \$x_1(t)=x_2(t)\$ for all time \$t\$ up to \$t_0\$
Since \$G\$ is causal, statement (1) implies that:
- (2) \$G(x_1)(t)=G(x_2)(t)\$ for all time \$t\$ up to \$t_0\$
Since \$F\$ is causal, assumption (2) implies that:
- (3) \$F(G(x_1))(t)=F(G(x_2))(t)\$ for all time \$t\$ up to \$t_0\$.
Since \$H(\cdot) = F(G(\cdot))\$, from statement (1) and the implication chain up to (3) we have that: for any signals \$x_1\$, \$x_2\$ and time \$t_0\$ such that \$x_1(t)=x_2(t)\$ for all time \$t\$ up to \$t_0\$ we have that \$H(x_1)(t)=H(x_2)(t)\$ for all time \$t\$ up to \$t_0\$. So we do have that \$H\$ is causal.
Examples
Given two causal systems \$F\$ and \$G\$ we can show that \$F+G\$, adding the output of the systems, and \$F \star G\$, the convolution of the outputs of the systems, will also be causal.
First we show that the system \$A\left(\begin{bmatrix}x_1 \\ x_2\end{bmatrix}\right) = x_1 + x_2 \$, adding the inputs at each instant, is causal. That should be intuitive since the output at any instant is just the sum of the inputs at that same instant.
The convolution normally used in circuits analysis and control systems is the one-sided convolution of two signals, it is defined as
$$ (x_1 \star x_2)(t) = \int_{-\infty}^t x_1(\tau) x_2(t-\tau) d\tau. $$
Notice that it only uses values/information of the two signals \$x_1, x_2\$ from before or at time \$t\$, so it will be causal. Lastly, define \$B\left(\begin{bmatrix}x_1 \\ x_2\end{bmatrix}\right) = x_1 \star x_2\$.
The compositions \$A\left(\begin{bmatrix}F(x_1) \\ G(x_2)\end{bmatrix}\right)=F(x_1)+G(x_2)\$ and \$B\left(\begin{bmatrix}F(x_1) \\ G(x_2)\end{bmatrix}\right) = F(x_1) \star G(x_2)\$ will be causal from all the systems being causal and the argument that composing/cascading causal systems leads to another causal system.
Regarding feedback loops
The analysis above does not hold for a feedback system, as the feedback loop, first and foremost, creates also the problem of the loop being well-posed or not. An example of an ill-posed system would be
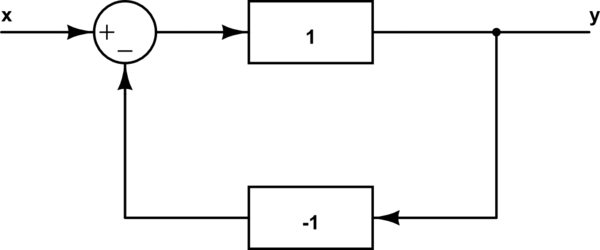
simulate this circuit – Schematic created using CircuitLab
and the issue becomes clear once we write the equations that "relate" the input to the output
$$ x(t)+y(t)=y(t) $$
which would imply that \$x(t) = 0\$, the input having to always be zero!
I couldn't find any simple source on why well-posed causal linear systems are causal, but here is a non-rigorous sketch:
- A linear system \$A(x+By) = y\$ (the plant is \$A\$, the transfer function on the feedback loop is \$B\$, and the summing junction is adds the feedback to the input) will be well-posed if the system \$(I-AB)\$ is invertible (for any signal \$y\$, the whole output signal \$y\$ leads to a unique input signal \$Ax\$ such that \$Ax = (I-AB)y\$.
- From linearity we have that for any signals \$x_1\$, \$x_2\$ and any time \$t_0\$ such that \$x_1(t)=x_2(t)\$ for all time \$t\$ up to \$t_0\$, the signals \$y_{x_1}\$ and \$y_{x_2}\$ can only differ up to time \$t_0\$ on the nullspace of \$(I-AB)\$, but that system is invertible so the nullspace of \$(I-AB)\$ will be just the zero signal (a signal that is zero for any time \$t\$).
- We have that \$y_{x_1}\$ and \$y_{x_2}\$ are equal up to time \$t_0\$.
- This all leads to the linear system made of causal systems with a well-posed loop being causal, that is, it holds that
for any signals \$x_1\$, \$x_2\$ and any time \$t_0\$ with \$x_1(t)=x_2(t)\$ for all time \$t\$ up to \$t_0\$, we have that \$y_{x_1}=y_{x_2}\$ up to time \$t_0\$
For in-depth discussion on the challenges to determine if the feedback of nonlinear systems is well-posed I can only refer to
https://doi.org/10.1109/CDC.1987.272730 and https://doi.org/10.1109/9.40742 which were the only papers I could find on the topic.


