Typical ripple current limits of C0G capacitors

Question

I notice that manufacturers don't tend to publish ripple current limits or dissipation factors for C0G capacitors in shunt operation like you'll see more commonly with class 2 dielectrics (X5R, X7R, etc.). I've also not been able to find good numbers on package thermal resistances and impedances. Naturally they publish |Z|, ESL, and ESR plots, along with S parameters, but that doesn't tell me how much ripple current they can take before self-immolating, and I'm left guessing with rules of thumb. I'm also mostly interested in this in the context of switching regulators, and most specsheets don't provide numbers for <10MHz when it comes to C0G. However, modern C0G caps are getting to have surprisingly high capacitance density, so we're reaching the point where one could select C0G over X7R for a buck output cap in some niche scenarios.

I know that normally the DF is so low on ceramics that you can mostly ignore it until you're pushing a few amps, and a lot of it is going to come down to the thermal properties of the package (and thus simply the size), but I'm mostly familiar with these generalities from barium titanate dielectrics rather than calcium zirconate.

What's a good way to approach quantifying the ripple current limits of C0G capacitors? Are there any good rules of thumb for what's very likely fine vs. what's very likely not going to fly? Preferably ones that don't involve me buying a bunch of capacitors and subjecting them to ripple currents until they catch fire. As noted, I'm mostly interested in the context of switching regulators, where ripple is present in the range of 500kHz to a few MHz.

Answer 1

I notice that manufacturers don't tend to publish ripple current limits

Kemet do a lot of the time. So, if you want to know about KC-link capacitors (suitable for higher power converters and resonant converters) go to their site. I've recently used this Ceramic, 0.022 uF, 5%, 1000 VDC, C0G, SMD, MLCC, Ultra-Stable, Low Loss, Class I, 2220 on a job and the link has the RMS current and voltage rating across a broad range of frequencies: -

So, if you really want to exploit the ripple current in a C0G capacitor, the ones that are very suitable for this type of application have decent data available.

most specsheets don't provide numbers for <10MHz when it comes to C0G

The one above goes down to 100 Hz and tops-out at around 10 GHz. All this information is available in the specsheet. In other words, they are prepared to release this information on paper so that your personal quality assessments of components are facilitated.

What's a good way to approach quantifying the ripple current limits of C0G capacitors? Are there any good rules of thumb

Find the right component that has suitable specifications and is supplied from a reputable manufacturer (as per above). I don't work for Kemet and I'm not affiliated with them them.

Answer 2

Here's the estimation process I use.

Consider a resistor of the same chip size, and its rating. The capacitor will tend to be thicker, and the material, lower thermal conductivity than the Al₂O₃ used in resistors.

Taking half the power rating of an equivalent chip resistor, seems safe enough.

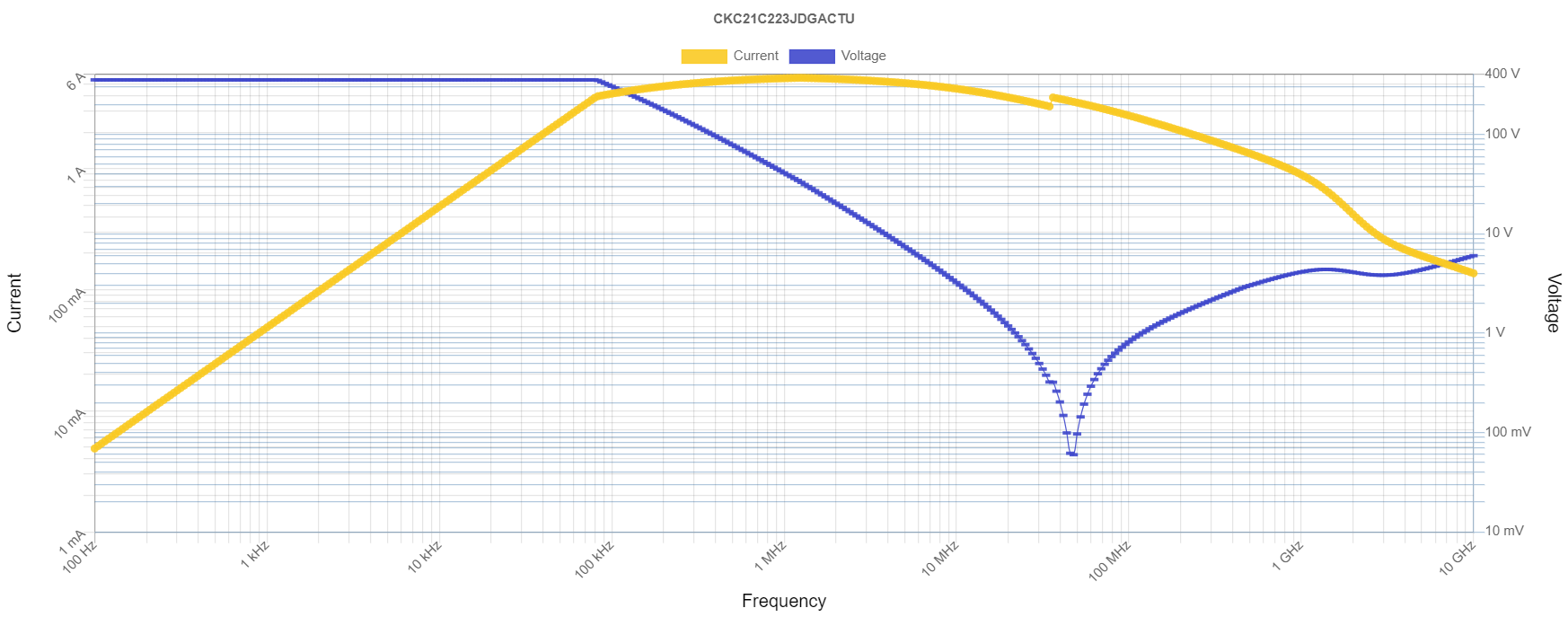
Then consider the ESR or Q. ESR is generally extremely low, to the point it can almost be ignored (which is a sometimes perverse thing already: for example, 10nF C0Gs placed side-by-side in parallel will resonate with each other, with the mere 5nH or so loop inductance between them!). Obviously for ripple current purposes, we must still take stock of that figure, and express the tolerable voltage or current vs. frequency.

Generally this suggests rated voltage (AC if given, or DC peak-to-peak), up to some 100s kHz at least, if not MHz still, and then dropping off above there as the current limit takes over.

There will be some additional derating at high frequencies (1s to 10s MHz onward) where skin effect reduces effective cross-section of the device (basically, think in terms of the solid metal end-caps shielding current flow to inner layers, thus ESR rises as \$\propto\sqrt{f}\$). This should be evident from the ESR as given; just a reminder that it's not perfectly straight asymptotes defining the operating limits.

You'll have a damned hard time violating a rating like that, where low ripple is required, as in a DC supply filter. This does suggest some potential optimizations, like using a C1 || (L+C2) motif to put a notch in the transfer function: the LC multiplies the ripple voltage from C1 onto C2, making C2 many times more effective -- but only near the resonant frequency, so this is only applicable to fixed-frequency converters where the notch(es) can be placed on Fsw and harmonics. And obviously you need a high-Q inductor to do that; but, why not just make a better filter overall using a poorer quality L with bulkier C's instead?

This is in part why type 2 dielectric are most popular at low voltages, and (still fairly boutique, but attractive when size is higher priority than cost) poled electrets at high voltages (~500V DC link, etc.).

Type 2 (normal, un-poled) are poor at high voltages for a couple of reasons: ceramic in general get expensive when you need bulk values and energy storage; and high-voltage types have fewer fatal dielectric defects, meaning they can handle a much higher electric field strength: which is to say, the C(V) curve you get on a, say, 250V part, is wildly more depressed than that of a 16V part -- you just can't get parts of any reasonable value-density.

But where applicable, the relatively low ripple of a DC filter means lossy types like type 2 ceramic, electrolytics, and powdered-iron inductors, are quite acceptable.

On top of that, you generally need losses in DC filters anyway, to make them insensitive to source/load impedance variations, which makes lossy types further attractive.

Somewhat unrelated, but handy in general: think of polymer electrolytics as low-voltage film caps. They're available in a range of ESRs, but the lowest are very comparable to DC-link type polypropylene film caps, scaled for low voltages. (Films don't scale to low voltages, because the film has to be so thin and wide, it's impossible to get adequate yield. Kinda C0G has the same problem below 100V or so, hence values like 100nF are "large" for it.)

Answer 3

This isn't really a full answer, but it felt like too much to put in comments, so:

Anecdotally, I've been able to push a few tens of amps RMS through good C0Gs in a high-power RF system. They got quite toasty, to the point that I actually ended up putting heatsinks on capacitors, something I've never done before or since, but they did survive. These were 2225 size capacitors; derate by size. Note that this was more stressful a use case than what you're talking about, as the capacitors were actually completely charged (to a few hundred volts), discharged, and reverse charged every cycle, so any losses related to that--though minor--would come into play as well. (In this application, any class II dielectric would have exploded in short order. Class I dielectrics are much more linear and thus non-hysteretic, but still not, I think, perfectly so, so there possibly could be some tiny amount of loss here.)

It's worth noting here that the dissipation factor for calcium zirconate is very nearly zero, especially at the low frequencies you're talking about. Losses will be dominated by the resistance of the plates themselves; for processing reasons, these plates are usually made of nickel, but you can get special ultra-low-ESR capacitors with copper plates (I know Knowles makes such; Johanson Dielectrics might too). They do cost more; evaluate whether it's worth it in your application.

Basically, I've always treated C0Gs as essentially just surface-mount high-temperature film capacitors in terms of characteristics, and it hasn't steered me wrong yet.

Answer 4

Murata supplies more information than most any other manufacturer with their simsurfing site and app. You can use that information to estimate heating (see other answers) but there is no ripple current spec. There's a temperature rise tab on simsurfing, but it is grayed out for all the large C0G caps that I checked.

Rather they suggest that you measure the heating and check that it is less than 20°C (possibly derating if your maximum Ta is very high).

Applicable to Rated Voltage of less than 100VDC

The load should be contained to the level such that when measuring at atmospheric temperature of 25°C, the product's self-heating remains below 20°C and the surface temperature of the capacitor in the actual circuit remains within the maximum operating temperature.