simulate this circuit – Schematic created using CircuitLab
From what I understand, the magnitude and direction from an independent current source is constant. What would happen in the circuit above? If currents flow they way they're supposed to from the current source, KCL is violated. So how would the circuit above behave?
How about this one?
In circuit 1 you get infinite voltages and in circuit 2 you get zero current.
EDIT due to wrong circuit posted in question - circuit 2 takes 45mA and I'm not inclined to feed the reasons on a plate to the OP because this sort of stuff should be reasoned through.
In circuit 2, each resistors pin has the same voltage (V1 = V2 = 1V). Since there is no voltage difference between these resistors pins, there is also no current flowing through them.
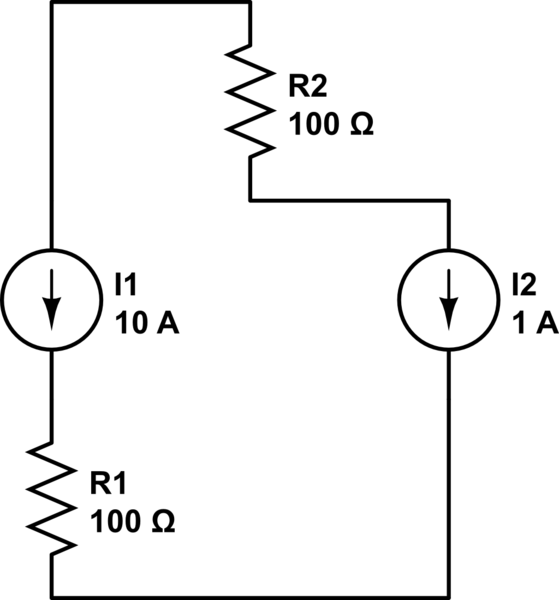
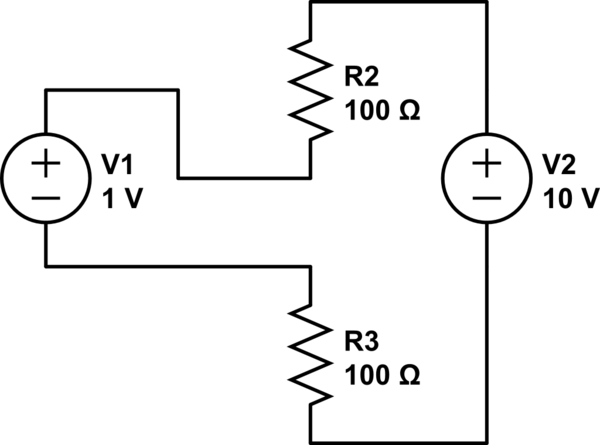
As stated in an earlier comment the first circuit is not solvable because its a simple series circuit and therefore must have the same current everywhere It can't be both +10A and -1A.
Now we come to an analysis of the second circuit.
It is also a series circuit so must have the same current everywhere.
From Kirchhoff's voltage law we know that if you follow any closed loop all the way round the voltages must sum to zero.
$$10 \text{ volt} + I \cdot 100 \ \Omega - 1 \text{volt} + I \cdot 100 \ \Omega = 0 \text{ volt}$$
$$9 \text{ volt} + I \cdot 200 \ \Omega = 0 \text{ volt} \Rightarrow I = - \frac{9}{200} = - 45 \text{ mA}$$
The minus sign just implies that the current is in the opposite direction to the one I originally guessed.
Working from the bottom of the diagram up the bottom node is 0V the voltage at the top of the bottom resistor is \$ 0.045 \text{ A} \cdot 100 \ \Omega = 4.5 \text{ volt}\$
The voltage at the bottom of the top resistor is \$ 4.5 \text{ volt} + 1 \text{ volt} = 5.5 \text{volt}\$ with 10 volt at the top node with a current of 45mA circulating counter clockwise around the loop.
I will just boil this down to a couple of fundamentals:
You can see that your first circuit creates a contradiction between these two rules, because you have ideal current sources in series and their specified currents are different. This means there is something wrong with your model. Either there are alternate paths for current to travel that aren't shown in the model, or the current sources are not ideal.
The dual of those rules are:
An ideal voltage source might have any current through it that's required to be consistent with the specified voltage.
Your second circuit doesn't create any contradiction because the voltage sources aren't placed in parallel. The net result will just be a current flowing in to the positive terminal of V1, which is no problem for an ideal voltage source (but might be a problem for some kinds of real voltage sources).
