Current step to LC circuit

Question

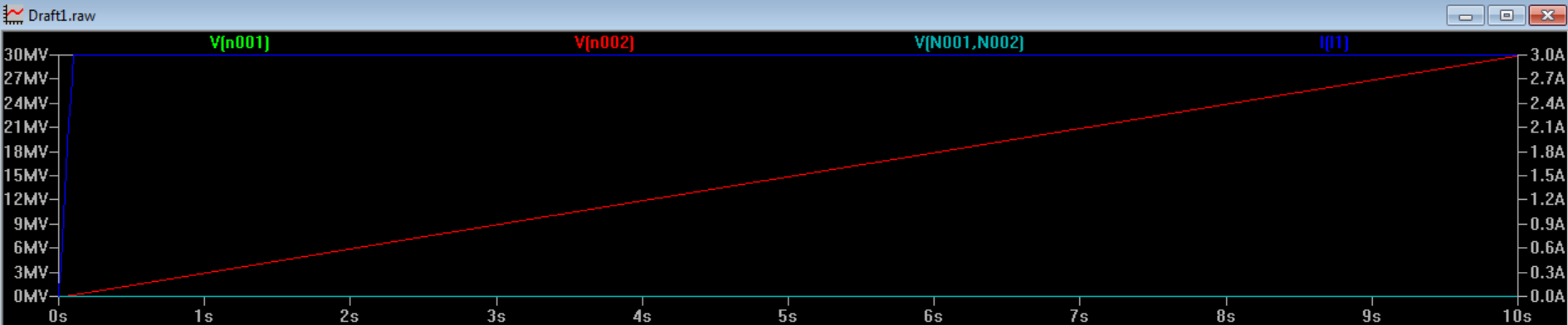
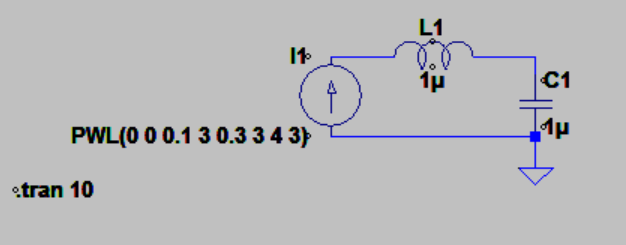
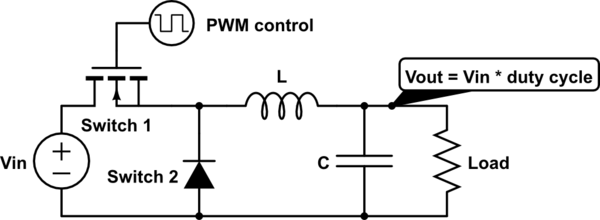
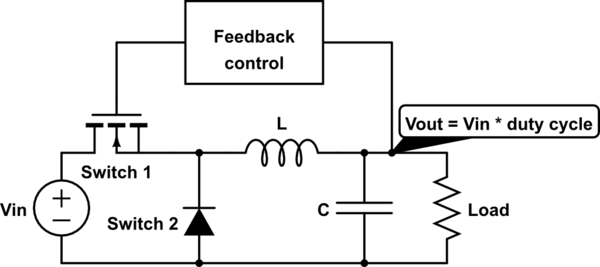
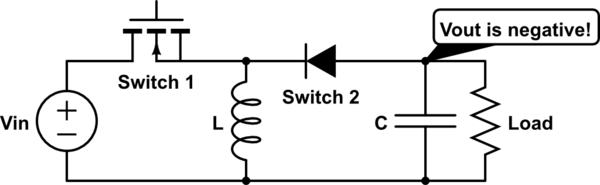
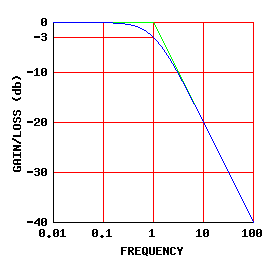
An inductor is a device which adjusts its voltage to keep the same current flowing through. However, the current exponentially decays when there is no source any more. Similarly, a capacitor is a device which keeps the voltage across itself constant. However when charged, the voltage exponentially decays when a load is connected. THe building block of a buck convertor seems to be the L and C. However I am having a hard time understading how an LC circuit actually operates(in a buck). I understand that the capacitor is kind of a snubber capacitor to allow current to flow through and provide a path when the high side PMOS is turned on. However, that job could as well have been done by an resistor, given that Voltage mode control is being used. The only advantage I see to using a capacitor is the efficiency, since energy is not wasted. I applied a current step to my inductor in LTSPICE and this is what I got:

I understand that initially, the voltage at the left hand side of the inductor should actually try to become 0 to allow no current to pass(the initial state). However this doesnt seem to be the case. Can someone explain intuitvely what exactly is going on in the LC circuit. How should it be analyzed?

Answer 1

A buck converter is basically a pulse width modulated (PWM) voltage in series with a low-pass filter. The filter's output is (ideally) the DC average value of the PWM voltage:

^{simulate this circuit – Schematic created using CircuitLab}

The PWM voltage is generated by switching a DC input voltage. When switch 1 is on, switch 2 is off, and vice-versa:

^{simulate this circuit}

The switching is commonly done with a MOSFET and a diode. The MOSFET is controlled by a PWM signal, which can be produced by an analog circuit (using a sawtooth wave and a comparator) or a digital circuit (using a counter):

^{simulate this circuit}

The low-pass filter could be an R-C filter, but that would waste a lot of power. An L-C filter is used instead because LC filters are (ideally) lossless:

^{simulate this circuit}

Finally, a feedback control system is added to compensate for component variation and improve the transient performance of the converter:

^{simulate this circuit}

And that's a buck converter. A common variation is the synchronous buck converter, which replaces the diode with another MOSFET to reduce conduction losses. Practical converters may have other features such as overload protection or soft start-up.

In a buck converter, the inductor is part of an L-C filter. But it's sometimes more helpful to think of it as an energy relay, especially when looking at other converter topologies. The basic idea is that when the MOSFET is on, the input voltage source stores energy in the inductor. When the MOSFET is off, the inductor releases that energy into the load. This is easiest to see in a buck-boost converter, where the input source and the load are never directly connected:

^{simulate this circuit}

In all cases, the capacitor is there to smooth the output voltage. The inductor current is not constant, so without the capacitor the output voltage would vary during the switching cycle. (In buck and buck-boost converters, the inductor isn't even always connected to the load!) It's not a snubber since there's always a current path for the inductor.

Answer 2

Can someone explain intuitvely what exactly is going on in the LC circuit. How should it be analyzed?

Analyse it in the frequency domain because it IS a filter: -

For frequencies up to about "1" the output is largely the same as the input. For higher frequencies, the output rapidly gets smaller as frequency rises.

For instance, between 10 and 100 the output amplitude drops by 40 dB and we can make a pretty reasonable approximation and uses the green line in the graph above. If we use the green line we can say: -

• From DC to 1 the output is constant
• From 1 to 100, the output has dropped by 80 dB

So, using a practical example let's say the buck regulator is switching at 100kHz and the filter is set to have a 3dB point at 1kHz. The average value of the switching waveform is the DC voltage we want at the output. But we get some noise from the switching as well. If the switching waveform were 10Vp-p we could make a reasonable argument to say this would get attenuated by 80 dB compared to low frequencies.

80 dB attenuation of 10Vp-p is 1mVp-p so, with a 10Vp-p square wave on the input, the output will be a dc value superimposed with a few millivolts of ripple. The DC output is of course the average value of the switching waveform and, if the duty cycle is 50% then the average value will be 5V.