Why do only thick wires have less resistance?

Question

I have learned that the thicker a wire is, the less resistance it has. However, this is not the case for other things such as a wall. If a (non-metal) wall is thicker it does not have a lower resistance. Why is this?

Answer 1

Resistance in a wire can be defined as

$$R = \frac{\rho L}{A} $$

where

\$ \rho \$ = resistivity

\$ L \$ = Length

\$ A \$ = cross sectional area

Thicker gauge wires have a larger A, and therefore the resistance of the wire decreases keeping everything else constant.

If you are asking about non metallic objects, than they might not be conductive (very high \$ \rho \$), and so their resistance would be extremely high. If the object is conductive, then the \$ \rho \$ of that material would play a factor in its overall resistance.

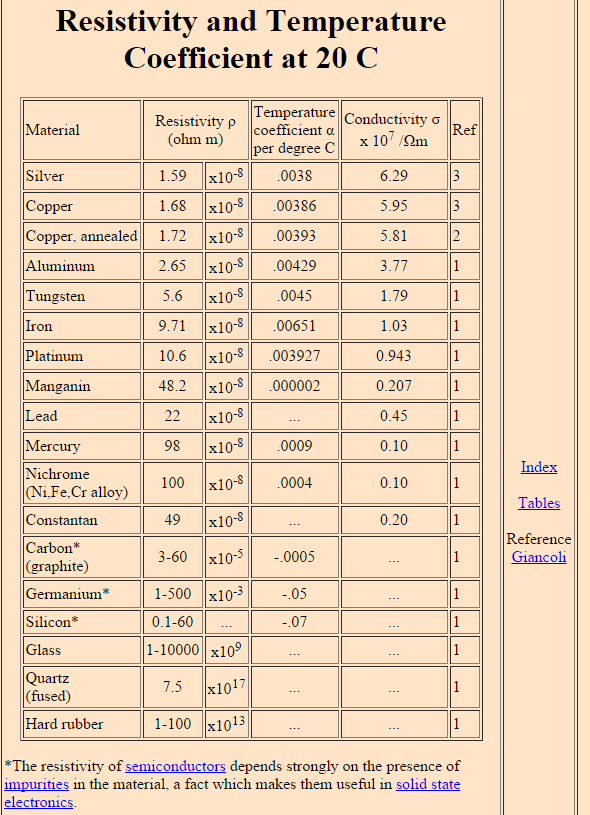
Below is an image that shows the resistivityof various types of meterial. Rubber is not considered to be conductive and look at its resistivity compared to copper which is conductive.

Source for image

Answer 2

Because it's not the thickness of the object, it's the thickness of the electrical path. A thicker wall does not increase the thickness of the path but instead increases its length, thereby increasing resistance.

Answer 3

How much electrical resistance does ANY wall have? Think of the wall as a very short and very fat conductor. It happens to have VERY high resistivity, so its resistance will be very high, but if you double the size of the wall, its resistance will be halved. Maybe still very large but halved.

So, your question is somewhat confused, because you are comparing two materials (metal in a wire and a wall that does not conduct much, at all) that are grossly different. So different that the material difference totally obscures the question about conductor cross-section.

Answer 4

Cross-sectional area is not the only property resistance depends on. It also depends on the nature of material through which you are trying to pass current. The nature of the material of a metallic wire is such that it contains free electrons. These free electrons drift in a perticular direction when a voltage is applied across the wire causing current in the wire. Where as a wall is made up of bricks, cement etc which donot contain free electrons to conduct electricity hence a wall's resistance to current is much higher than that of a metallic wire.

Answer 5

Consider that there are circumstances where thicker conductors don't have significantly lower resistance. See skin effect and proximity effect. When winding high frequency transformers, litz wire is used because of those effects.

Answer 6

Resistance behaves the same geometrically regardless of the material. The problem is one of the specific terminology used in the context of walls and wires.

Usually 'thicker' in the context of a wire means a lower gauge which means a larger diameter wire. However, for a well, thickness simply means the depth, or how, well, thick it is.

To put things in equivalent terms, 'thicker' in the case of the wire actually means wider. And thicker in the case of the wall would mean longer in wire-speak.

And indeed, regardless of the exact material the wall is made from, a very wide and tall wall will have lower resistance than narrower and shorter wall, if the thickness of both is the same.

If you imagine the wall is made from copper, you will find this is the same behavior as with a wire. A larger diameter wire is wider and taller in the cross-section, and likewise has lower resistance.

But why?

One can do a very, very deep dive on the physical mechanisms behind resistance and conductivity. But to keep things simple (and classical/non-quantum), imagine that electrons, when moving through a material, behave more like waves that propagate between the atoms of the material (at least if that material is a metal).

This is fine as long as the atoms of this material are arranged in a perfectly regular lattice or grid with no irregularities.

But if we introduce defects in this repeating crystal structure, or worse, impurities of entirely different atoms, then some electrons will propagate as waves that will cause wave interference with other electrons as they all pass through the material.

This is the wave analog of a collision. And the result is largely the same - a loss of momentum which is converted into heat. One might also think of it as being analogous to friction.

This is also why temperature has such a noticeable impact on resistance - higher temperature means the atoms the electrons are moving between are jiggling around more, causing yet more small irregularities that make electrons interfere slightly with each other.

Austere ampere

So why would a wire (or wall) of a larger cross section result in lower resistance? This isn't going to make the atomic level structure of the material more free of defects or anything. It's just the same old stuff as far as an electron is concerned.

Well, we need to think about what the definition of current actually is.

An ampere is defined as 1 coulomb per second. And a coulomb refers to a specific (and very large) number of individual electrons. So an amp is, ultimately, just saying 'this many electrons must pass through through here in one second'.

Note that our definition ignores everything about the actual geometry of 'here'. 'Here' could be a hair-thin length of magnet wire, or a 50mm square bus bar used to power one Pentium IV. The definition of current doesn't care. As long as the right number of electrons pass through a given cross-sectional slice of something, it counts as (and is exactly equivalent to any other) an amp of current.

But now consider the mechanics of making a fixed number of electrons pass through those two very differently sized conductors.

Electron marathon

Imagine a wide running track, and it is holding an electron marathon. These marathons are extremely popular, so pretty much every single space on the track is filled with electrons participating.

The goal of this marathon is for 100 electrons to cross the an arbitrary line (let's call it the "finish line" even though it isn't really a race) every second. This is a "faux ampere" (with a real amp being the same, only with far more electrons - around 6,241,509,074,000,000,000 electrons crossing the finish line per second).

If the track is wide enough to fit 100 electrons side-by-side, then the electrons don't really have to go very fast. As long as they move so just one electron length of distance is made each second, then multiply that by 100 of them side-by-side, and you get our single faux amp requirement.

But now, let's imagine a track that can only fit two electrons side-by-side. Now they're going to get a work out. For 100 electrons to cross the finish line per second, they have to cover a distance of 50 electron lengths per second. In other words, they have to run 50 times faster to achieve the same current!

But more importantly, this means the electrons will have to effectively cover 50 times more distance per second.

Resistance is the distance, resistivity is the friction

The resistivity of materials is constant - it doesn't change with geometry. This can be thought of as a measure of how 'hard' it is for the electron to move through that material, or how much friction it will experience as it moves through it.

Resistance, on the other hand, can be thought of the total distance an electron will have to move through a material for a given amount of current.

Narrower cross sections require electrons move faster for a given current, and thus they will have to cover more distance per second through that material. Twice as fast means twice the distance means twice the resistance even if the friction/resistivity stays the same.

With a wide cross section, the electrons can move much more slowly and cover less distance for a given amount of current, resulting in less resistance.

This is no different for a metal wire or a rock wall. Material doesn't really matter. A rock wall will probably have fairly high resistance, but it will still be less with a larger cross section of that material when compared to a smaller cross section of that same material.

That was a lot to get through for something seemingly so simple, but I think this is well-helped by some good visual analogies.

Oh, and that is really what happens - electron drift velocity is determined by the current and cross section conducting it. They really do move faster through narrower cross sections vs wider ones for the same current. And as a result, the momentum/energy they lose to friction as heat will be proportionally more.