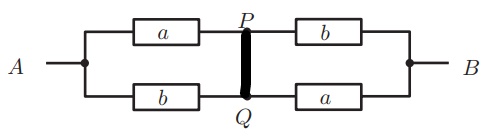
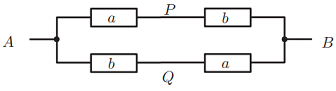
Using only intuition, without mathematics, why can't the total resistance in the circuit of the 1st image be less than the total resistance in the 2nd image?
The 2nd circuit gives more path options for a current to flow, but I don't see why this could result in less resistance than the 1st circuit.