Voltage drops not adding up - beginner question

Question

I'm fairly new to electronics and playing around with a very simple series circuit - 2 resistors of ~220k Ohm and a 3v power supply, which I'm measuring with a digital multimeter.

If I measure the voltage across both resistors (or direct across the power supply terminals) I get a reading of 3.37 volts, but if I take a reading across the individual resistors I get 1.656 and 1.676 V (adding up to 3.332). I'm wondering what would account for the difference in total voltage? Even if the multimeter wasn't calibrated properly I'd expect it to be consistent?

Thanks!

Answer 1

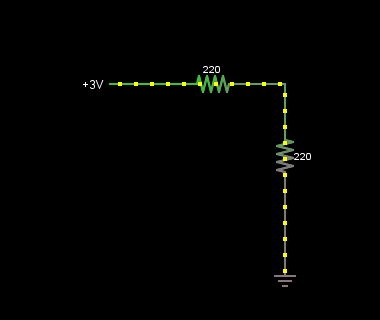
I'll assume this is the circuit you`re testing

Usually a voltmeter (PMMC) type:

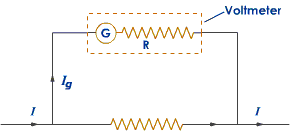
Is a galvanometer with a HUGE series resistance (Mega ohm), such that a very small amount of current pass through it whenever this voltmeter is connected in parallel with a resistance

For the circuit shown

Voltage source is 3.3 V

Current = 0.0075 A

Load resistances = 220 Ω each

The voltage drop across each of the 220 Ω is 1.65 V = (0.0075 * 220)

BUT when you connect your voltmeter now

Assuming that: R:The voltmeter resistance = 10 MΩ And you want to measure the drop across one of your resistances

so you'll now connect your voltmeter in PARALLEL with one of these 220 Ω resistances

Now you`re adding a parallel resistance with your load of value 10 MΩ

So the current is now not 0.0075 A since the circuit equivalent resistance is no longer the two series 220 Ω resistances only

its a combination of two parallel resistance 220 Ω,10 MΩ and a series resistance 220 Ω such that the equivalent resistance is now 439.9951 Ω (Almost less than 440)

so you'll not have the same amount of current (0.0075 A) but a slightly more amount of current in this case (0.00750008249 A) Almost 0.0075!

this in turn affects the voltage drop across each resistance by a very very small value that we usually neglect

So you'd expect a slightly smaller reading than the calculated value

Other factors also affects your reading such as

• The source output resistance

• Wire losses

Answer 2

A typical voltage meter has about 10 MOhm input resistance, which you have to account for in your calculation. The measurement error is about 2%.

Answer 3

There are several sources of error in your measurements. One is the input resistance of the meter. Another is the full scale calibration error. We also have offset error and nonlinearity errors. Offset is normally negligible with modern meters and nonlinearity is pretty low for most (not all) meters. Nonlinearity for a 7106-based 3.5 digit meter is typically +/-0.2 count. I'll assume you have such a meter.

So, a good first order approximation of the error in reading a voltage with source impedance RS is Vr = Vx * (Rin/(Rin+Rs)*(1+a) where |a| << 1 is the full scale error for a particular range and Rin similarly is the input resistance for a particular range. In your case, two equal resistors of value R connected to a stiff voltage source, the source resistance is R/2 for the measurements across each resistor or 110K\$\Omega\$.

Looking at the total of the two measurements of closely equal resistors we have:

Vt ~= Vx * Rin/(Rin+Rs)*(1+a1). The measurement of the total is just

Vx = Vx * (1 + a2)

So Vt/Vm = Rin/(Rin+Rs)*(1+a1)/(1+a2).

To put some numbers on these errors, if the input resistance is 10M and the error on the 2V scale is -0.2% and the error on the 20V scale is +0.2%, the ratio would be 0.985. If measured on the same scale the error would be 0.989, ignoring the +/- 0.5 count uncertainty in each measurement. Your number was 3.37/3.332 =0.989, so if the assumptions were correct you got lucky in using the two different ranges- the error could have been much higher.