3
\$\begingroup\$

So I thought the ripple voltage was approximated by the formula

Vr,pp = Vp / fRC

for a half-wave rectifier, and Vp/2fRC for a full-wave.

Yet simulation shows about 55% of this value.

So is the formula wrong? Just very inaccurate? Or under what conditions is it otherwise more accurate?

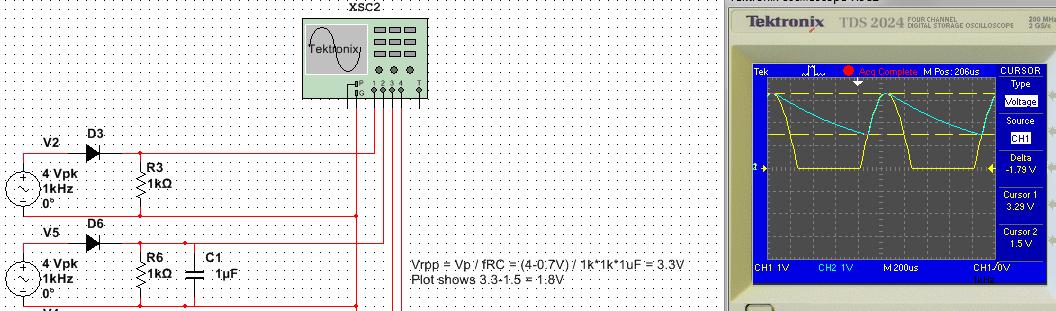
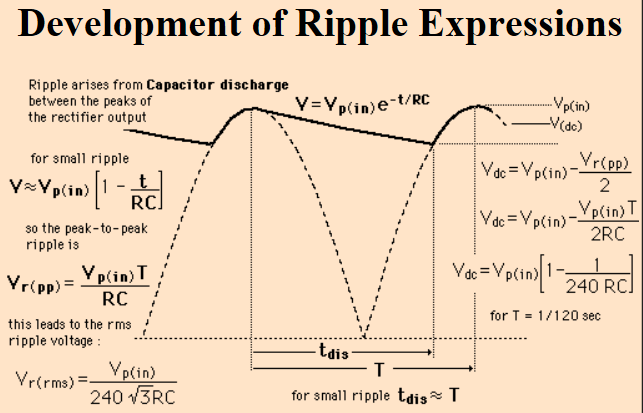
enter image description here

\$\endgroup\$
1
  • 2
    \$\begingroup\$ It only really applies to ripple that is small relative to the DC voltage. The assumption is that the conduction period of the diode is small and the current through the load is essentially constant. Your example does not meet either of those constraints. Try increasing the capacitor to 10uF and look at it then. \$\endgroup\$
    – Kevin White
    Commented Nov 9, 2016 at 23:41

3 Answers 3

Reset to default
4
\$\begingroup\$

There is a mass of online help to aid calculating this. This one at Hyperphysics is particularly complete with a calculator provided.

enter image description here

\$\endgroup\$
2
\$\begingroup\$

The OP's original post has formulae reversed. The ripple for half-wave rectification is larger than that for full wave.

Easy to think about: if you charge a cap with a half a wave of voltage and current, it will discharge less if you have another charging half wave right after the first, and more if you have to discharge the cap another half wave with no input.

Ripple with full wave rectification is \$I / (2 f C)\$, and with half wave is \$I / (f C)\$.

Probably poor practice to use the \$R\$ from the filter in the equation: what matters is load current which will reflect both filter AND load resistance.

\$\endgroup\$
1
\$\begingroup\$

Remember this Rule of Thumb for % voltage ripple, using rectified frequency out f=1/τ

  • If RC = 8 τ then Vpp/Vavg ≈ 10%

  • If RC = 10τ then Vpp/Vavg ≈ 8%

  • Then \$ \frac{Vpp_{ripple}}{V_{avg}} = \frac{ I_{avg}}{I_{pk}}\$= \$\frac{discharge}{charge}\$

i.e. \${\%Vpp_{ripple}}\$ is inverse to peak diode and cap current ratio.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.