How to explain why equivalent resistance between two points in this network is dependent on specific resistance?

Question

The original network is an square network with two diagonals as you can see here there is no intersection between the diagonals.: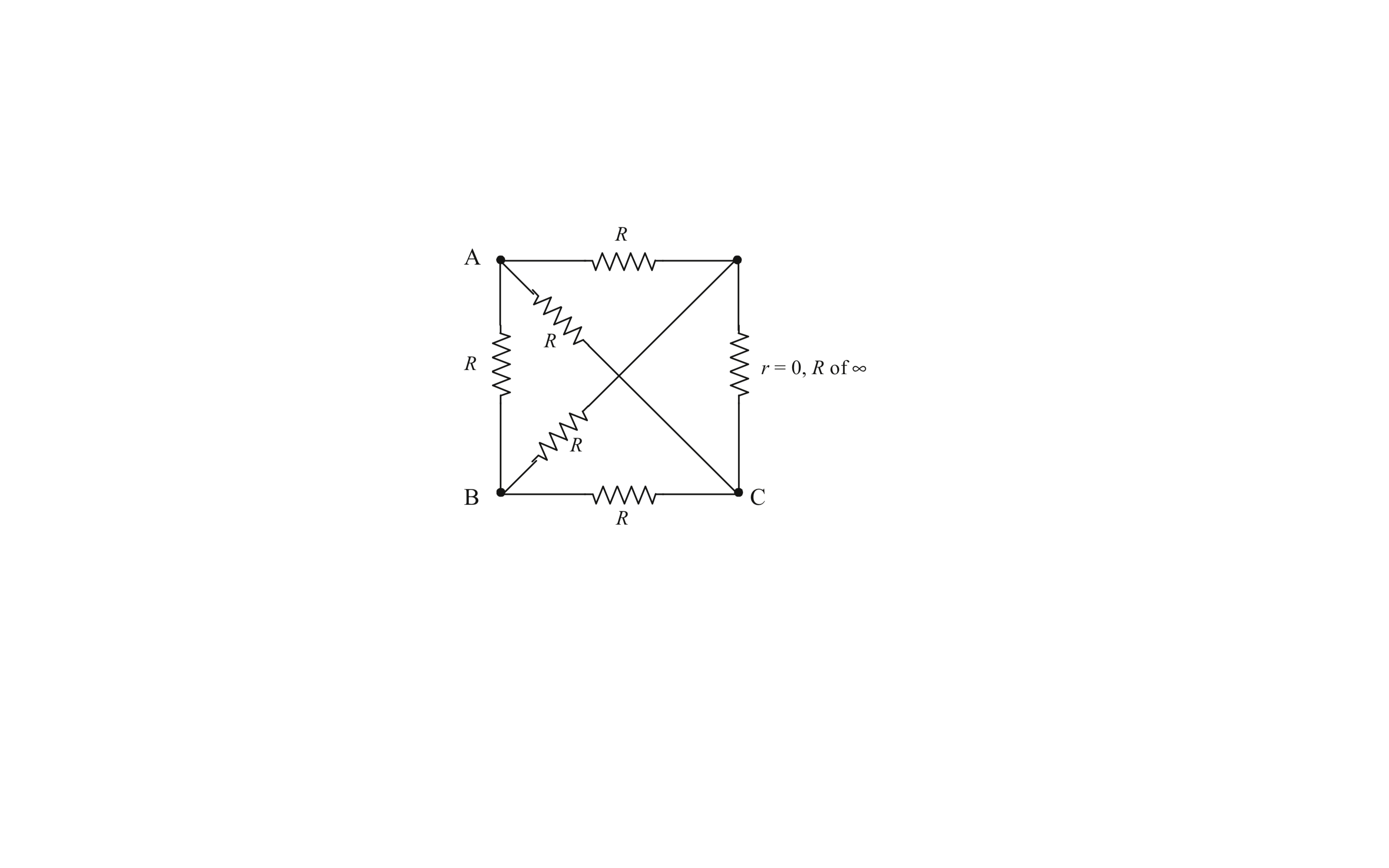

The question is what is the equivalent resistance between points A and C as wel as A and B ?

I rearranged the network to the equivalent network here under (all the resistances which labeled as '1k' below is actually 'R' in original network):

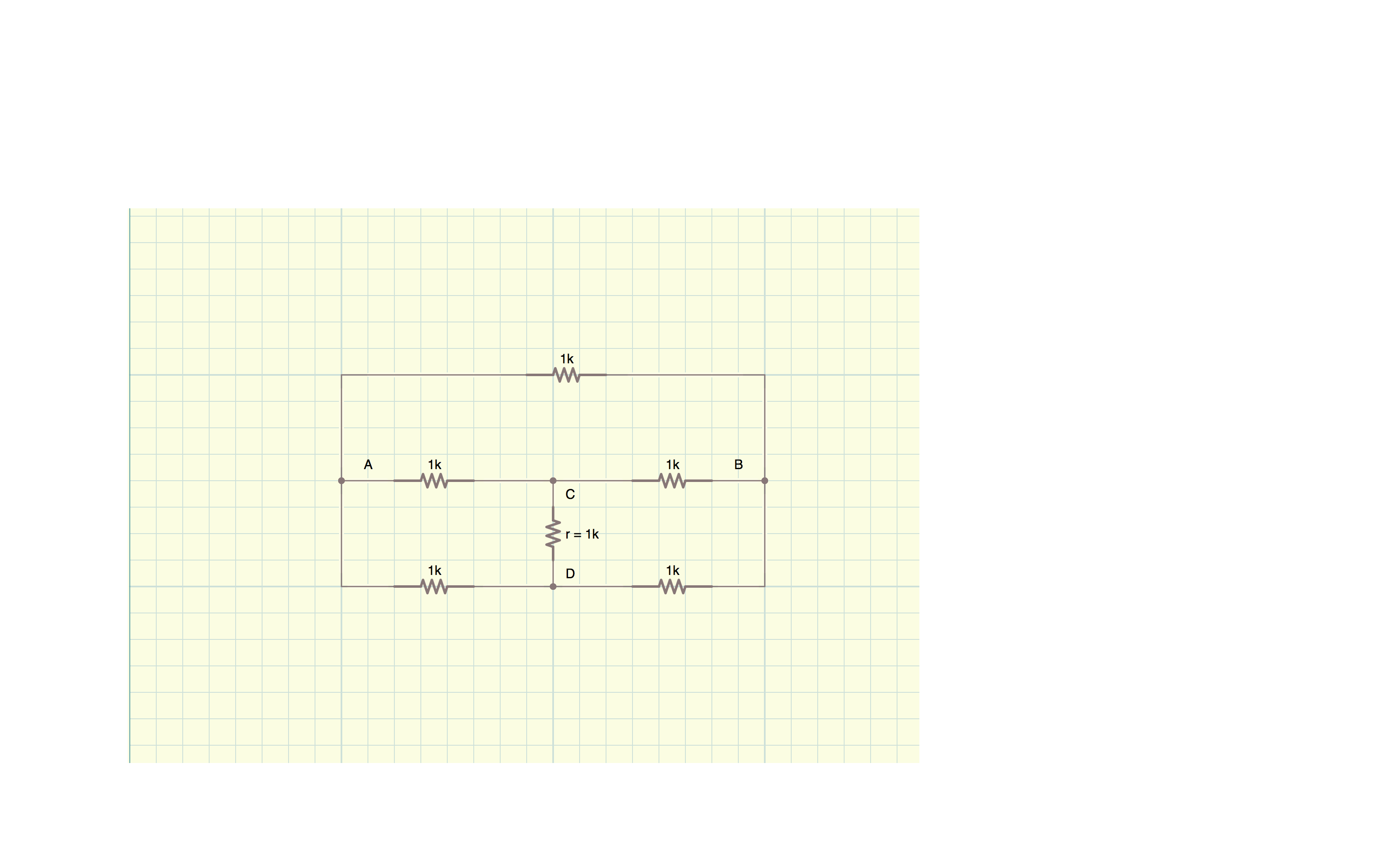
now i see the bridge of Wheatstone so the equivalent resistance is $$\frac{R}{2}$$. The bridge is in equilibrium and the current in 'r' is zero. Now i want to evaluate the equivalent resistance between the points A and C, if i take exact the same rearrangement and suppose that there is no current in resistance 'r' i would find an expression independent of 'r', but that's clearly false and i wonder why ?

The correct answer is $$\frac{R(3R+(5r)}{8(R+r)}$$. I find it by using the general methode.

Answer 1

Take the original circuit, redraw it the way you did for A and B resistance, but for the resistance between A and C, and you will see that \$r\$ is not the center resistor that holds no current, but one of the leg resistors that does carry current.

Measurement points matter.

Two ways to think about it: If you imagine the network as a system of springs, measuring the resistance between A and B is like pulling on those two points, and seeing how the system reacts. You can easily imagine \$r\$ not moving much because of how the system is laid out. But, if you pull at A and C, \$r\$ will move a lot.

Another way to think about it is to imagine you set A to ground and B at 5V, and look at where the current flows. Point C is at 2.5V, and \$r\$ has no current flowing into it. To conduct the same test between A and C, we could just hold C at 2.5V, and move B up to 1.25V. Adding voltage into B in this way changes how the currents flow through the whole system, and now we'll get current flowing through \$r\$.

Learning something like this is like juggling or riding a bike. It is easy to understand the theory, but just like you need to run your body through biking before you can get all the bits used to it, you need to run your brain through this stuff for a while, in different ways, smashing into trees and shrubs, and then it all just clicks.