Comprehension problems with op-amps

Question

The common differential amplifier takes two signals and amplifies the difference between these two signals. I am aware that this difference usually is around a few millivolts or microvolts so, to put it in engineering terms, it is zero.

Everywhere here, in the text books, or internet, the mathematical calculations are based on the fact that the voltages at the inverting and non-inverting terminals are exactly equal to each other, so conceptually we should have a zero output voltage, and that's not the point, because this circuit is going to amplify nothing.

Am I fundamentally wrong here?

Answer 1

Typical opamps have DC gains in the 10^5 to 10^6 region. This means if the output is within the rails, say 0 to 5v, or +/- 15v, then the input will indeed be measured in microvolts.

As you say, this is sufficiently close to zero to be deemed to be zero for many purposes.

One of the purposes for which input=0v is a good enough approximation is when solving for the DC gain of a fed back amplifier. Typically, several resistors will draw current from input and output voltages measured in volts, and sum their currents at one of the amplifier input terminals. Whether that terminal has a voltage of 0uV, or 10uV, is irrelevant for most purposes, as the error is parts per million.

Typically, amplifier input offset errors will be measured in mV, so for accurate systems, we have to worry about input offsets long before we have to worry about whether the input voltage is really zero or not.

For an ideal opamp, where the gain is infinite, then the input voltage is zero, theoretically.

Answer 2

First, a classic op amp amplifies the DIFFERENCE in the voltages at the + and - terminals.

First and a half, an op amp has a VERY high voltage gain.

Second, every linear circuit that uses an op amp provides a feedback path from the output to the - input.

This creates a control loop, where the whole point of the feedback path is to drive the difference between the two inputs to zero. In normal operation, the op amp in circuit actively drives that difference to zero. For analysis of the circuit, then, it is generally good enough to assume that the difference is zero.

Start with the classic noninverting buffer stage, where the input is fed to the + input and the output is tied to the - input. The only output value that will yield zero difference is Vout = V+ (the voltage at the + input).

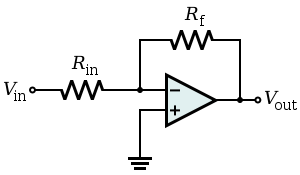
Consider the classic unity gain inverting buffer, where the + input is tied to ground, the signal is applied through a resistor to the - input, and an equal resistor ties the - input to the output. The op amp will try to drive the - input to ground. If the input signal is above ground, current must flow in the input resistor, and attempt to flow into the - input. This would induce an offset voltage across the op amp input impedance, which is amplified by the op amp, and that gives rise to an output voltage, which in turn gives rise to current through the feedback resistor. If the current in the input resistor is equal to the current in the feedback resistor, then there is no current left to induce an offset voltage at the - input, and the amp is happy.

Answer 3

All calculations are not based on zero differential input voltage, only approximate calculations. It's usually a pretty good approximation at DC if the op-amp is a precision type (high gain, low input offset voltage, and low input bias currents and offset current relative to resistor values). Often it is less than the input offset voltage (usually not with 'zero-drift' types, however).

However, if you want to account for finite open-loop op-amp gain (and for offset voltage and CMRR) you need to make a bit more complex calculation, and in any case, you have to keep in mind that the differential input voltage is only approximately zero.

Since open-loop gain drops with frequency (typically at -20dB/decade above something like 10Hz), it's not hard to have a significant closed-loop gain error at higher frequencies, especially if you have a higher frequency and/or higher ideal closed-loop gain.

Answer 4

Everywhere here, in the text books, or internet, the mathematical calculations are based on the fact that the voltage in inverting and non-inverting terminals are exactly equal to each other , so conceptually, we should have a zero output voltage, and that's not the point, because this circuit going to amplify nothing.

If you are talking conceptually (your word) then consider the concept of an ideal op-amp with infinite gain - that can then convert 0 volts into a value that is useful to be used in this rather theoretical exercise.

Answer 5

It took me a while to wrap my head around too.

Best way I found to look at it was look at a simple inverting amplifier:

The standard way to solve this circuit is to assume + and - are the same (in this case, GND) and give the opamp infinite gain. Pick some values and work it through by hand.

Then do as you suggest and use a real op-amp. Head over to CircuitLab and simulate the same circuit using a real world opamp like a 741 or some such.

Note how similar the two results are. Once you see it for yourself that way, you'll understand why the approximation works.

Yes, there is a tiny difference between the + and - terminals, but it is so small you can basically call it a short for the sake of making your calculations quick. You see this a lot in EE. A computational short cut that makes you able to do the math quickly often times does not describe the physics of exactly what is going on.

Answer 6

Zero difference between the op amp's inputs is an assumption which is useful to perform basic analysis of an op amp circuit and to achieve a useful understanding of circuit operation. In reality there is always a voltage difference between the inputs. If a situation existed where the inputs are at exactly the same voltage then, as you spotted, there would be no current out of the input stage differential amplifier, no current to the base of the output driver stage and therefore the output voltage would start to change but it would not have to change very far before feedback caused a small difference voltage at the input which is amplified by the differential input stage and voltage amplification stage which prevents the output changing any further. The output has stabilised with a small voltage difference at the input. If the output rises the input difference increases and turns the output driver on harder, if it falls the input difference decreases, the output driver reduces drive and the output rises. The output has stabilised. The output has come to rest with a small error. The higher the open loop gain of the op amp the smaller this error and the smaller the difference between the inputs. Precision op amps have high gain.

The above description is for DC. The situation for AC is a bit different. For AC, as the input to the circuit moves up and down one op amp input will oscillate about the other input causing a changing difference voltage at the inputs. The amplitude of the input difference voltage depends upon the frequency. As the frequency increases the open loop gain reduces due to the dominant pole compensation caused by the miller capacitor around the output driver stage. As the open loop gain reduces there must be a larger "error" at the output in order to create a larger difference voltage at the inputs. For DC operation this error at the output is actually an error but for AC operation the error at the output is a phase lag. So as the frequency increases the open loop gain reduces and the output phase lag increases, increasing to 45 degrees at the -3dB frequency. At this point the difference between the inputs can be quite large. Remember that the op amp's output is largely determined by the closed loop gain determined by the resistor network around the op amp. But the difference voltage at the input is determined by the open loop gain. The amplitude of the voltage at the output equals the amplitude of the input voltage difference signal times the open loop gain.