0
\$\begingroup\$

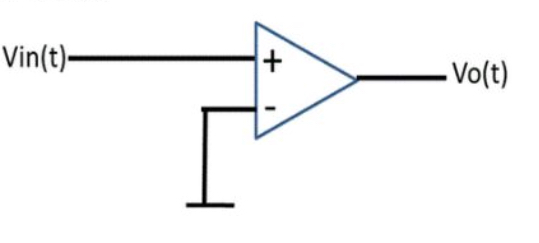
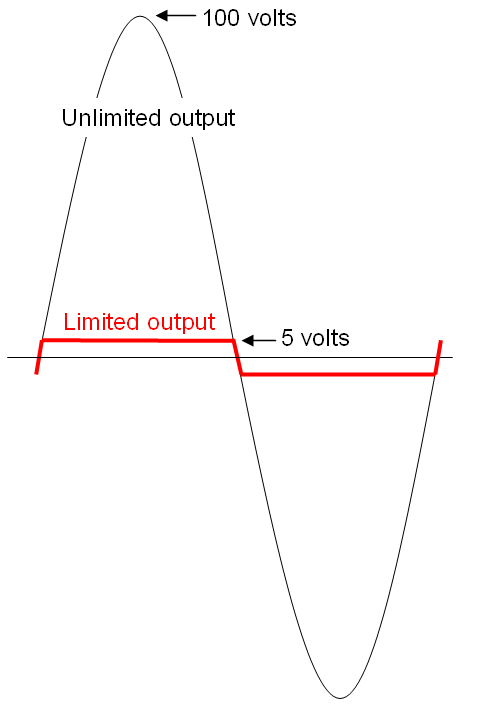
There is a given op amp circuit with an open loop gain of 100. Rest of the parameters of the op amp is ideal. The voltage rail of the op amp is +/- 5V. The input wave form Vi(t) = cos(100t).

So now the question asks to find the rise time (0-100%) of Vo(t). Can anyone please help me with this question?

enter image description here

So my attempt was

At t = 0 sec, my output should be 100xcos (0) = 100V But since my output voltage cannot go beyond +5V (Vsat), Vo = 5 V.

So I can calculate the final value, but I can’t figure out how to find the rise time. I was thinking for an ideal op amp it should be 0s. But that’s a wild guess and I’m not really sure.

\$\endgroup\$
9
  • \$\begingroup\$ You need to try and explain what you have tried to do in order to solve the problem. This site isn't a free homework answering service. \$\endgroup\$
    – Andy aka
    Commented Aug 1, 2018 at 16:25
  • \$\begingroup\$ @Andyaka Sorry was my first questions in SE. I edited it to add my attempt. Thanks for pointing it out. \$\endgroup\$
    – Saptarshi Ghosh
    Commented Aug 1, 2018 at 16:40
  • \$\begingroup\$ It doesn't matter if it's a sine or cosine waveform so, assume a sine and ask yourself how many degrees of phase must pass (from 0 degrees and 0 volts) for a sin wave with peak amplitude 100 volts to reach 5 volts. Convert that phase angle to time. \$\endgroup\$
    – Andy aka
    Commented Aug 1, 2018 at 16:45
  • \$\begingroup\$ Is the frequency response of the opamp given? This might be a Laplace Transform based question \$\endgroup\$
    – ijuneja
    Commented Aug 1, 2018 at 16:52
  • \$\begingroup\$ @ijuneja no unfortunately, this is all that’s given \$\endgroup\$
    – Saptarshi Ghosh
    Commented Aug 1, 2018 at 16:54

1 Answer 1

Reset to default
1
\$\begingroup\$

I'm not giving a full answer because the OP needs to learn.

Saying that the input is a cosine wave is confusing you. The output will have the same rise and fall times if it were a sine wave except now you can start at t = 0, whence the output waveform begins at 0 volts and 0 degrees of phase angle.

If the amplified waveform was unbounded by the +/- 5 volt rails it would reach peaks of +/- 100 volts. If you superimpose the 5 volt limits you get something very similar to this: -

enter image description here

It's a simple matter of using your brain/equipment to calculate arcsin(0.05) to give you the degrees that have passed to hit a level of 5 volts.

Can you take it from here?

\$\endgroup\$
2
  • \$\begingroup\$ *begin nitpicking* It's not just arcsin(0.05) though, the input to the sine wave was 100 t, not 1 t. *end nitpicking* \$\endgroup\$
    – Harry Svensson
    Commented Aug 1, 2018 at 17:43
  • 1
    \$\begingroup\$ @HarrySvensson arcsin(0.05) gives you degrees based on attaining 5% of the peak i.e. 5 volts in 100 volts. You've then got to convert to time by accounting for the frequency. \$\endgroup\$
    – Andy aka
    Commented Aug 1, 2018 at 18:12

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.