Infinite output impedance in amplifier, instead of 0?

Question

The question asks: "Exercise 14.5 Assume an ideal op amp and use the summing-point constraint to find an expression for the output current io in the circuit of Figure 14.14. Also find the input and output resistances of the circuit".

I'm confused about the answer for output impedance. The solutions manual says that "because io is independent of RL, we conclude that the output impedance of the amplifier is infinite." Isn't the output impedance always 0 in an ideal op amp? What do they mean by current independent of load? Wouldn't that always be the case unless there was a controlled source?

Answer 1

Isn't the output impedance always 0 in an ideal op amp?

The ideal op amp itself has zero output impedance, yes. But the output impedance of the amplifier you build with it can be anything but zero, depending on the configuration, of course. Take a look at the marked text in your question again. It clearly says "the amplifier has infinite output impedance", not the op amp.

What do they mean by current independent of load?

Basically a current source.

The circuit in your question is a form of voltage-controlled current source. As you already know, an op amp with negative feedback keeps the input terminal voltages equal. The negative feedback loop is closed by the load resistor, R_L. Since the voltage across the R_F is equal to the non-inverting terminal voltage (or the input/control voltage), the current through R_F and therefore R_L will be kept constant. So you have a current source and its magnitude is set by the input (control) voltage and R_F.

And remember, a current source has theoretically infinite output impedance.

Answer 2

This opamp is being used as a transconductance amplifier, so it doesn't work the way you normally think of an opamp working.

Assume \$V_{in}\$ is a fixed voltage 500 mV, the opamp wants to make both inputs have the same voltage so it will output enough current to cause there to be a 500 mV drop across \$R_F\$. Since the output current depends on \$V_{in}\$ and \$R_F\$, it is independent of \$R_L\$. Since \$\Delta R_L\$ has no effect on the current it's like the output impedance of the opamp is infinite.

Imagine a current source made with a very high voltage and a very high resistance in series with a relatively low resistance load.

^{simulate this circuit – Schematic created using CircuitLab}

With a 0 Ω load the current is 1 uA, with a 1k load it's 0.999999 uA, very little change. If you could make the voltage and resistance of the source infinite the current wouldn't change at all with a change in the load. So if the load doesn't affect the current the impedance must be infinite.

Answer 3

Let's treat the op-amp and its periphery as a "black box", leaving only the load resistor \$R_L\$ outside:

^{simulate this circuit – Schematic created using CircuitLab}

The "black boxes" above each have two terminals, X and Y, and you have no idea what's inside the box. Each box has some internal resistance, which we aim to measure.

We could measure it by changing the external resistance \$R_L\$, or by applying some external potential difference between X and Y, or even by forcing some current through the box. Whatever method we use, we shall measure the resulting change in voltage \$\Delta V_{XY}\$, and change in current \$\Delta I_O\$. Effective resistance \$R_{XY}\$ between X and Y inside the box will be, according to Ohm's law:

$$ R_{XY} = \frac{\Delta V_{XY}}{\Delta I_O} $$

For box B, you'd find that there's no change in voltage, since its internal voltage source will strongly oppose any change in \$V_{XY}\$, but current \$I_O\$ is able to rise and fall freely:

$$ R_{XY(B)} = \frac{\Delta V_{XY}}{\Delta I_O} = \frac{0}{\Delta I_O} = 0\Omega $$

In other words, box B (or any ideal voltage source) is said to have zero impedance.

Box C is a current source, and we would expect it to strongly oppose changes in current through it, \$\Delta I_O=0\$, while freely permitting \$V_{XY}\$ to change:

$$ R_{XY(C)} = \frac{\Delta V_{XY}}{0} = \infty \Omega $$

Ideal current sources, therefore, are said to have infinite source impedance.

Box D will have an impedance somewhere in between zero and infinity, because in this case it would be possible to impose any arbitrary voltage between X and Y, and it's also possible to inject an arbitrary current through the box. With either "perturbation" method, if you plotted \$I_O\$ on the horizontal axis, vs. its corresponding \$V_{XY}\$, the slope of that graph is \$R_{XY}\$. Just by inspection, you could predict that this slope would be:

$$ R_{XY(D)} = \frac{\Delta V_{XY}}{\Delta I_O} = 100\Omega $$

For more information, and a graphical treatment of using voltage and current changes, and the slope of the characteristic V-I graph to represent impedance, see this answer.

To answer your question, it remains to be determined which of the boxes B, C and D has behaviour which most closely resembles the behaviour of box A.

We are permitted to attach any load \$R_L\$ we desire and you could even apply some arbitrary potential difference between X and Y, and it would still keep \$I_O\$ constant. The op-amp will adjust \$V_{XY}\$ to whatever value is necessary to keep \$I_O\$ fixed. Thus \$\Delta I_O = 0\$, so:

$$ R_{XY(A)} = \frac{\Delta V_{XY}}{0} = \infty \Omega $$

This is consistent with the behaviour of the ideal current source in box C.

It's true that the op-amp is indeed a voltage source, using voltages to obtain this behaviour, but in the context of a two-terminal "black box", for which we only have access to the exposed terminals, nodes X and Y, any external "observer" circuitry would perceive it to behave like a current source, for which the impedance inside the box is effectively infinite. No amount of voltage applied, and no impedance of load attached (op-amp constraints notwithstanding, such as limited output voltage range and current limits) will result in a change in \$I_O\$.

Answer 4

You are correct, the output impedance of an ideal op-amp is zero.

However, the output impedance of the whole op-amp circuit that drives the load resistance Rload is not zero.

You can think that the load resistance Rload can be any arbitrary value, and still the current only depends on the value of Rf and Vin.

You can use Kirchhoff's laws or golden rules of ideal op-amps to prove that voltage over Rf equals Vin and thus current is only defined by Rf.

So no matter how many teraohms or micro-ohms the Rload is, there will be up to teravolts or whatever is required at the op-amp output, and over Rl to make enough current flow that voltage over Rf equals Vin.

Answer 5

When they say "output impedance of the amplifier" they mean output impedance of the closed loop amplifier.

The circuit represented by the symbol below is an op amp, which you typically put feedback around to make various kinds of amplifiers. In other words, the op amp is an open loop amplifier and, after placing feedback around it, you create a closed loop amplifier.

^{simulate this circuit – Schematic created using CircuitLab}

Depending on the feedback network, you can make 4 types of closed loop amplifier: voltage, current, transconductance, and transimpedance. They will have either infinite or zero input impedance, and infinite or zero output impedance.

So, you are right, an ideal op amp does have zero output impedance, but depending on the kind of feedback network, you can create a closed loop amplifier with either zero or infinite output impedance.

Answer 6

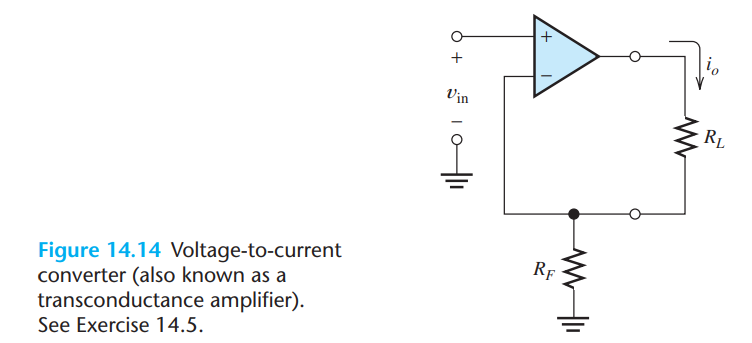
Short answer

The circuit presented by OP is a constant current source (op-amp and current-setting resistor RI) that supplies a "floating" load RL. Through negative feedback, the voltage across RI is maintained equal to the input voltage (Vin). As a result, the current flowing through both RI and RL depends only on Vin and RI, following the equation IL = Vin/RI.

---

Beyond IL = Vin/RI

Such "textbook explanations" using established terms that have become verbal clichés do a good job in many cases but not when the goal is to understand the circuit solution. Understanding is different from knowing and doing. It requires you to understand the idea, the concept, the principle... Apparently, that is why the authors of the famous book from which the OP's question was born showed this necessity in the book title, but for some reason they left it to us to do it. Then let's take advantage of this unique opportunity and discover the principles on which this class of circuits is built. This will give the OP a good foundation in their future encounters with similar circuit solutions.

Classic negative feedback solution (OP's circuit)

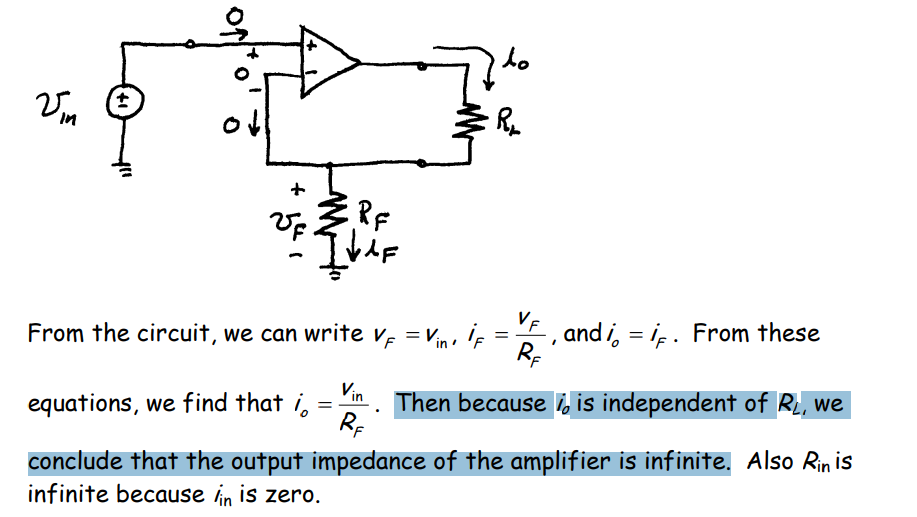
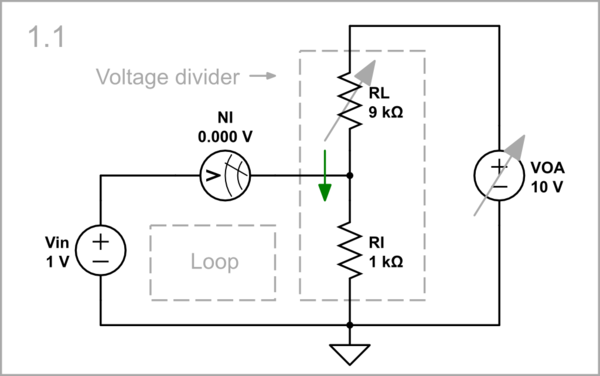
Basic idea: To set a current of a certain value through the load according to the principle of negative feedback, we need to convert it to a voltage (because our devices work with voltage) and compare it with the input voltage. Then we vary the current until the difference between the two voltages becomes zero. As a result, IL = VRI/RI = Vin/RI.

Implementation (conceptual circuit): For this purpose, we (the "op-amp" ) apply a variable voltage VOA to the RL-RI network. Then we subtract in series manner the voltage VRI from Vin, and properly apply the result to the op-amp differential input. Finally, we vary the voltage until reach equilibrium (VNI = 0 V).

^{simulate this circuit – Schematic created using CircuitLab}

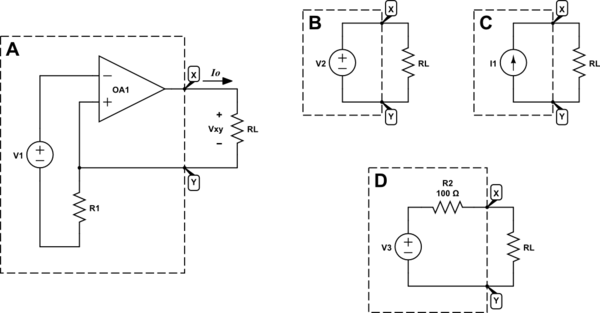
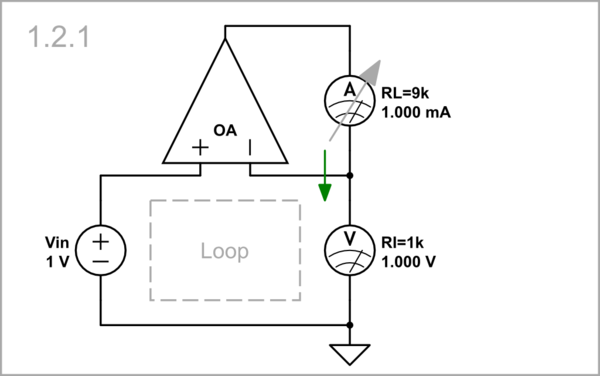
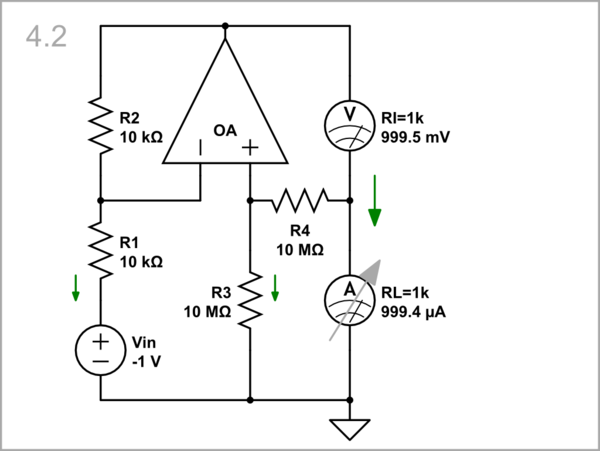
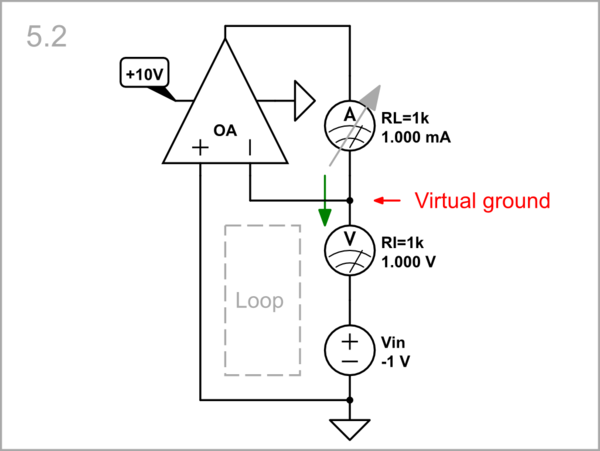
Op-amp non-inverting current source (OP's circuit): We can make an op-amp do this routine work. For simplicity, I have replaced the resistors with meters of the same internal resistance.

^{simulate this circuit}

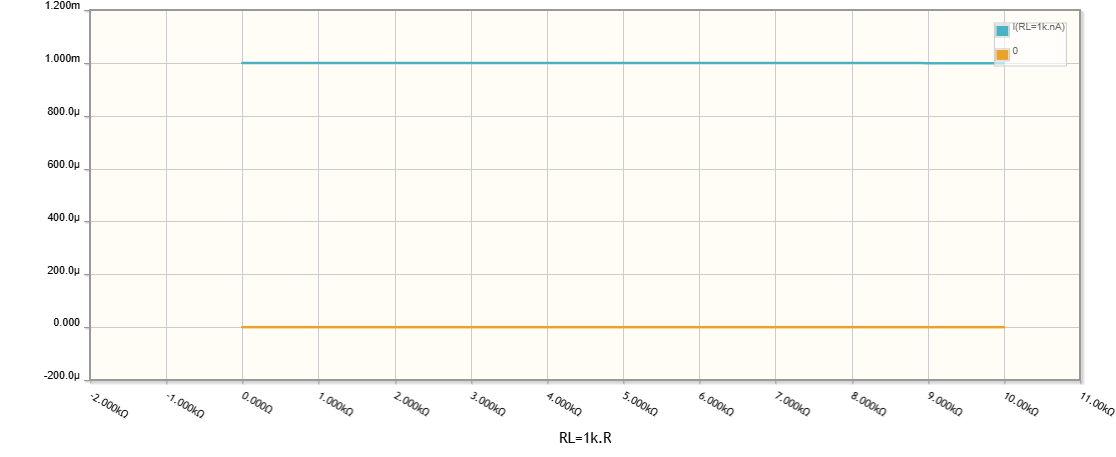
Let's investigate the current source at various loads (from 0 to 10 kΩ) by the help of the CircuitlLab DC Sweep Simulation. As you can see, the curve of the current (in blue) is absolutely horizontal.

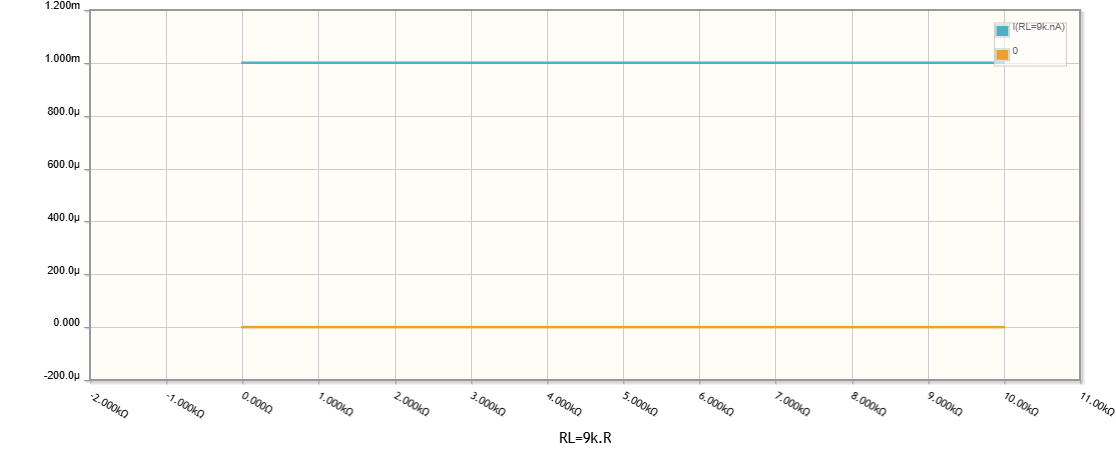
Transistor current source with emitter degeneration: This circuit solution has long been known in its transistor implementation. To make the circuit more perfect, I have added a bias voltage.

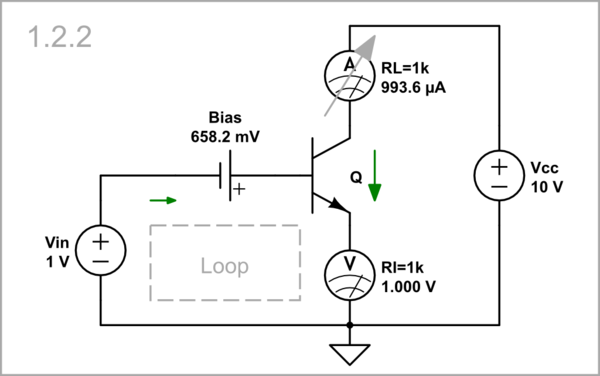
^{simulate this circuit}

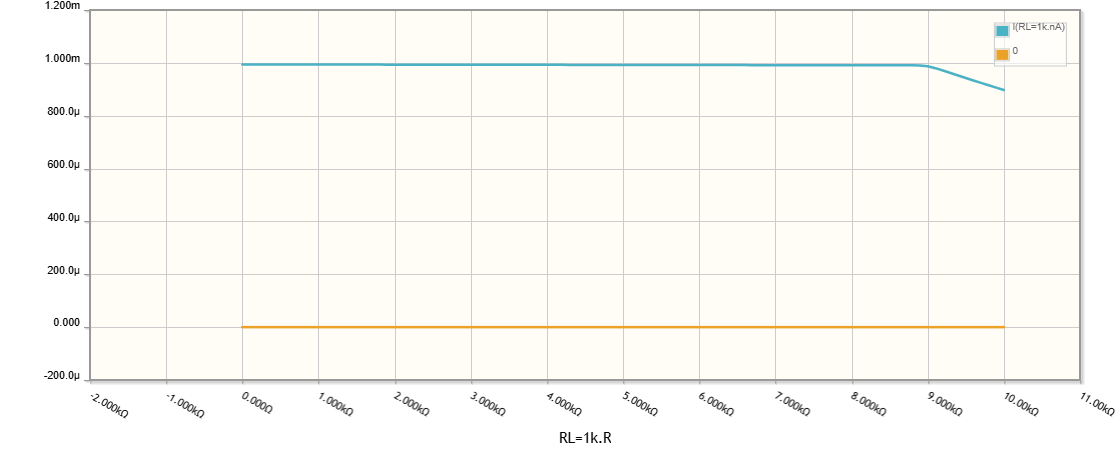
Problem: In the configurations above, both input voltage source and current-setting resistor are grounded but the load is "floating" which is in most cases inconvenient.

Negative feedback solution with floating input voltage source

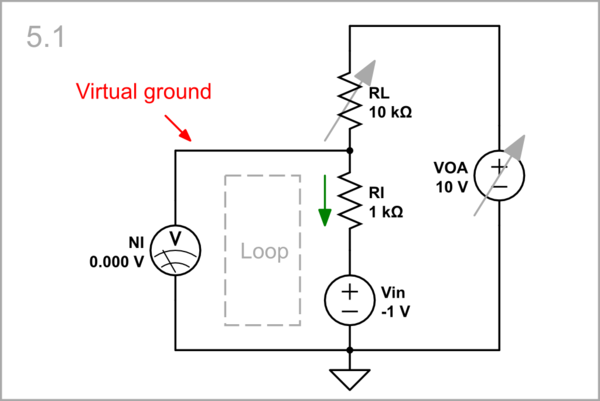
Conceptual circuit: We can swap the resistors so that the load is grounded but now the input voltage source is "floating". As you can see, Vin, RL and the null indicator NI are connected in a loop.

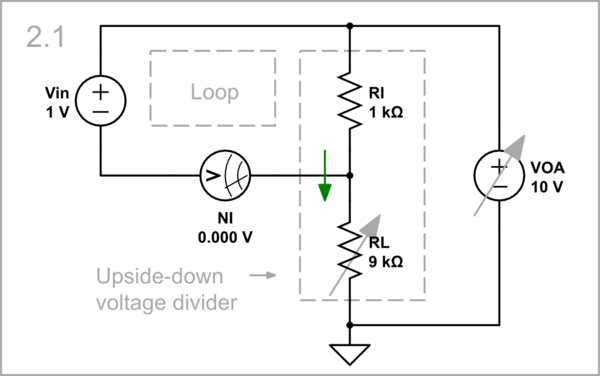
^{simulate this circuit}

Op-amp circuit: Vin, RL and the op-amp differential input are connected in a loop.

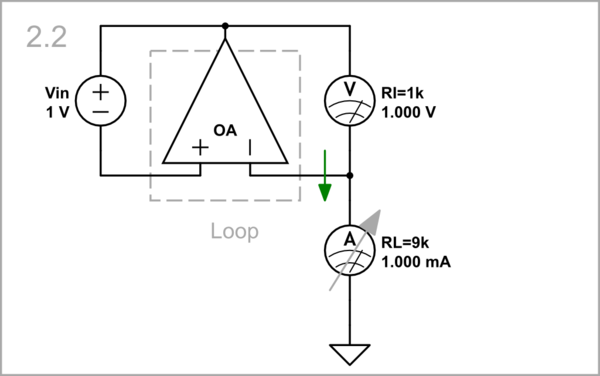
^{simulate this circuit}

Both negative and positive feedback solution (NIC)

Conceptual circuit: The input source may be grounded if it is a current source.

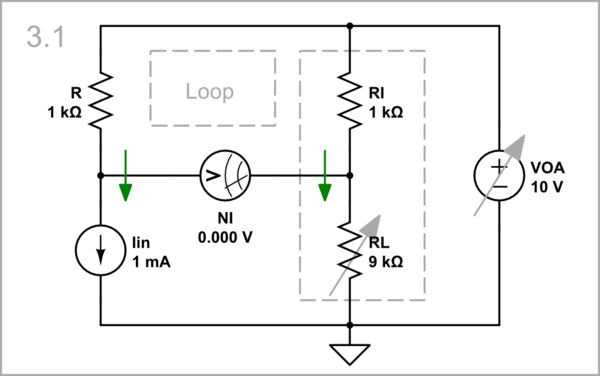
^{simulate this circuit}

Op-amp current mirror: This is the odd negative impedance converter (NIC). The op-amp simultaneously changes the voltages at its inputs to reach equilibrium (VRI = VR).

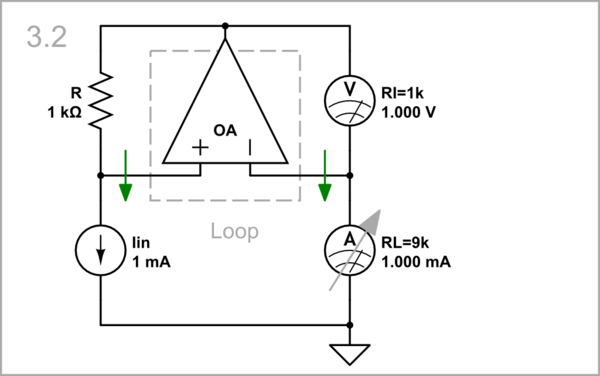
^{simulate this circuit}

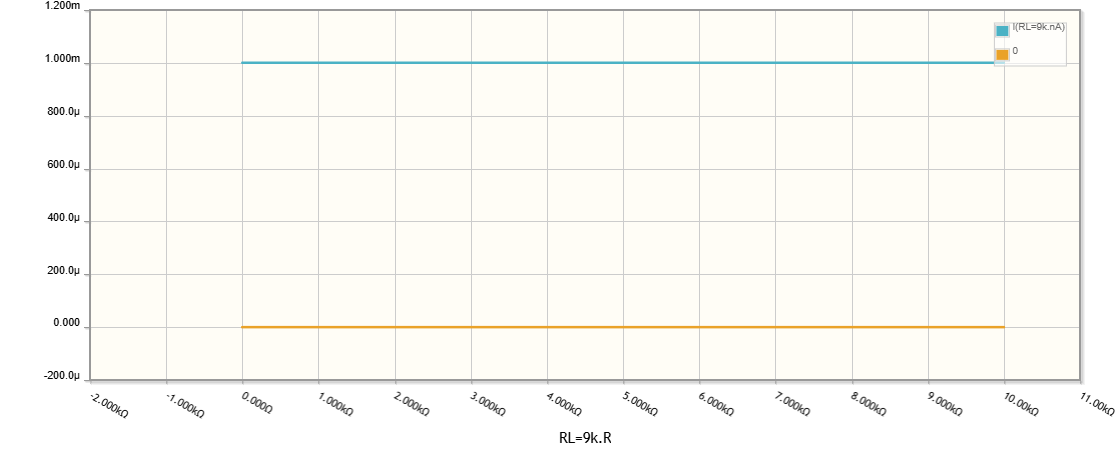
No negative feedback solution (bootstrapped RI)

Conceptual circuit: Constant current can also be obtained without using the principle of negative feedback. If we are observant enough, we will notice that the voltage at the upper end of the current-setting resistor RI follows the voltage at its lower end. So we can add a constant offset voltage to the load voltage and apply it to the upper end of RI.

^{simulate this circuit}

As you can see in the graphs beluw, the curve of the upper end is lifted by 1 V above the curve of the bottom end.

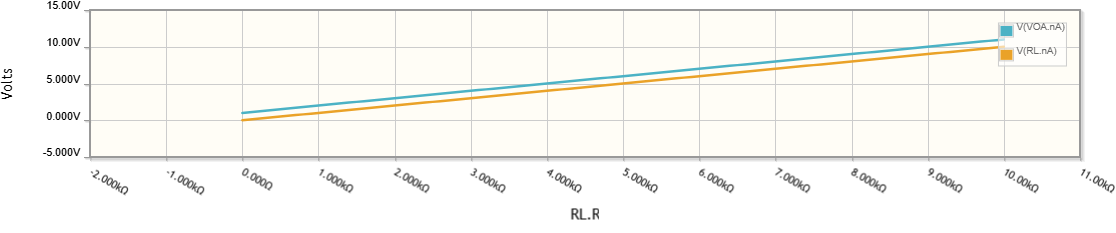
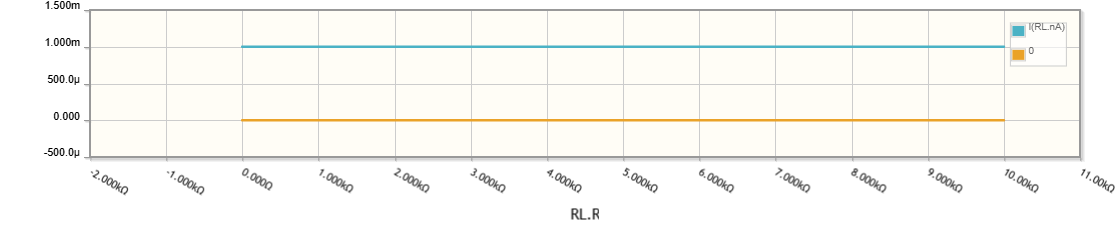
Improved Howland current source: This idea is implemented in the so-called "Improved Howland current source". The load voltage variations are amplified 1x and appear at the top RI end.

^{simulate this circuit}

Inverting current source

Conceptual circuit: Finally, let's see how the same idea is implemented through an inverting op-amp configuration.

^{simulate this circuit}

Op-amp inverting current source: Here, the voltage across the current setting resistor RI is subtracted from the input voltage and the result is zero (the so-called virtual ground). The load is virtually grounded which is better than to be floating. I have used an op-amp with supply ends to show the load limitations.

^{simulate this circuit}

When RL exceeds 10 kΩ, the op-amp output voltage reaches the supply rail and stops changing.

What "infinite output impedance" means

The main property of a constant current source is that its current does not change when we change the resistance of the load (the voltage across it). But if we don't want them to understand us :-), we say that "its output impedance is infinite". What is actually going on in the OP's circuit that this is the case?

Regardless of the specific implementation, the same thing happens: When we start increasing the load resistance RL, the op-amp current source starts increasing the load voltage VRL by the same amount so the ratio VRL/RL = IL does not change. So the main idea in these constant-current circuits is dynamic voltage.

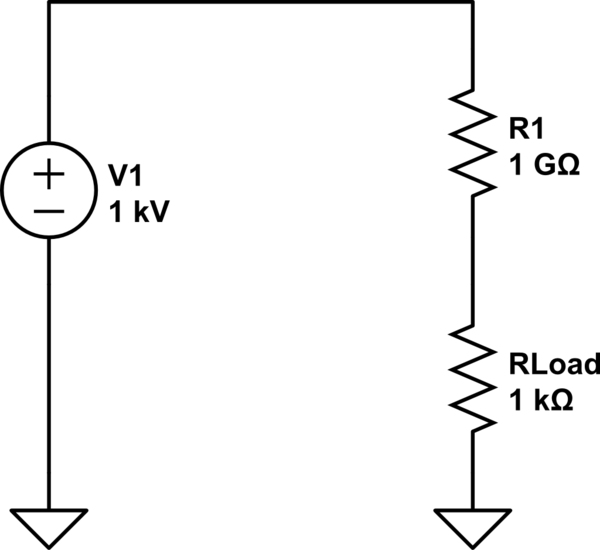
The same effect can be achieved with infinite "static" (constant) voltage and infinite constant resistance but the losses will be great.

So the end result for both implementations is the same - infinite resistance, but the nature of that resistance is different:

• In the first case it is not physical resistance but dynamic voltage that creates the same voltage opposition as the physical resistance (aka "bootstrapping").

• In the second case it is physical resistance.

Answer 7

The output impedance of a two port network can be found by replacing the load resistor by a current source with known current \$I_\text{TEST} \$, calculating the voltage drop across it, and dividing the two. In this case it would look like this: -

^{simulate this circuit – Schematic created using CircuitLab}

No current flows into the opamp terminals so \$V_\text{TEST-} = -I_\text{TEST}R_f \$. The output of the opamp is equal to the open loop gain \$A_0\$ multiplied by the voltage difference between the terminals, so $$V_\text{TEST,+} = A_0(V_\text{in}-V_\text{TEST-}) $$ Assuming that \$V_\text{in} \neq V_\text{TEST-}\$ we can without loss of generality set \$V_\text{in} = 0 \: \text{V} \$, causing

$$V_\text{TEST,+} = -A_0V_\text{TEST-} = A_0I_\text{TEST}R_f $$

The voltage drop across \$I_\text{TEST}\$ is

$$V_\text{TEST,+} - V_\text{TEST,-} = A_0I_\text{TEST}R_f - I_\text{TEST}R_f = I_\text{TEST}R_f(A_0-1)$$

Finally , the output impedance

$$Z_\text{out} = \frac{V_\text{TEST,+}-V_\text{TEST,-}}{I_\text{TEST}} = \frac{I_\text{TEST}R_f(A_0-1)}{I_\text{TEST}} $$

$$Z_\text{out} = {R_f(A_0-1)} $$ For an ideal opamp \$A_0 \to \infty\$ so clearly $$Z_\text{out} \to \infty $$

Two things to note here: First, is that the circuit is simply a non-inverting amplifier. The interesting part, is that the feedback resistor is considered as the load resistor \$R_L\$ which I haven't seen before. Secondly, in the analysis above note that the principle of a virtual short doesn't apply to the opamp's input terminals because the ideal current source determines the voltage across \$R_f\$ independently of everything else. In a general setting with a regular load resistance the virtual short principle will apply, however.