Sallen and Key
R. P. Sallen and E. L. Key's 1955 IEEE (IRE) paper, "A Practical Method of Designing RC Active Filters," is worth reading. But even better is their (now) declassified report, R. P. Sallen and E. L. Key, "A Practical Method of Designing RC Active Filters," MIT Lincoln Laboratory Technical Report, No. 50, May 6, 1954 (aka "TR 50".) Either one of these is worth reading, but TR 50 is in my opinion far better. Unfortunately, the US Air Force still has to clear you getting a copy of it and you have to write a justification to do so before MIT will release a copy. (Sadly.)
I have both and I consider them very well worth the time to read. They don't really bother with 1st order filters (I think they figure that's "too easy" to cover.) But they do cover 2nd order and higher, which is very nice. (Appendix I of TR 50, for example, covers both Butterworth and Tschebyscheff.)
The modern interpretation of Sallen-Key filters is usually a 2nd order filter using two resistors (in equal-valued pairs) and two capacitors (also in equal-valued pairs.) They do happen to cover the idea of same-valued components in their paper, but I don't consider it to be the main thrust. So I think it's just popularizers of their ideas, such as Don Lancaster, that has deepened this connection more than the original authors did, themselves. (Sallen and Key were after more theoretical "fish," so to speak.)
If you want, you can refer to a couple of things I wrote here and here. May help. May not.
Sallen and Key HP Filter
For the first stage, the simplest forms of high-pass Sallen-Key filters are:
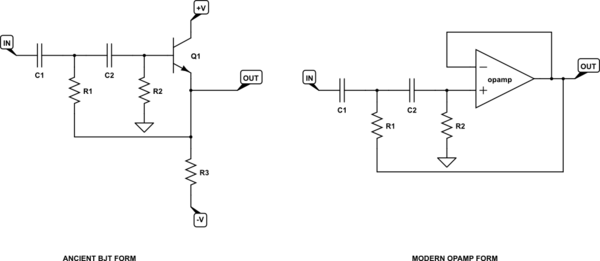
simulate this circuit – Schematic created using CircuitLab
(I'm including the ancient version just so you can think about it.)
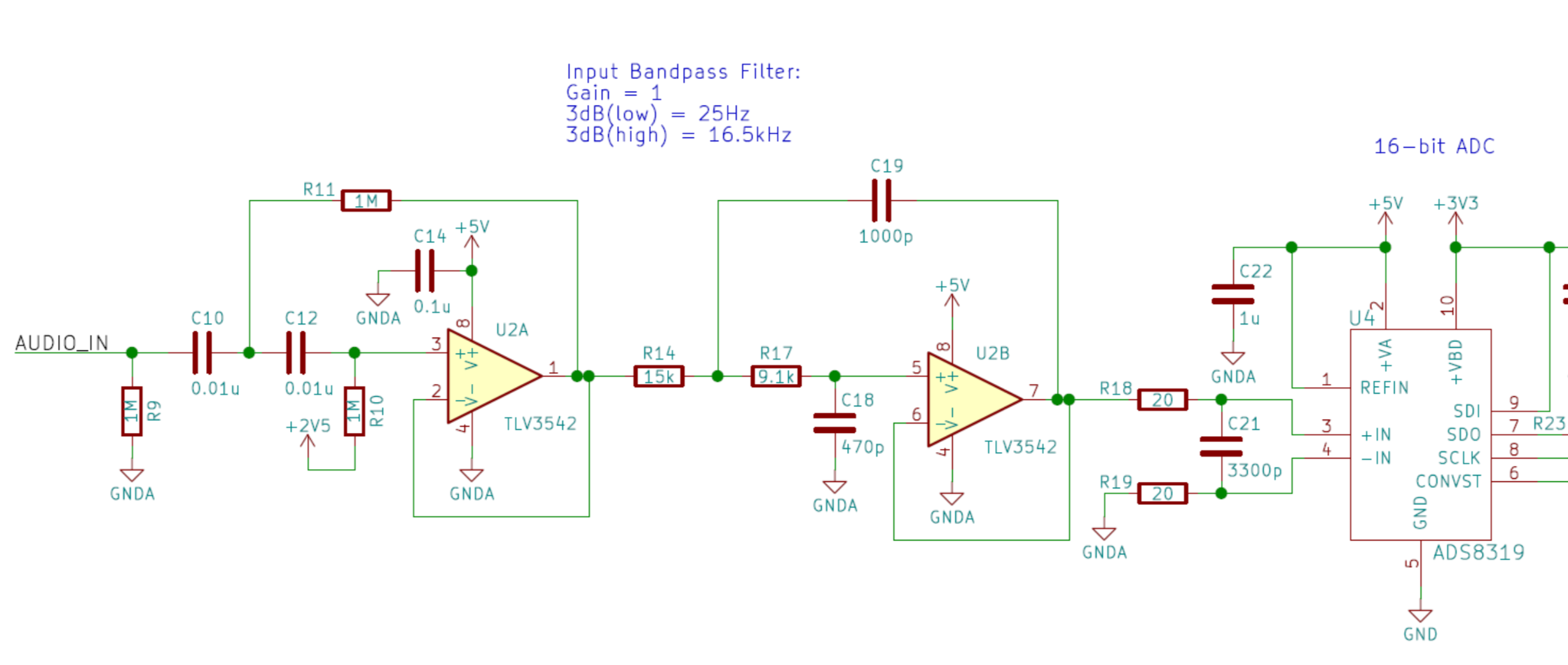
Your first stage looks a lot like the right-hand side (modern) case. If you want, you can read though this EESE link to see how you interpret the above schematic. But when both resistors and both capacitors have the same value and the voltage gain \$K=1\$, then \$\omega_{_0}=\frac1{R\,C}\$ and:
$$\begin{align*}\frac{e_{_\text{out}}}{e_{_\text{in}}} &=\frac{K\cdot \omega_{_0}^2}{s^2+\left(3-K\right)\omega_{_0}\,s +\omega_{_0}^2}=\frac{\omega_{_0}^2}{s^2+2\zeta\,\omega_{_0}\,s +\omega_{_0}^2}\end{align*}$$
You should easily see that with \$K=1\$ then damping factor \$\zeta=1\$, which is a critically damped Butterworth filter. With the values you have in hand for that first stage, you can compute \$\omega_{_0}=\frac1{R=1\:\text{M}\Omega\:\cdot\: C=10\:\text{nF}}=100\$ or that \$f\approx 15.92\:\text{Hz}\$. (The \$2.5\:\text{V}\$ reference that feeds one end of \$R_{10}\$ simply establishes a DC biasing for the opamp input node so that its output will be similarly biased half-way between the rails.)
\$R_9\$ provides a DC path for the input node (good idea) and depending on the source resistance might adjust the exact roll off point. Not likely and I don't think it changes as much as the diagram suggests. So I'm just calling it good enough, for now. Maybe it is my own ignorance.
Sallen and Key LP Filter
For the second stage (low pass), the simplest forms of low-pass Sallen-Key filters are:
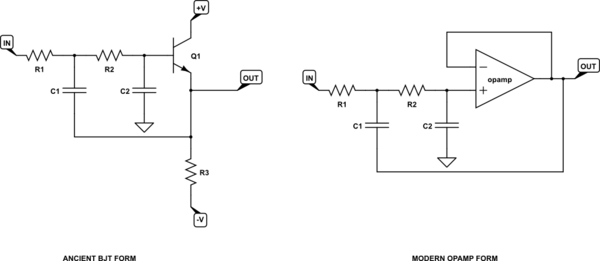
simulate this circuit
Here again the gain voltage gain is \$K=1\$, but now the resistor and capacitor values are not the same, so \$\omega_{_0}=\frac{1}{\sqrt{R_1\:C_1\:R_2\:C_2}}\$ (I'm just using subscripts 1 and 2 for convenience -- obviously, these don't match the schematic's numbering) and \$\zeta=\sqrt{2}\$. So I get \$f\approx 19.87\:\text{kHz}\$. But since \$\zeta=\sqrt{2}\$, it is over-damped (probably to provide a maximally flat time delay) so the roll-off may occur a little earlier than what \$\omega_{_0}\$ suggests. But again, I didn't go further to verify the given value.
That's as much as I want to add here and I certainly may have made mistakes. But it should provide an approximate overview that helps, at least, identify some of the components you weren't so certain about.