1
\$\begingroup\$

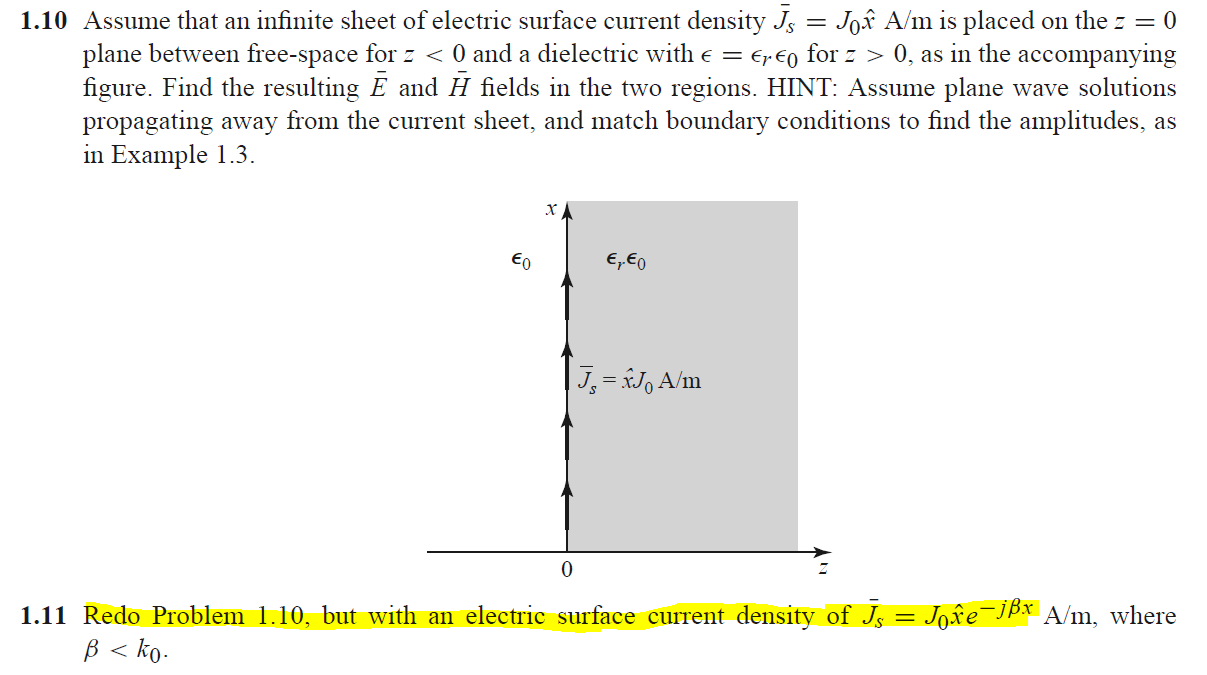
I am asking this question because, even though I have the solutions manual from the exercises of the book "Microwave Engineering 4th edition", by David M. Pozar (one of the Bible books for microwave circuits and systems), I just don't understand the solution.

The exercise 1.11 from this book states the following:

"Assume an infinite sheet of electric surface current density \$ \vec{Js}=Jo·exp(j\beta z)·\vec{x} \$ is placed on the z=0 plane between free-space for z>0 and a dielectric with \$\epsilon = \epsilon_0·\epsilon_r\$. Find the resulting E and H fields in the two regions"

enter image description here

I was thinking of solving it by using the boundary conditions between two dielectric media. In fact, the previous exercise (1.10) is similar, but without the exponential term in the expression of the current sheet:

\$\nabla\$ x \$(\vec{E_2} - \vec{E_1}) = 0 \$

\$\nabla\$ x \$(\vec{H_2} - \vec{H_1}) = \vec{J_s} \$

But the solutions manual starts the exercise by assuming that this current sheet will generate obliquely propagating plane waves, without any further information, thus employing expressions for E and H fields which have components in the z direction. I can't understand the reasons why it makes this assumption. I hope you can help me.

\$\endgroup\$
5
  • \$\begingroup\$ The solution assumes a propagating plane wave where both H and E have z components? That cant be right? \$\endgroup\$
    – MAM
    Commented Sep 26, 2019 at 21:35
  • \$\begingroup\$ Hmm given that the plane is the XZ plane, it is still a plane wave! \$\endgroup\$
    – Terahertz
    Commented Sep 27, 2019 at 14:50
  • \$\begingroup\$ What i mean to ask is, E and H must be orthogonal so cant both have Z components. Do you mean E and H both vary with z or they both have a non zero z axis component? \$\endgroup\$
    – MAM
    Commented Sep 27, 2019 at 15:14
  • \$\begingroup\$ sorry, I didn't understood properly. In the solution given by the manual, it states that the E field has both x and z components, while H must, therefore, have only a y component \$\endgroup\$
    – Terahertz
    Commented Sep 27, 2019 at 16:05
  • \$\begingroup\$ Now that makes sense. But in your question you say "thus employing expressions for E and H fields which have components in the z direction" \$\endgroup\$
    – MAM
    Commented Sep 27, 2019 at 16:22

1 Answer 1

Reset to default
1
\$\begingroup\$

With a discontinuity the tangential fields are equal, the normal fields are not.

The normal fields are not only if there is a surface charge, if there is a surface charge then use the equations below:

enter image description here

enter image description here
Source: http://www.antenna-theory.com/tutorial/electromagnetics/electric-field-boundary-conditions.php

The only difference is now you are given a surface charge density so to find the magnetic field component, you must take the gradient of the surface charge which will vary by the equation given.

The difference is you are given a surface charge density

\$\endgroup\$
1
  • \$\begingroup\$ The only relationship between the suface current density and the change that I know is the Law of conservation and it establishes a relation between the gradient of the current and the time derivative of the charge, which is not straight forward in this case. What am I missing then? \$\endgroup\$
    – Terahertz
    Commented Sep 26, 2019 at 16:14

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.