Calculating uncertainty in a current divisor

Question

I had the following exercise to do for college:

I designed an analog ammeter exactly like this:

^{simulate this circuit – Schematic created using CircuitLab}

The idea is to design an ammeter capable to measure up to 1 mA, with the D'Arsonval galvanometer of 50 μA. So we put a shunt resistor of 26.32 ohms to redirect the excess current.

The problem is that the shunt had an uncertainty of 3%, everything else is exact. So to calcule the uncertainty of the ammeter I do the following calculations with the current divisor:

$$I_{m}max = I * \frac{R_s max}{R_s max + R_m} $$ $$I_{m}min = I * \frac{R_s min}{R_s min + R_m} $$ $$\Delta I = \frac{I_{m}max - I_{m}min}{2} $$ Defining epsilon as the uncertainty: $$\varepsilon = \frac{\Delta I_{m}}{I_{m}} $$ And Rmax and Rmin are: $$R_{s}max = R_s + 3\%R_S$$ $$R_{s}min = R_s - 3\%R_S$$ Ok lets do the math: $$I_{max} = 1mA * \frac{26.32 * 1.03}{26.32 * 1.03 + 500} = 0.05143\,mA$$ $$I_{min} = 1mA * \frac{26.32 * 0.97}{26.32 * 0.97 + 500} = 0.04858\,mA$$ $$\Delta I = \frac{0.05143 - 0.04858}{2} = 0.001464\,mA $$ So the result is: $$\varepsilon = \frac{0.001464\,mA}{0.05\,mA} = 0.029 \simeq 2.9\% $$

The problem I found doing this is when using other method: uncertainty propagation, the result is considerable different when we talk about uncertainty, look:

Considerer the uncertainty of a multiplication is the sums of each uncertainty, and to get the uncertainty of a summ first u have to calculate the absolute uncertainty of each.

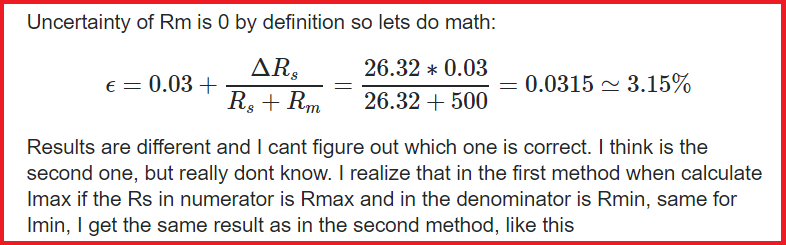
$$\epsilon = \epsilon (I) + \epsilon (\frac{R_s}{R_s + R_m}) $$ Uncertainty of current is 0 by definition. $$\epsilon (\frac{R_s}{R_s + R_m}) = \epsilon (R_s) + \frac{\Delta(R_s + R_m)}{R_s + R_m} = 0.03 + \frac{\Delta R_s + \Delta R_m}{R_s + R_m} $$ Uncertainty of Rm is 0 by definition so lets do math: $$\epsilon = 0.03 + \frac{\Delta R_s}{R_s + R_m} = 0.03 + \frac{26.32 * 0.03}{26.32 + 500} = 0.0315 \simeq 3.15\%$$

Results are different and I cant figure out which one is correct. I think is the second one, but really dont know. I realize that in the first method when calculate Imax if the Rs in numerator is Rmax and in the denominator is Rmin, same for Imin, I get the same result as in the second method, like this

$$I_{m}max = I * \frac{R_{s}max}{R_smin + R_m}$$ $$I_{m}min = I * \frac{R_{s}min}{R_smax + R_m}$$

But I dont think that is correct to do, cause I am treating each Rs as differents variables.

Thanks everybody!

Answer 1

Sanity checks: -

• If Rs increases by 3% then the current through the meter rises from 50 uA to 51.43 uA when 1 mA feeds the circuit
• That's an error of 2.86%

OK I agree with part 1 of your answer. But part 2 seems all mixed up. Extract from question : -

• You didn't alter Rs in the denominator to account for it having an uncertain value.
• Putting the numbers into the formula (corrected or not) does not give 3.15% by a long way

Answer 2

The first method is fine but there is a mistake in the second method. You are right until the first error propagation step.
$$\epsilon = \epsilon(I) + \epsilon(\frac{R_s}{R_s+R_m})$$ You need to do the error propagation for the quotient as well: $$\epsilon = 0 + \epsilon(R_s) - \epsilon(R_s+R_m)$$ $$\epsilon = \frac{\Delta R_s}{R_s} - \frac{\Delta (R_s+R_m)}{R_s+R_m}$$ $$\epsilon = \frac{R_m}{R_s+R_m}.\frac{\Delta R_s}{R_s} = 0.0285 = 2.85\%$$ Same as what you get from the first method as it should be.