How do analyze input impedance of Class AB amplifier biased with resistors?

Question

EDIT: I realize that the original circuit is deeply flawed and will burn up upon re-entry. Thanks to anyone who can help me understand why!

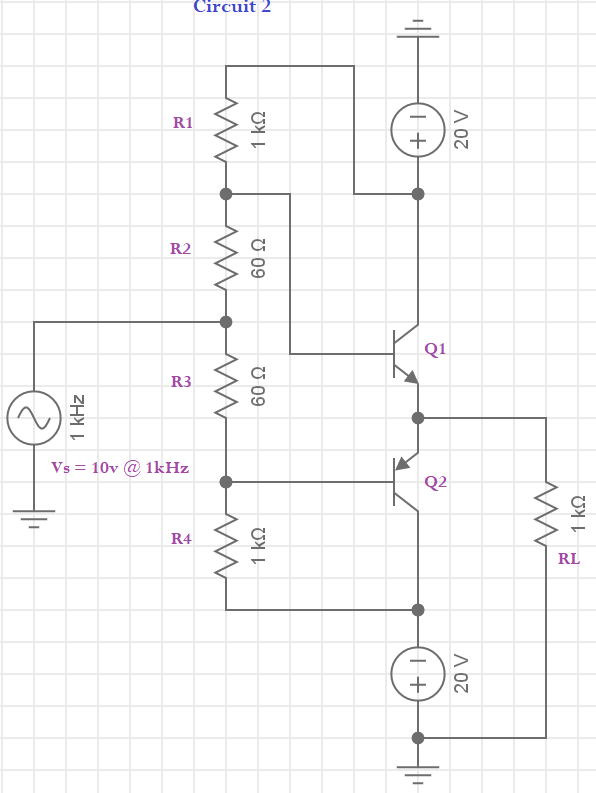
I'm learning to analyze Class AB amplifiers, but haven't found a good source with information on how to determine their input impedance. I built this circuit as an example. It's a simple Class AB amplifier with resistors to overcome crossover distortion. I would love feedback on whether my understanding of \$R_{in}\$ is correct. I assumed both transistors have a \$\beta=50\$.

As far as I can tell, \$V_S\$ will see two branches: first, it will see the \$60\Omega\$ R2 in series with the parallel combination of R1 and the impedance looking into the base of Q1; in the other branch, it sees \$60\Omega\$ from R3 in series with the parallel combination of R4 and the base of Q2. So an equation like the following should work, yes?

$$R_{in}=(R_2+(R_1||R_{ibQ1}))||(R_3+R_4 || (R_{ibQ2}))$$

I started with the Q1 branch. First I found \$r_e\$. \$I_C\$ fluctuates between 2.6mA and 2.7mA, giving a very small value for \$r_e=25mv/2.6mA=9.62\Omega\$. With this in mind I can solve for \$R_{ibQ1}=\beta*(r_e+R_L)=50*(1000+9.62)=50,481\Omega\$. \$R_{ibQ1}\$ is in parallel with R1 and that combination is in series with R2, so the entire Q1 branch of the circuit is $$R_{Q1branch}=60\Omega+(1000\Omega||50481\Omega)=1040\Omega$$

From here I'd like to think it's as easy as imagining the Q2 half of the circuit as equivalent in impedance. The total input impedance of the circuit, then, is half of the previous calculation, or \$R_{in}=1040||1040\Omega = 520\Omega\$

I'm sure this isn't exactly correct, as it seems way too simple to only solve half the circuit. I'd love to hear all the ways I've been led astray. Please note that this is not an amplifier I imagine has any real purpose, just one that works enough to help me understand how to analyze its input impedance.

Answer 1

At midpoint, your transistors will be conducting AMPS and AMPS, because of the 1.2 volts across each base. And depending accuracy of the transistor model and if a power_transistor model, the current could be thousands or millions of amps, which melts the copper wires in your circuit.

These AMPS will flow thru BOTH transistors, dissipating hundreds of watts.

Or even millions of watts.

And the BETA likely will be very low, because BETA does collapse at high current densities.

I doubt that is what you intended.

============================================

Impose a zero volt input. We'd like approx. zero volts output, and some moderate milliamp emitter current.

For easy back_of_envelop analysis, remove the bottom half of the circuit. Now we have two resistors and a NPN. Ground the emitter of the NPN. What are the currents?

We'd like the 2 resistors (1Kohm and 60 ohms) to be a well-behaved voltage divider. So temporarily remove the NPN.

The 1Kohm dominates, thus the current (viewing that 1 Kohm resistor as a nice 1mA/volt beast) from the 20 volts (ignore the 60 ohms) is 20 milliAmps, closely. Not exactly . But this is back_of_envelope.

Now reinstall the 60 ohms. 1mA produces 60mV. 10mA produces 600mV (about what we need). And 20mA produces 1,200 milliVolts. Which is what we get. So?

If we assume 0.6 volts for 1milliAmp for many bipolars (approximately), then 1.2 volts will produce

• scale_factor = 10^ (1.2 - 0.6)/0.058

• scale_factor = 10 ^10

and the collector current (very close to the emitter value) will be

• 1mA * 10^1 = 10^7 amps or 10,000,000 amps

To avoid that, let us eat up that extra 0.6 volts, by inserting a 3 ohm resistor between each emitter and the Vout. The approximate current will be 0.6v / 3 = 0.2 amps, much more controlled than that 10,000,000 amps.

And if your simulator has a Power Bipolar Transistor model, then you will see about 0.2 amps (maybe 0.05 or 0.5) but not 10,000,000 anps.

Inserting small value resistors in emitter-to-output-node is a standard method for class AB amplifiers.

====================================

to nuggethead

the "scalefactor" in this case is just the name I attached to the RATIO of current at 0.6 volts across the emitter_base to the current at `1.2 volts across the emitter_base.