0
\$\begingroup\$

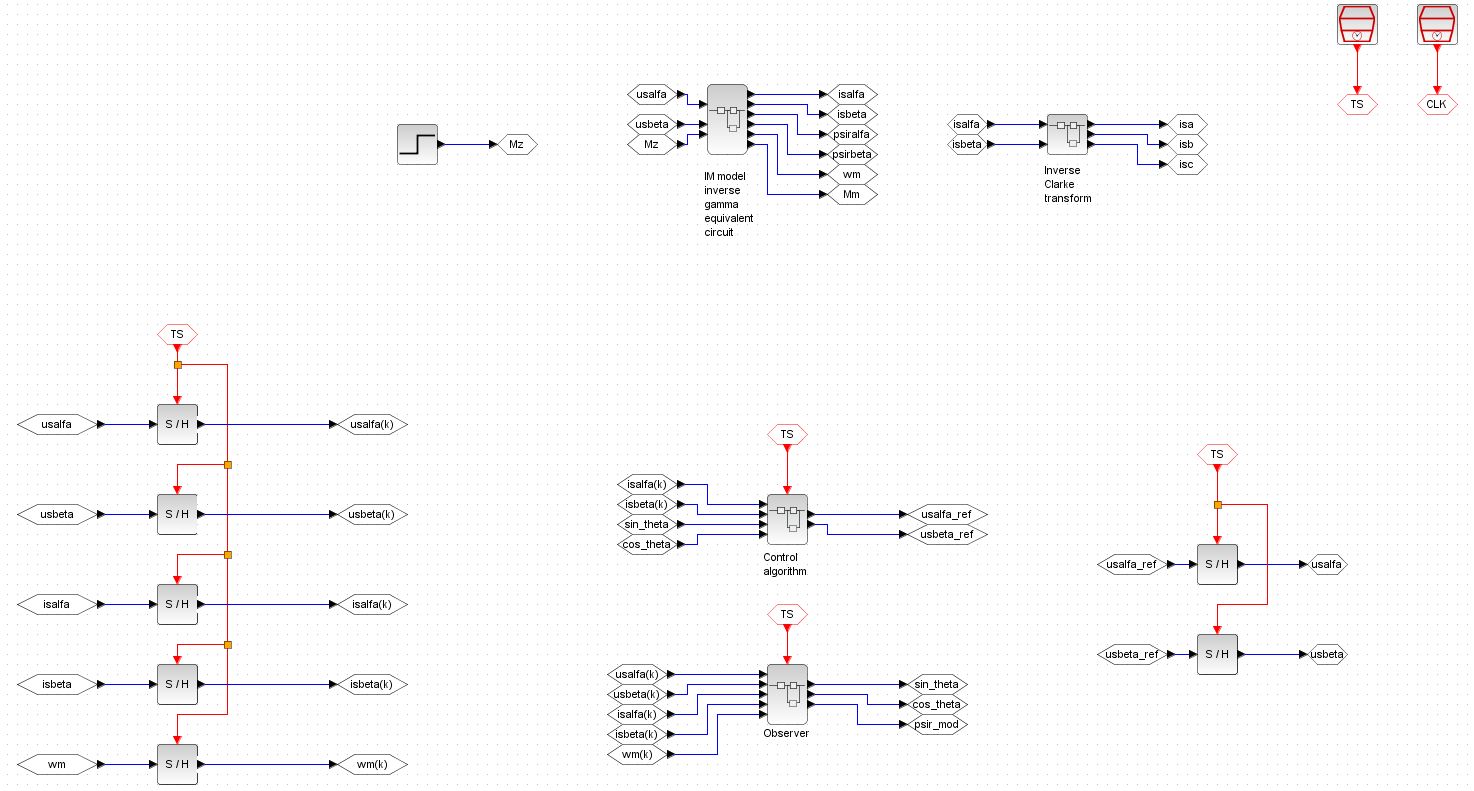
I have been developing a simulation of the field oriented control of the three phase induction motor. The simulation is developed in the Scilab/Xcos (v5.5.2) which is a free of charge Matlab/Simulink like software. The diagram of my simulation in the Xcos toolbox looks like this

enter image description here

As you can see the simulation basically consists of below mentioned main blocks:

  • IM model

    Dynamic model of the three phase induction motor

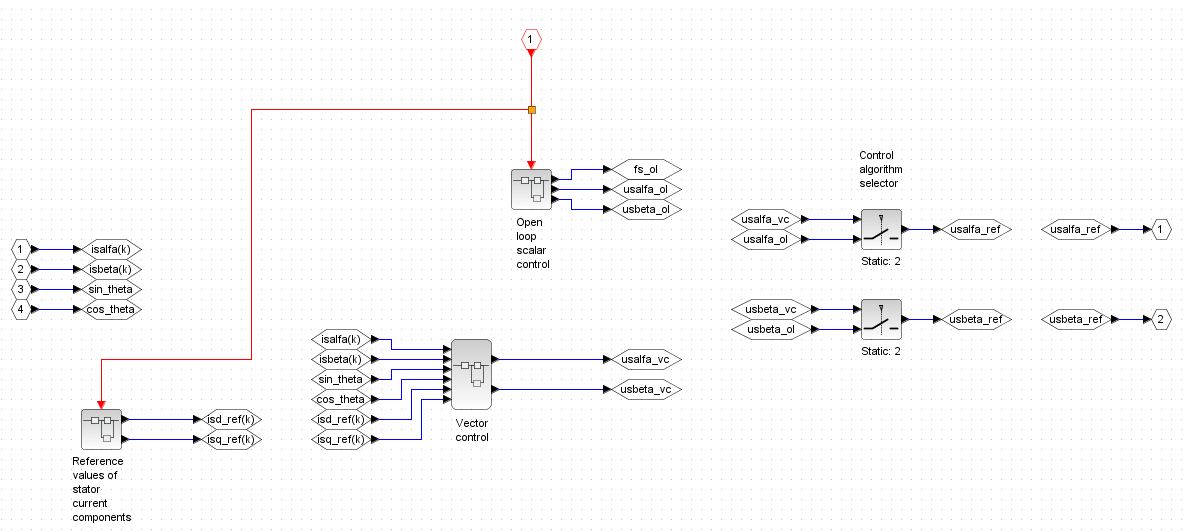
  • Control algorithm

    Produces components of the reference stator voltage in the stationary reference frame based on chosen control algorithm (open loop scalar control x field oriented control)

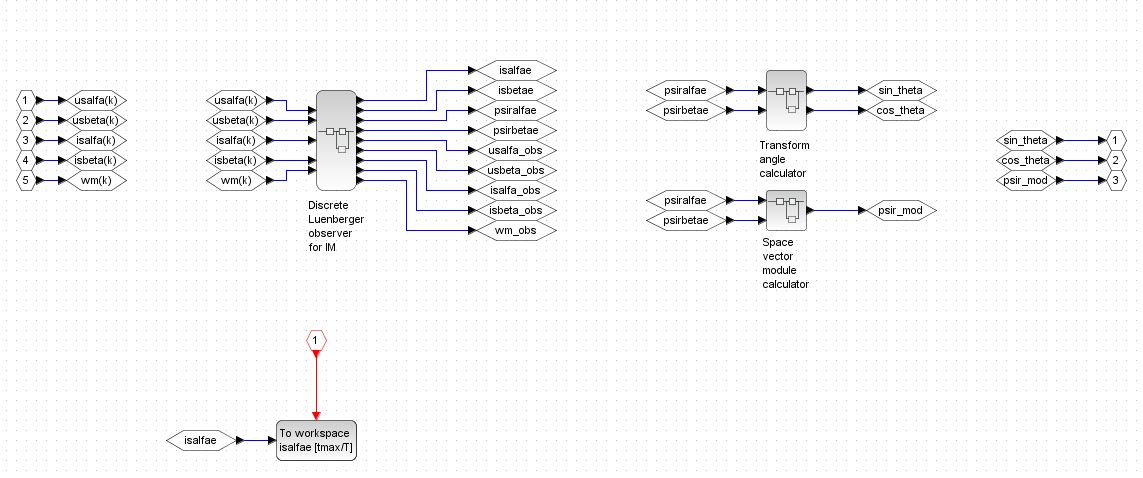
    enter image description here

  • Observer

    Calculates estimate of the components of the unmeasurable rotor flux and based on that calculates orientation of the space vector of the rotor flux for the field oriented control algorithm

    enter image description here

The block a) is a continuous time domain system and the blocks b) and c) are discrete time domain systems (they model software). Due to that arrangement the blocks b) and c) are separeted from the continous world (plant domain) via the ADC converter (modeled via sample and hold blocks) at their inputs and via the DAC conveter (first order hold algorithm) at their outputs.

The simulation has been developed in two steps:

  • induction motor controlled via open loop scalar control algorithm

    Simulation works correctly because no feedback is present

  • induction motor controlled via field oriented control algorithm

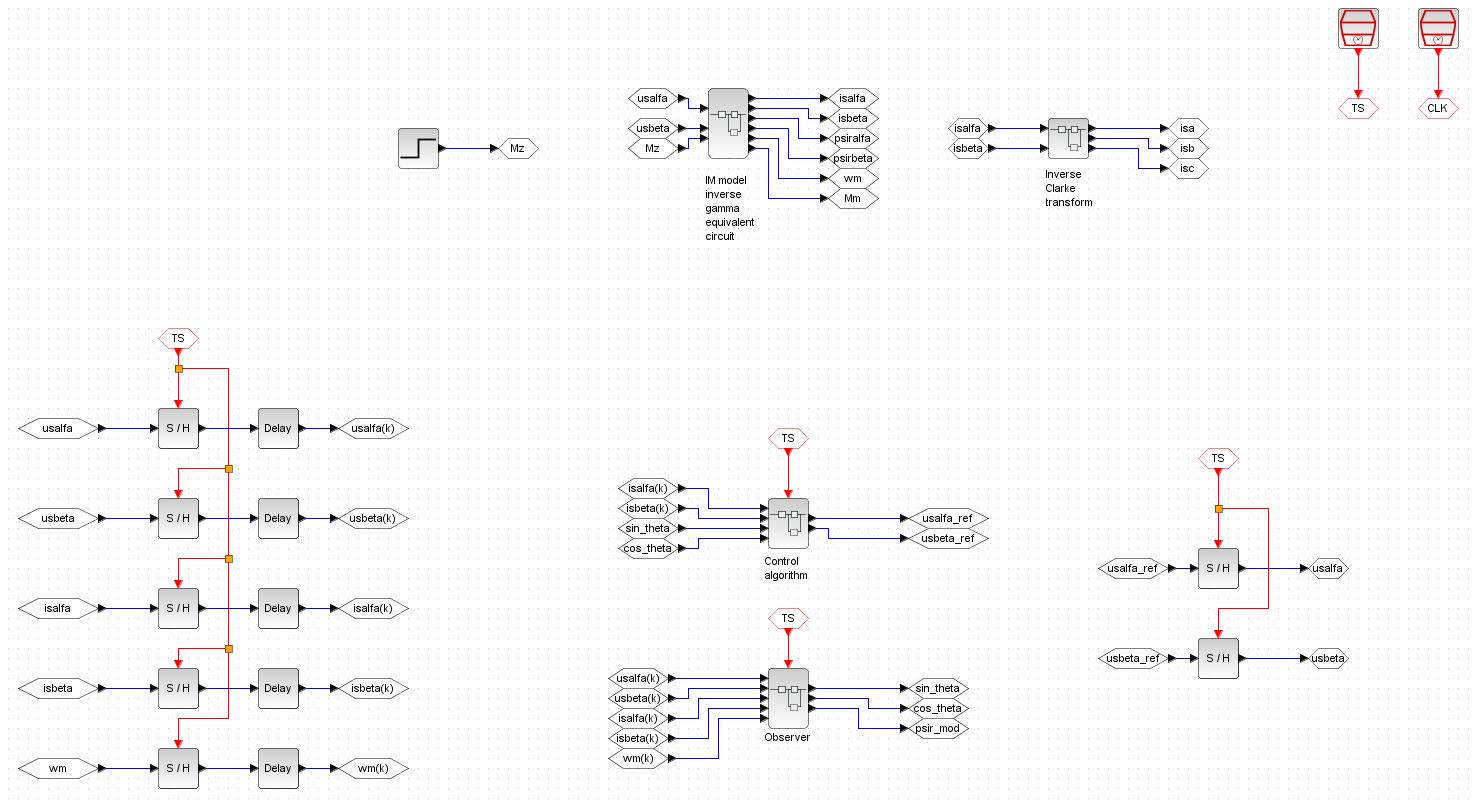
    At first I have encountered a problem with the algebraic loop error

    enter image description here

    Based on my experience with the Matlab/Simulink I have inserted a time delay into the control loop (please see the blocks "Delay" in the ADCs part)

    enter image description here

    I have set the delay of these blocks to a small fraction of the sampling period (\$T_s = 0.0001\,\mathrm{s}\$ and the delay has been set to \$0.1\cdot T_s\$). Insertion of that delay has resolved the problem with the algebraic loop but it has also caused two unwelcome facts. Firstly the state estimation error in the observer is high despite the "small" value of the delay. Secondly the delay insertion caused huge increase of the simulation time.

    I have also thought about simulation diagram rearangement to avoid the algebraic loop inherently but I can't see any way how to do that - ultimately I simulate the feedback loop.

Does anybody have any idea how to split the algebraic loop in such a manner which does not corrupt the state estimation process in the observer and also does not increase the simulation time too much?

\$\endgroup\$
1
  • \$\begingroup\$ "Firstly the state estimation error in the observer is high despite the "small" value of the delay." Can you add more details to this statement ? Observer takes a copy of the plant input u as input as well as a copy of the plant output y as another input. If all of these (usalpha, isalpha, wm etc.) are delayed equally and fed to an observer, why would it have any effect on the estimation error ? Is the estimation error computed by comparsing to true value which, to be fair, should also be delayed if used for comparison with an observer running with a delay ? \$\endgroup\$
    – AJN
    Commented Oct 9, 2020 at 17:28

1 Answer 1

Reset to default
2
\$\begingroup\$

Algebraic loops occur when the present-state calculations depend on the present-state output - how can it provide a calculation if it does not have an initial condition?

How to break such loops can be as simple as placing a block that provides an initial output.

  1. Memory block (delay block in XCos)
  2. Transfer function \$\frac{1}{1\mu s + 1} \$

Both provide an initial output of 0 and thus permit the downstream calculations to initialize to then feed back into itself.

If your solver is fixed-step then a Memory block (Delay block) is the best solution as it delays the signal by 1 step. If however the solver is variable then a transfer function with a suitably high time constant is the better solution.

Where to place such block? on all feedbacks used by the control loop

\$\endgroup\$
2
  • \$\begingroup\$ thank you for your reaction. As far as I have understood correctly your answer you suggest in the first point directly what I have already done. As far as your second point you suggest to remove all the delay blocks in my diagram and instead of them use the first order low pass filter with small time constant (in respect to the time constants of the plant) placed before the S&H blocks. Is that correct? \$\endgroup\$
    – Steve
    Commented Oct 9, 2020 at 14:22
  • \$\begingroup\$ I have done what I have described above as in the second point and it resolved my issues. Thank you very much for your help JonRB. \$\endgroup\$
    – Steve
    Commented Oct 11, 2020 at 15:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.