0
\$\begingroup\$

I need help solving this task, if anyone had a similiar problem it would help me.

The task is:

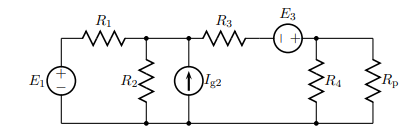
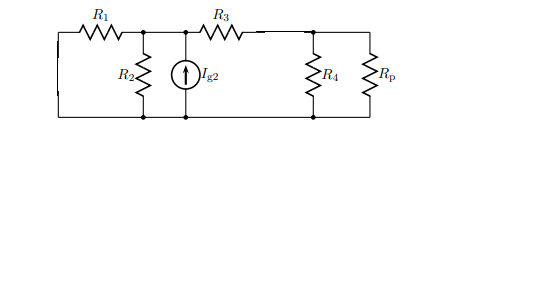
Determine the power dependence for an electrical circuit current through the receiver Rp in relation to all generators in the circuit. What is the contribution current through the receiver of each of the generators separately?

enter image description here

I tried this:

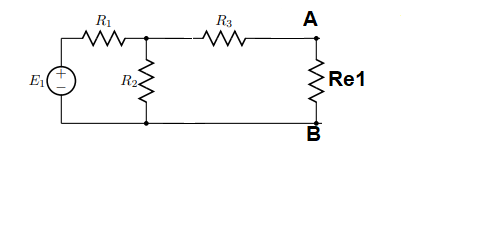
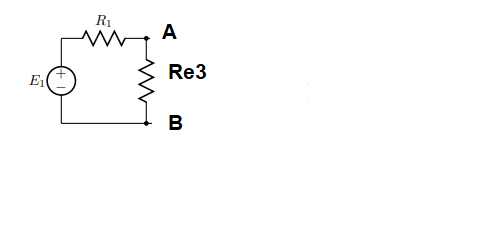
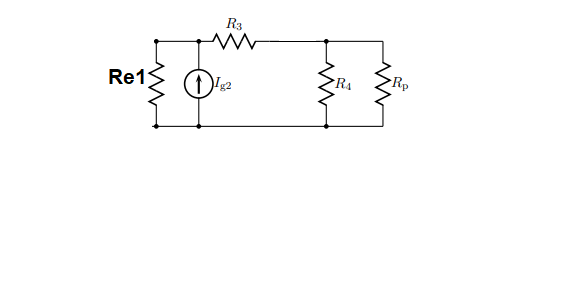
enter image description here

$$Re_1=\frac{R_p\cdot R_4}{R_p+R_4}$$

enter image description here

$$Re_2=Re_1+R_3$$

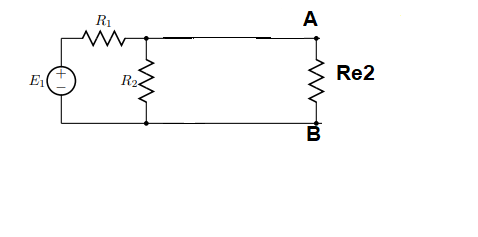
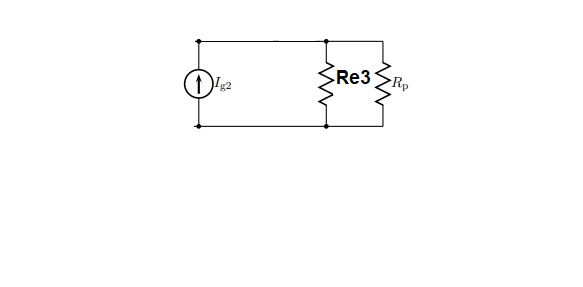
enter image description here

$$Re_3=\frac{Re_2\cdot R_2}{Re_2+R_2}$$

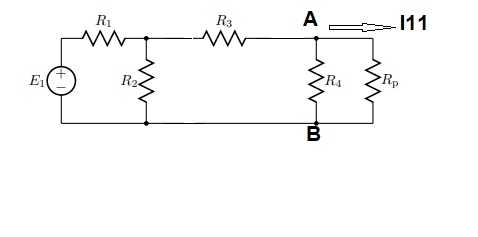
enter image description here

$$ U_{AB}=\frac{E_1}{R_1+Re_3}\cdot Re_3$$ $$I_{11}=\frac{U_{AB}}{R_p}$$

Is this right for I11?

Thanks in advance !

\$\endgroup\$
2
  • \$\begingroup\$ Add a circuit diagram for each of the resistances Re_n where you draw the reduced diagram after combining resistances. If you do that I think it is likely that you will spot your mistake yourself, and if not it will make it easier for other people to point out where you went wrong... Your solution is not correct. \$\endgroup\$
    – user173292
    Commented Jan 2, 2021 at 9:49
  • \$\begingroup\$ In my opinion I put: Re1= Rp II R4, Re2=Re1 serial R3, Re3= Re2 II R2 , Re4= R1 serial Re3? \$\endgroup\$
    – LogicNotFound
    Commented Jan 2, 2021 at 9:55

2 Answers 2

Reset to default
1
\$\begingroup\$

No, this is not correct for \$I_{11}\$. You combined a number of resistors along the way. Remember that when you combine two resistors in parallel that you lose information about the current through either of the two individual resistors. Likewise, when you combine resistors in series you lose information about the voltage across the individual resistors.

The current you solved for is the current from \$E_1\$ through \$R_1\$. You need to take this information and work your way back to the original circuit to find the current through \$R_P\$.

\$\endgroup\$
0
\$\begingroup\$

I tried a little differently, I'll start with the current generator: enter image description here

I tried this:

$$Re1=R1|| R2$$

enter image description here

$$Re2=R3+Re1$$

enter image description here

$$Re3=Re2+R4$$

enter image description here

$$I_{P1}=I_{g}\cdot \frac{R_{P}}{R_{P}+Re3}$$

\$\endgroup\$
3
  • \$\begingroup\$ You cannot add Re1 with R3 because they are not in series. \$\endgroup\$
    – G36
    Commented Jan 2, 2021 at 17:44
  • \$\begingroup\$ I don't understand then, is it a parallel connection? \$\endgroup\$
    – LogicNotFound
    Commented Jan 2, 2021 at 18:05
  • \$\begingroup\$ It is neither in parallel nether in series. You need to solve it symbolically or you just dos not shown us the component values? Because we can use a source transformation to further simplify the circuit. \$\endgroup\$
    – G36
    Commented Jan 2, 2021 at 18:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.