I understand how/why graded absorbers work - you achieve a match with free space by gradually varying the electromagnetic properties from "almost air" to strong absorption (either geometrically like pyramidal absorbers or through density like graded foam sheets).
How does this work for those (very thin compared to e.g. pyramidal absorbers) absorber sheets? How come they actually absorb energy and don't just reflect most of the waves due to this very sharp step?
These sheets are made of a loss material – it has a \$\mu_r=\mu'+j\mu''\$ whose imaginary part is particularly high.
Reflections happen when the boundary conditions enforce that – for example, a metal surface reflects well, because there can't be (much) E-Field inside the metal, leading to equation describing an incident wave having a solution where the surface-normal component of the pointing vector is inverted, but the parallel part remains untouched.
Perfect conductivity is not the case here.
The other option to enforce reflection would be attenuate the magnetic field (set it to 0 to get total reflection) inside the material – and that's what these materials do. However, they are mainly lossy, typically the real part \$\mu'\$ of the permeability is lower in magnitude than the (loss-indicating) imaginary part \$\mu''\$ for the frequencies you're trying to shield. Take this figure from Würth as an example:
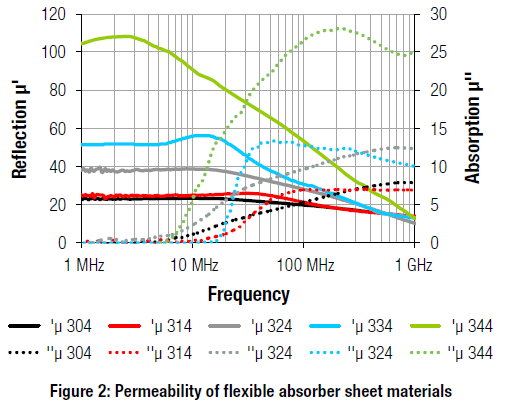
As you can see, there is a lot of reflection – but seeing that you do this to shield a box, typically that reflected power in the end "just" gets reflected around within the device and gets absorbed eventually (if you're not in extremely bad luck).
