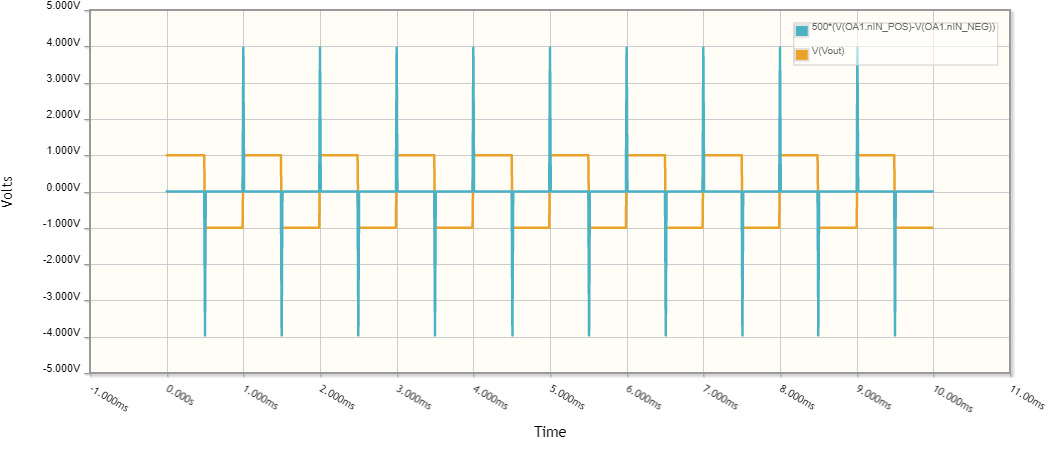
How come that the absolute output level when analyzing op-amp circuits is assumed to be \$V_{out} = Aol(V_{inp} - V_{inm})\$?
Simply put, it is not. No-one in the industry assumes this \$V_{OUT} = Aol(V_{inp} - V_{inm})\$ to be true or even useful for analyses embracing common mode parameters as is the case of your \$V_{IN,CM}\$ and \$V_{OUT,CM}\$.
But you are right, the handling of opamp's VTC in the presence of non-negligible common mode signal gain should be taken with special care: you should be familiar with the terminology, be able to read the relevant sections of datasheets, and trace the issues of input/output common-mode ranges violation and output latchup in your designs.
A good starting point to learn about common-mode issues might be Analog Devices' tutorials. MT-042 introduces the notion of Common-Mode Rejection Ratio:
If a signal is applied equally to both inputs of an op amp, so that
the differential input voltage is unaffected, the output should not be
affected. In practice, changes in common mode voltage will produce
changes in output. The op amp common-mode rejection ratio (CMRR) is
the ratio of the common-mode gain to differential-mode gain.
INLINE COMMENT
When citing their CMRR definition, I noticed that the MT-042 definition of CMRR (the ratio of the common-mode gain to differential-mode gain) is at odds with the generally accepted one (The CMRR is defined as the ratio of the powers of the differential gain over the common-mode gain).
Evidently, this is a typo, because further on, the CMRR value they use in the text and formulas, is the ratio of the differential-mode gain to common-mode gain, the inverse value w.r.t. their wording. Analog Devices, please correct this typo!
Back to the answer. Pay attention to formulas in Fig. 2: Calculating Offset Error Due to Common-Mode Rejection Ratio (CMRR) of this tutorial:
$$
\text {ERROR (RTI)} = {\frac {V_{CM}} {\text{CMRR}}} \\
V_{OUT} = \left[1+{R2 \over R1}\right] \left[V_{IN}+{\frac {V_{CM}} {\text{CMRR}}}\right]\\
\text {ERROR (RTO)} = \left[1+{R2 \over R1}\right]{\frac {V_{CM}} {\text{CMRR}}}
$$
These are the formulas for a closed-loop configuration. Listed in datasheets, the parameters CMRR and CMR characterize the rejection of a common-mode signal component in the total output of opamp.
The middle formula is valid for the approximation of \$R2/R1 \ll Aol\$. For \${1 \over R2} = 0\$ (no feedback, open-loop configuration), it should be written (https://en.wikipedia.org/wiki/Open-loop_gain, section Role in non-ideal gain)
$$
V_{OUT} = Aol \left[V_{IN}+{\frac {V_{CM}} {\text{CMRR}}}\right] = Aol \left[V_{IN}+{\frac {Acm} {Aol}}V_{CM}\right] = Aol·V_{IN} + Acm·V_{CM}
$$
because \${\text{CMRR}} = {\frac {Aol} {Acm}}\$ by definition. Also by definition, \$Acm·V_{CM} = V_{OUT,CM}\$, and \$V_{CM}\$ is a common-mode voltage at the input, \$V_{IN,CM}\$. In the end, we arrive at your formula \$Vout = Aol(V_{in+} - V_{in-})+V_{OUT,CM}\$.
Also pay attention to this phrase in the first paragraph
please note that there is very little consistency in this throughout the semiconductor industry with regards to the use of dB or ratio
values for CMR or CMRR.
which emphasizes the importance of following the terminology.
MT-041 considers practical basic points regarding the allowable input and output voltage ranges of a real op amp. These obviously vary with not only the specific device, but also the supply voltage.
To grasp a deeper understanding of CMRR, follow also tutorials and datasheets referenced in these two tutorials, as well as app notes, handbooks and tutorials of the other opamp manufacturers (Texas Instruments, STMicroelectronics), textbooks and EE course notes.