0
\$\begingroup\$

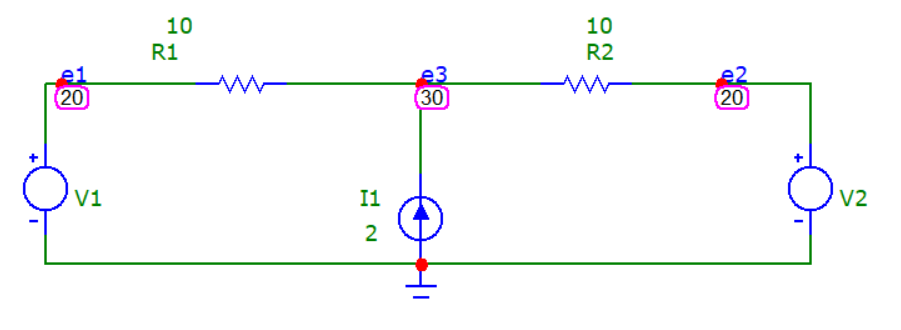
I'm trying to understand the behavior of the circuit below containing both voltage and current sources.

I don't really see how can we get 30 V at the node in the middle when we have only 20 V voltage sources. I assume this would be the effect of the current source...

Does anyone know how to analyze the circuit analytically to get the 30 V? I tried it without success.

enter image description here

\$\endgroup\$
6
  • 3
    \$\begingroup\$ An ideal current source can produce an infinite voltage. \$\endgroup\$
    – Finbarr
    Commented May 17, 2023 at 12:13
  • \$\begingroup\$ "Without success" doesn't help us to help you. Show the steps you tried and we can see where you went wrong. \$\endgroup\$
    – Finbarr
    Commented May 17, 2023 at 12:14
  • \$\begingroup\$ @Finbarr, So would you say that this situation (having 30 V at middle node) would not be realistic if I had a non-ideal current source? \$\endgroup\$
    – Likely
    Commented May 17, 2023 at 12:21
  • \$\begingroup\$ @Finbarr, Right, I should've added more details. Basically, I did a simple analysis where I said current will only be coming from the current source and divided equally between left and right since we have no voltage difference between left and right. This leads to V_R1 = V_R2 = 10*1 V and because current comes from current source only, voltage at middle node should be higher than left or right nodes. Hence, 30 V!! \$\endgroup\$
    – Likely
    Commented May 17, 2023 at 12:24
  • \$\begingroup\$ "Voltage source" should really be called a "fixed voltage" because in circuits like this the power is actually flowing into the so-called source. If it helps, you can imagine a battery getting recharged. \$\endgroup\$
    – Criticizing Israel not allowed
    Commented May 17, 2023 at 12:26

1 Answer 1

Reset to default
2
\$\begingroup\$

Well, using KCL we can see that:

$$0=\text{I}_1+\text{I}_{\text{R}_1}+\text{I}_{\text{R}_2}\tag1$$

The voltage across the resistors is given by Ohm's law:

  • $$\text{I}_{\text{R}_1}=\frac{\text{V}_1-\text{V}_{\text{e}_3}}{\text{R}_2}\tag2$$
  • $$\text{I}_{\text{R}_2}=\frac{\text{V}_2-\text{V}_{\text{e}_3}}{\text{R}_2}\tag3$$

So, we get:

$$0=2+\frac{20-\text{V}_{\text{e}_3}}{10}+\frac{20-\text{V}_{\text{e}_3}}{10}\space\Longleftrightarrow\space\text{V}_{\text{e}_3}=30\space\text{V}\tag4$$

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.