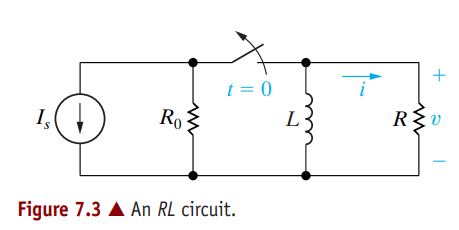
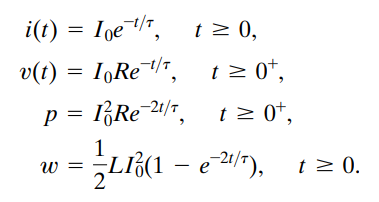I am curious about how the time bounds in power (t>=0+) and energy (t>=0) are developed in the natural response of RL and RC circuits. For the RL circuit, the natural response of the current is $$i(t)=I_0e^{-(R/L)t}=I_0e^{-t/\tau}, \, t\ge0$$ because there can be no instantaneous change in current to an inductor acting as a short-circuit when the inductor experiences a constant current for a long time. Because there is no voltage across the inductor initially, I see that the voltage is only bounded to time after the switch is opened since the voltage exactly at t=0 is indeterminate, $$v(t)=I_0Re^{-t/\tau}, \, t\ge0^+.$$ I am wondering why the power dissipated to resistive components then is bounded as $$p=I_0^2Re^{-2t/\tau}, t\ge0^+$$ whereas the energy delivered to resistive components is bounded as $$w=\int_0^\infty p\,dt = \int_0^\infty I_0^2 Re^{-2t/\tau}dt, \,t\ge0$$ in the basic circuit example where the inductor is suddenly removed from a current source and undergoes the natural response decay. In some way, I can see that the power should be bounded to the t=0+ side, if the voltage is not defined at t=0, however the power should be able to be derived with any of the equations, $$p=vi=v^2/R=i^2R$$ where I would think that I should be able to find the power with the last expression even at strictly t=0. Furthermore, I am most curious to know why the energy can be integrated from t>=0, if the power is only defined from t>=0+. If an definite integral gives the instantaneous rate of change between two bounds, I thought that the range of t should be only defined for t>=0+.
edit update: I think i(t) would be the current going through the resistor R branch, in the time interval t>=0 based on the picture that the text showed in Figure 7.3. The initial current in the circuit is the source current short-circuited through the inductor branch and is explained as, $$I_s=i(0^-)=i(0^+)=I_0, \, t<0$$ which is the current running through inductor that was acting like a short circuit right until the current source is removed, and so in the resistor branch, $$i(t)=i(0)e^{-t/\tau}=I_0e^{-t/\tau}, \, t\ge0$$ $$i(t)=0, \, t<0$$.