Wikipedia has an article about toroidal transformers and inductors. It is well known that an ideal toroidally wound coil has total magnetic field confinement due to symmetry in the case where the current through the coil is constant. That is, the magnetic field is confined within the toroid, and (with an ideally wound coil) is zero outside the toroid. However, the authors of the Wikipedia article seem to have misunderstood this to mean that there could be (with an ideal winding) total magnetic field confinement in a toroidally wound coil with time varying current.
The article (as of Jan. 2024) has a subsection entitled "Total B field confinement by toroidal inductors" which begins with the correct statement
In some circumstances, the current in the winding of a toroidal inductor contributes only to the B field inside the windings. It does not contribute to the magnetic B field outside the windings. This is a consequence of symmetry and Ampère's circuital law.[Emphasis added!]
It then goes on to describe "Sufficient conditions for total internal confinement of the B field". Unfortunately, it fails to note that a necessary condition, and therefore an essential part of any set of sufficient conditions, for total internal confinement of the B field is that the induced electric field must be time invariant. Ampère's circuital law, is not an unqualified law of classical electrodynamics, but is a special case of Ampère's circuital law with Maxwell's addition. Ampère's circuital law, by itself states that
$$\nabla \times \vec{B} = \mu_0 \vec{J}$$
(where \$\vec{J}\$ is the current density (due to the movement of charges).
Maxwell's addition changes Ampère's original law to
$$\nabla \times \vec{B} = \mu_0 \vec{J} + \mu_0\epsilon_0\frac{d\vec{E}}{dt}$$
Since the \$\vec{E}\$ field extends outside of the toroid, the term \$\mu_0\epsilon_0\frac{d\vec{E}}{dt}\$ will be non-zero outside of the toroid if the derivative of the \$\vec{E}\$ field is non-zero.
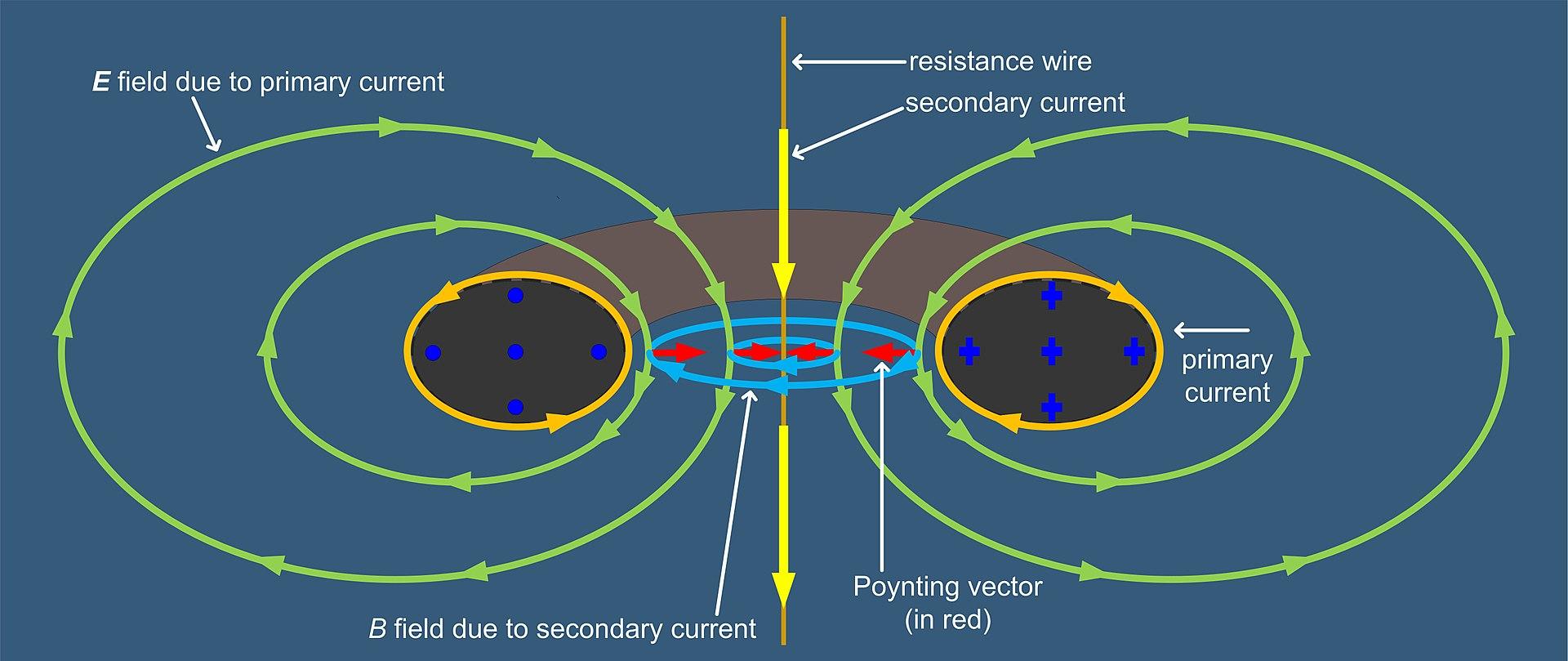
The claim that there is no magnetic field outside of the toroid implies that every Poynting vector outside of the toroid would be zero. But this provokes the question of how a toroidal transformer, with the secondary in the "hole" of the toroid could possibly work. The article confronts this by claiming the current in the secondary creates the magnetic field outside the toroid (i.e. in the hole).
This image from Wikipedia illustrates this argument.
However, I am quite convinced that there is a magnetic field in space around a sinusoidally energized toroidal inductor, including the hole, (with no transformer secondary), based upon my understanding of Ampère's Circuital Law with Maxwell's addition.
Is my understanding correct? Or is there an error in my understanding?