Why does the resonance peak dampen when an air cored coil is kept on a conducting material?

Question

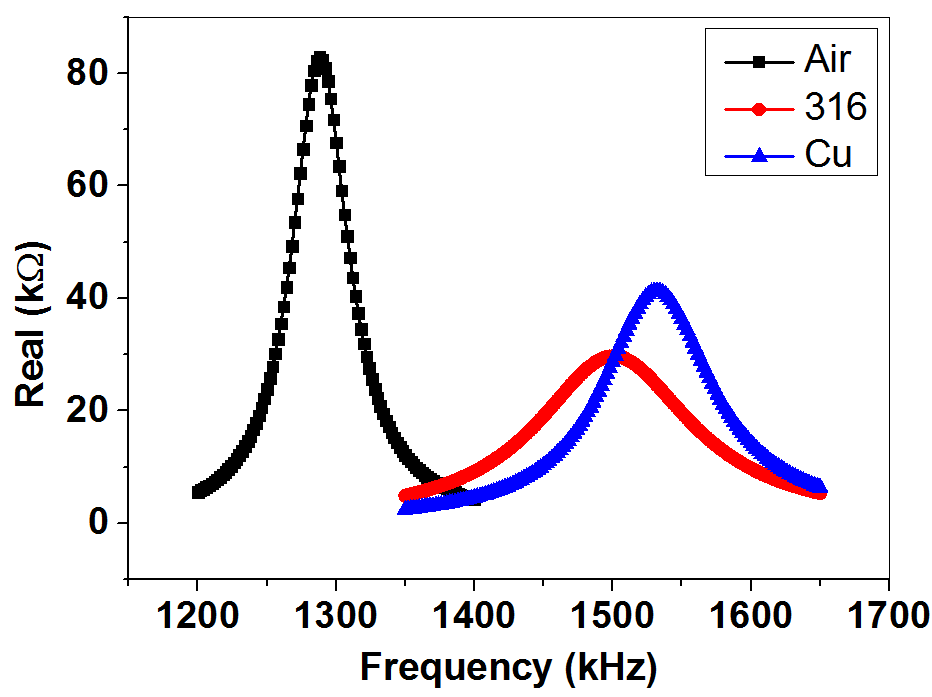
I was performing an experiment on an air cored coil, when I noticed that the resonance peak of the coil (Real(Z)) in air was sharp, but when kept on an SS316 plate, the peak dampened. When the coil was kept on copper, the peak amplitude increased slightly as well.

I understand that the increase in resonance frequency is due to the formation of eddy currents on the material reducing the flux within the coil, which reduced the coil inductance. So when inductance reduced, the resonant frequency increased.

But why does the impedance amplitude (max[Re(Z)])change?

Can anyone explain why?

Answer 1

If there are eddy currents in the material then some energy is going to be lost as heat. That loss of energy has the same affect as any other loss in the coil, it reduces the Q, which leads to a lower, wider resonant peak.

The difference in the affect of the different materials is due to different losses, the copper is showing less loss than the SS316.

Answer 2

When you use a "conducting" material with a coil in the neighborhood,
you create a "coupled" LC circuit with a "low" factor of "coupling" kc.

Here is the behavior of such a circuit.
When coupling is higher, resonance frequency is higher ... and "peak" is lower.
Here are the curves for a coupling factor kc = 0 until 0.5, step 0.1.

Answer 3

Eddy currents in the plate not only reflect back to the exciting coil to change its inductance, but the eddy currents in the plate heat it up, due to the plate's internal resistance.

The power dissipated in the plate show up as increased losses in the exciting coil. Your model of the inductance apparently is a parallel LRC circuit, where inductor losses show up as the parallel R.

A perfect inductor would have infinite parallel resistance.