Particular current divider

Question

Consider this circuit:

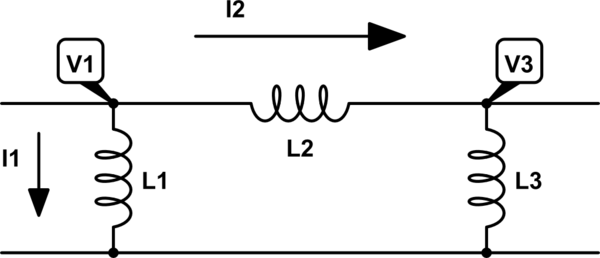
^{simulate this circuit – Schematic created using CircuitLab}

(this part is preceeded and followed by other components).

$$I_1 + I_2 = \displaystyle \frac{V_1}{sL_{12}} - \frac{V_3}{sL_{12}} \left( \displaystyle \frac{L_1}{L_1 + L_2} \right)$$

with

$$L_{12} = L_1 || L_2 = \displaystyle \frac{L_1 L_2}{L_1 + L_2}$$

How can this relation be obtained? Any hint?

The ratio \$ \displaystyle \frac{L_1}{L_1 + L_2} \$ seems a voltage divider; but I can't see anything similar. Moreover, \$ L_1 \$ and \$ L_2 \$ are not parallel.

Answer 1

Just realize that \$\frac 1 {sL_{12}} (\frac {L_1} {L_1+L_2})=\frac 1 {sL_2}\$

Answer 2

Maybe it would be more direct to see that $$I_1 + I_2 = \displaystyle \frac{V_1}{sL_{1}} + \frac{V_1 - V_3}{sL_{2}}$$ and that $$L_{1} = \displaystyle L_{12} \left(\displaystyle \frac{L_1+L_2}{L_2} \right) $$ From there it's just algebra to see that it's equivalent to what you've got.