High input impedance devices - induced noise sensitive

Question

I am currently reading about 4-20mA current loops and the reason we use them .As stated there , using voltage to transmit signals we get voltage drops over long cables due to cable resistance. We can use high input impedance devices to circumvent signal loss , however these devices are sensitive to noise. So my question is why high impedance input devices are sensitive to noise and low are not? I have read other topics on this subject but none of this could explain it clearly.

Please give me some explanation. Thank you in advance

Answer 1

This is what the article says: -

By using current signals and low impedance data acquisition devices, industrial applications benefit from better noise immunity and longer transmission cable lengths.

The article also says, in relation to devices that produce voltage signals, that: -

These devices are sensitive to the noise induced by nearby motors, conveyor belts, and radio transmissions.

Basically it's true but there are some caveats. Consider the noise induced by motors and for this, I reckon induction motors are a likely culprit. They produce magnetic fields that can induce an interfering voltage in a cable whatever the signalling type is.

When voltage signalling is used, the interfering voltage is additive to the signal just like batteries in series are additive. This adds an error.

When current signalling is used AND, providing the induced voltage is not several volts, the current flowing in the cable (due to the signal) remains exactly that current and no voltage interference is seen at the receiving end - this is because of the high-compliance of the 4-20mA current source: -

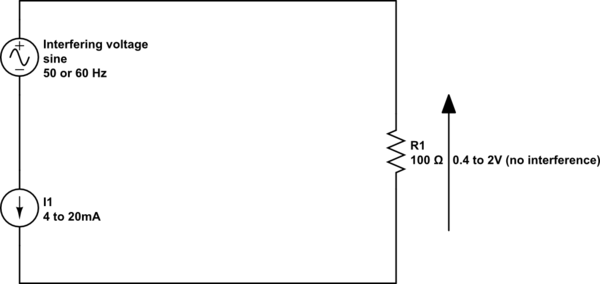
^{simulate this circuit – Schematic created using CircuitLab}

Hopefully you can see that for a high-compliance current source, interfering voltages that arise in series with the current loop have little effect.

Where does this start to go wrong: -

1. If the interference is large enough to cause the current loop transmitter to fall-out of high compliant sinking or sourcing of current
2. When the frequency is high and the current source/sink is unable to provide a high-compliance.

(1) The compliant current source may need a few volts across it to maintain performance and if the series voltage causes the minimum voltage to drop-below this point there will be glitching introduced onto the signal.

(2) At high frequencies, the compliance will change from theoretically infinite resistance to more like a small value capacitor (due to the transistors and chips in the device). This will allow high frequency interferers to circulate a current through the 100 ohm receiver (R1).

If low frequency signalling is used (with appropriate low-pass filtering at the receive end) HF interference can largely be avoided and it is advised to use screened/shielded twisted pair cable.

High energy E-field interference (as opposed to magnetic interference) tends to be seen as a voltage in parallel with the two wires and this also directly impinges on R1 so shielding and filtering is needed.

Answer 2

I like to give simplistic answers first. Then if more technical or details are needed, they can come later. - - - Imagine that a powerful external source of energy (magnetic, electromagnetic, nearby radio transmitter, whatever) had the ability to induce a current of 100uA (micro-amps) onto your signal leads. If you have a high input impedance, say 100 K ohms, the induced voltage (noise) would be 10 volts (ohms law). - - - If you had a low input inpedance (250 ohms common in 4-20mA system), the 100uA would result in .025 volts (25mV noise) impressed upon your signal.

Further, 100uA is only 2.5% of your lowest signal of 4mA in 4-20mA system.

Answer 3

The explanation you've been given is not very accurate. Imagine a typical voltage-output instrument (say 1-5V). The output impedance of the source might be well under an ohm, while the receiver might have a 1M ohm input impedance. So the two in parallel are actually very low impedance, yet the wire resistance matters little because it's high in relation to 1M.

Now, let's look at your current loop. The source, being a current source, is very high impedance, well over 1M for a precision instrument. The receiver might be 250 ohms to give a 1-5V voltage. So the two in parallel are 250 ohms, which is much higher than < 1 ohm. The wire resistance doesn't matter much until you run out of compliance voltage on your current source.

So, what gives?

The voltage circuit has a (very) low impedance at the transmitter, and a high impedance at the receiver. The current loop has a (very) high impedance at the transmitter and a fairly low impedance at the receiver. In between is a lot of wire that can have common mode or normal mode signals induced. If there are no ground loop issues (both sides perfectly isolated), then common mode noise in the cable is ignored in both cases. Normal mode noise will be ignored by the current loop, but will be passed on directly by the voltage device. Twisted pairs will have very little normal mode noise, but not zero.

Normal mode noise is like two voltage source in series with each wire (that add). Common mode noise is the same, but the voltage sources cancel each other.

A modern reason to use 4~20mA current loops is that the transmitter can sometimes be powered from the 4mA (with a bit to spare) so that only two wires are required (no extra power supply). That also facilitates making the transmitter "intrinsically safe" by limiting the energy so that it cannot cause an unsafe condition in a hazardous atmosphere.

Answer 4

The capacitance of the cables forms a low pass filter with the high input impedance. The higher the impedance, the higher the upper limit on the passing frequencies is, thus allowing more noise.