simulate this circuit – Schematic created using CircuitLab
For t > 0 I have determined the transfer function:
$$ \hat{H}(s)=\frac{\hat{Y}(s)}{\hat{F}(s)}= \frac{s}{s^{2}+s+1} $$
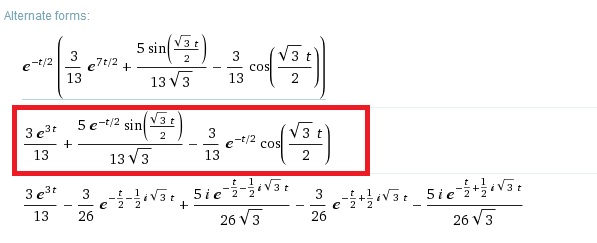
Now I need to find the zero-state response for t > 0 if: $$ f(t)=e^{3t} $$
And the zero-input response for t > 0 if: $$ y(0^{-})=1V \ , \ i(0^{-})=0 $$
Are the ZSR and ZIR related to h(t) or would it be i(t)? I know that for ZIR F(s)=0. But what piece of this problem is the response?
For the zero state: Find
$$ F(s) =\frac{1} {(s-3)} $$
Which is computed by taking the Laplace transform of course. Now, multiply F(s) with your transfer function. You will have $$ Y(s) = \frac{s} { (s-3)(s^2+s+1)} $$
Now simply, use partial fraction and take Laplace inverse to find y(t)
Solution on wolframalpha:
As for the zero state response: You can find the differential equation by doing the cross multiplication As for s^2 is second derivative and "s" is first derivative: $$ y' ' + y' + y = f ' $$ Take Laplace transform again considering initial conditions: $$ s^2Y(s) - sy(0) - y'(0) + sY(s) - y(o) + Y(s) = sF(s) - f(0) $$ No as for the input F(s) = 0 (for zero input)
So, $$ Y(s) = \frac{sy(0) + y'(0) +y(0)}{(s^2 +s +1)} $$ Now sub for the initial conditions and take Laplace inverse to find y(t).
