0
\$\begingroup\$

If a single wire is moving at \$v\$, inside a magnetic field \$B\$, with length \$L\$ then induced EMF is:$$\epsilon\ = -vBL$$

That is for a vertical wire that's motion is perpendicular to a magnetic field, the direction of induced \$I\$ is quite simple to find.

What about this diagram:

enter image description here

I know there is induced EMF, however, the wire is tilted. Is $$\epsilon\ = -vBL$$ still valid or must I add a trig-function due to the change in orientation?

There is no current flow because this is an open circuit, however, if connected to a circuit from the upper and lower ends, what would \$\epsilon\$ = ?

\$\endgroup\$
1
  • \$\begingroup\$ Write it as a vector equation and break the velocity into a sum of perpendicular and parallel terms. The rest multiplies out and you have your answer. \$\endgroup\$
    – caveman
    Commented Jan 16, 2015 at 23:37

2 Answers 2

Reset to default
2
\$\begingroup\$

There are two approaches of the same phenomena. For an observer located inside the wire, the sources of magnetic field are moving, and therefore, an electric field appears according to Faraday's Law, generating an EMF \$\epsilon\$. On the other hand, an observer located outside the wire (in the same inertial frame of the magnetic field source) will only see the charges (electrons and protons) on the wire moving at the velocity \$\overrightarrow{V}\$. Those charges will experiment a Lorentz force \$\overrightarrow{F}=e\left(\overrightarrow{E}+\overrightarrow{V}\times\overrightarrow{B}\right)\$ and will accumulate at the tips of the wire which creates a potential difference \$\epsilon\$ between the tips, same as the EMF calculated by the other approach.

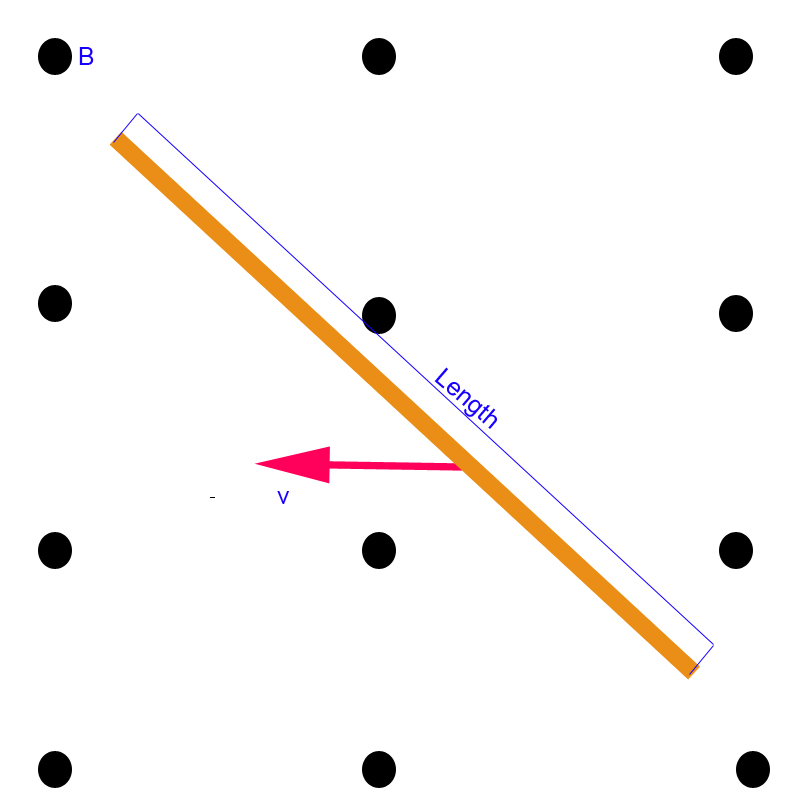
Therefore, since that potential difference \$\epsilon\$ is proportional to the component of the Lorentz force parallel to the wire, the inclination of it will decrease \$\epsilon\$.

In order to obtain an equation, first consider the wire perpendicular to \$\overrightarrow{V}\$. It can be demonstrated that \$\epsilon = \left|\overrightarrow{V}\right|\left|\overrightarrow{B}\right|L \$ (as you said). Now, consider the wire inclinated an angle \$\theta\$ with respect to \$\overrightarrow{V}\$. The component of the Lorentz force in the direction of the wire will be \$\left|\overrightarrow{F}\right|\sin(\theta)\$, so, the resulting potential difference between tips is \$\epsilon = \left|\overrightarrow{V}\right|\left|\overrightarrow{B}\right|L \sin(\theta)\$

\$\endgroup\$
1
\$\begingroup\$

The actual (physics) expression is v X B (where X is the cross product between the two vectors), So there is an angle in there vBl*sin (theta)... putting the angle at the top of your drawing.

\$\endgroup\$
3
  • \$\begingroup\$ That makes sense, a wire at a slope would have less EMF than a vertical wire, and the more it tilts the smaller the angle the less EMF induced on that wire. Hence a horizontal wire would have 0 emf. \$\endgroup\$
    – Pupil
    Commented Jan 17, 2015 at 0:22
  • \$\begingroup\$ The cross product is fun. What happens if you spin a square coil in a constant field? \$\endgroup\$
    – George Herold
    Commented Jan 17, 2015 at 1:36
  • \$\begingroup\$ Would cancel out, equal and opposing parts connected in opposition. \$\endgroup\$
    – Pupil
    Commented Jan 17, 2015 at 2:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.