Would oversampling reduce in-band signal noise floor?

Question

I understand that oversampling will reduce quantization noise floor.

Would oversampling also reduce the noise floor of the noises in band of interest?

*******EDIT 20150320*********

The correct answer is from the comment of Brian Drummond.

Oversampling combined with proper DSP will help reducing quantization noises and thereby increase ADC resolution, under given conditions. Details can be found at: http://www.silabs.com/Support%20Documents/TechnicalDocs/an118.pdf

However, it cannot reduce in-band noises.

Answer 1

You can't reduce noise that is already present at base band but, by appropriate filtering and over-sampling you can reduce out of band noise aliasing into the base band when the ADC converts. Aliasing: -

Here, a signal (in red), is grossly under-sampled and it produces the blue signal. The blue signal is not representative of the original red signal and this is called an aliased signal. This is due to not designing a secure-enough anti-alias filter before the ADC. The anti-alias filter would attempt to significantly reduce the amplitude of the red signal before it entered the ADC.

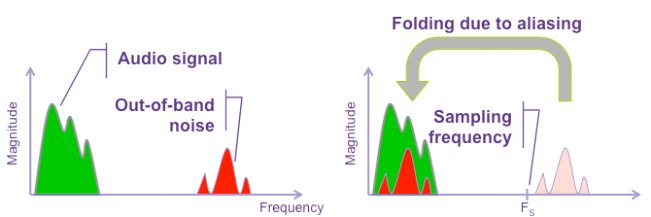
It's the same for noise - noise above the Nyquist frequency of an ADC can alias down into the base band after conversion and this adds to the original base band noise. Here's a picture of several signals of which one is below the Nyquist frequency, the rest becoming aliased: -

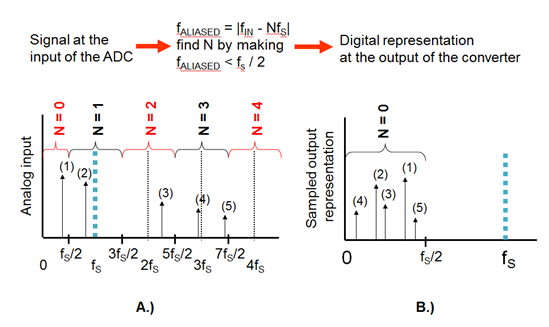
Looking at the left picture, signal(1) is converted and remains at its correct frequency on the right hand picture but, all the other signals (those above fs/2) are aliased down into the base band.

So, if you use over-sampling, the anti alias filter becomes more effective and signals 2, 3, 4 and 5 become much more heavily filtered by the anti alias filter. There will still be some aliasing artifacts but significantly reduced.

Noise is just a wide-band signal and is treated no differently by the ADC: -

Answer 2

Oversampling (i.e. sampling at frequencies significantly higher than the Nyquist rate) reduces neither the in-band noise nor the quantization noise. It makes better antialiasing possible, which is important, but that's all it does.