Insight on analog and digital Lock-in amplifiers operation principle?

Question

As far as my knowledge goes:

in the analog case, the discrimination of the, low S/N ratio, signal is done by (demodulation) comparing it to local oscillator with almost identical frequency and then passing it through low pass filter.
So it must be that, if the input signal is: \$\sin(a)\$ and the reference signal is: \$\sin(b)\$ (almost equal), are multiplied and integrated, long enough, the result will be: \$\frac{(sin(a+b)+sin(a-b) + phase)}{2}\$, and after the filter only the low frequency component will be left, i.e.: \$\sin(a-b)\$.

Question:

More complete view in the operation principles (of both the digital and analog amplifiers) and the stages of signal processing would be greatly appreciated.

Answer 1

I know two ways to do a lockin (you can implement either with analog or digital parts..) The first involves multiplying two signals together. (one signal and one reference) The second is +1/-1 gain switch of the signal. The switch is controlled (flipped) by the reference. The low pass follows either operation.

Edit: Of course I forgot the phase adjustment. (or do both phases ala Spehro above.)
If you have a digital 'scope you can make a poor man's lockin. Signal into one channel and the reference into the other, trigger the 'scope off the reference and average.

Edit2: schematics. For the digital version I suppose you just digitize both the signal and reference and do the math in software. (I'm sure there are some tricks). In analog, the classic "lock-in" IC is the AD630 there are some nice schematics in the linked data sheet.

Answer 2

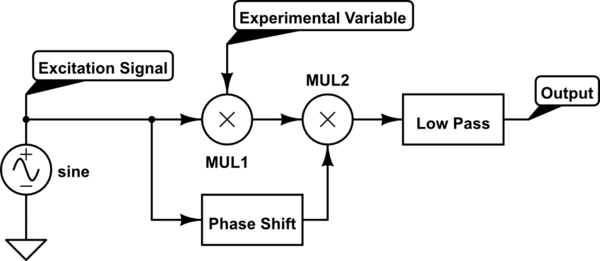
I have built several lock-in amplifiers: both analog and digital. The mathematical theory is identical for the digital and analog case. The technique is based on time-domain multiplication:

^{simulate this circuit – Schematic created using CircuitLab}

• We produce an excitation voltage with a local oscillator. It is usually a sine wave, but could also be a square wave.
• We use that voltage to excite some experiment in which the amplitude of the voltage is effected by the thing I want to measure. Alternatively we can measure other signals not produced in this way, but at that point a phase sensitive detector is probably not desired.
• We then demodulate the signal with a multiplier MUL2. We can optionally shift the phase of the reference signal to produce a quadrature indication. It is common to do both at the same time.
• The product is low pass filtered in order to remove the content at frequencies other than the frequency of interest (noise, the excitation frequency, the experimental signal at other phases, ...)

I find it easiest to think of in the frequency domain as convolution. See a quick explanation in this video.

One can implement the above theoretical concept either with analog components, or by using converters (a DAC after the excitation signal is generated and an ADC to read the output of the experiment) and then digitally computing the phase shifted reference, product, and low pass filter (perhaps a moving average). The math doesn't care about the digitization, provided that the samlping rate is adequately fast.