Differential input voltage of op-amp with feedback

Question

I learned that either positive or negative feedback on an op-amp makes voltages at a noninverting input and an inverting input same.

Then, I think I don't need to care about absolute maximum value of differential input voltage. Is it right?

I have TL972IP and I am trying to convert a bipolar sqaure wave into a unipolar square wave swining 0 V and 5 V.

And I have to determine an amplitude of the input square wave. As regarding the absolute maximum value, a signal will swing -0.4 V and +0.4 V.

But, if my guess--the absolute maximum value can be bypassed, ignored with feedback--is right, I can increase the amplitude a little bit more, from +- 0.4 V to +- 1 V.

By doing this, I can reduce the gain, which results in increase of bandwidth since GBWP = Gain * BW and it is constant.

ADDED

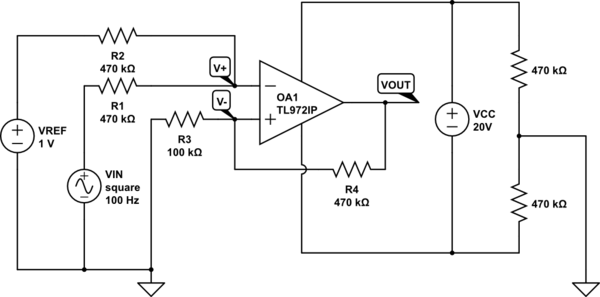
^{simulate this circuit – Schematic created using CircuitLab}

In the case above, I think V+ and V- will be the same. Then the differential input voltage will be zero. Thus, I don't need to care about the absolute maximum rating of the differential input voltage. I only need to care about other ratings and conditions. Is that right?

Answer 1

The maximum input differential voltage is a breakdown rating on the input stage structure and cannot be ignored; it is in the datasheet for the very good reason that exceeding this may damage the device.

If you power this device from (for instance) +5V and ground, then the output can only slew to within a few millivolts of ground, so if your input goes to -1V, you will definitely be violating the maximum rating of the device.

If you want to convert a bipolar square wave to a unipolar one, a series diode (to prevent the input going negative) and a comparator may serve you better.

Note that the gain bandwidth product of an amplifier is not directly tied to slew rate, which determines what the output looks like. To remain within the small signal region (i.e. the output is not in slew rate limit), the following rule applies:

fmax = Sr/2πVp where fmax is the highest frequency in your signal, Vp is the peak voltage at the output and Sr is the slew rate from the datasheet.

Answer 2

You must maintain the differential input voltage below the absolute maximum value of 1V. Also current may flow if there is substantial differential input voltage.

It will not damage the op-amp if you limit the current as you have (with relatively high-value resistors), so I think the circuit is okay since the internal network across the input pins will draw current and limit the voltage to a safe value.

What you have drawn is a comparator with hysteresis, of course, not an amplifier. I would suggest reducing the 470K rail splitter resistors (the two on the right) quite a bit, maybe to 1K each, since the 'ground' will not be very stable the way you've drawn it.