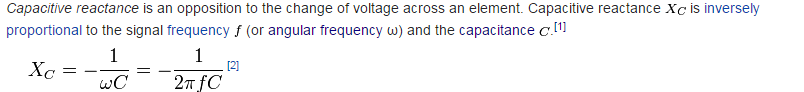Is capacitor reactance [sometimes] defined with negative sign?

Question

Wikipedia currently claims so

but I've looked in 6 books via Google Books and it's not defined like that, i.e. it's just

$$ X_c = \frac{1}{\omega C} = \frac{1}{2\pi f C} $$

Is Wikipedia full of nonsense on this, is that just a fringe def, or somehow all six books I've checked via GB just happen to contradict that and some EE bible actually defines it with a minus sign like that? Wikipedia cites one book and one unverifiable website; I can't access that book right now. The ones I've checked: 1 2 3 4 5 6. Note that depending on your Google luck you may not be able to see all of these. And I've checked the 3rd ed. of the Art of Electronics by H&H; it also gives it the positive way (on p. 42).

I was actually able to verify a newer edition of the textbook cited in Wikipedia, and indeed it defines it that way with a negative sign. So I'm guessing it's one of those egg-end issues. Still I'm curious if there are any EE standards (IEC etc.) that take a stance on this. Perhaps someone knows...

---

I've accepted Adam's answer as good enough (and I've fixed Wikipedia too), however if someone knows more about IEC, IEEE or whatever other standard bodies might have said about this, please contribute...

And from the Wikiality department, that article has changed quite a few times it seems; back in March it gave the positive definition.

Answer 1

The impedance of a capacitor is given by the formula:

$$Z_C = \frac 1 {j \omega C} = \frac 1 {j 2 \pi f C}$$

where \$j = \sqrt{-1}\$. It takes a bit of algebra to get the negative sign:

$$\frac 1 j = \frac j j \cdot \frac 1 j = \frac j {j^2} = \frac j {-1} = -j$$

$$Z_C = \frac 1 j \cdot \frac 1 {\omega C} = \frac {-j} {\omega C}$$

The reactance is the imaginary part of the impedance, so you could say that it's:

$$X_C = Im\{Z_C\} = -\frac {1} {\omega C}$$

If you want to combine series inductors and capacitors into a single equivalent reactance, the sign matters.

But what the \$-j\$ really represents is a -90 degree phase shift between the capacitor's voltage and current (current leads voltage):

(source)

If you want to talk about the magnitude and phase shift effects of the reactance separately, then you can drop the negative sign:

$$Z_C = \frac 1 {\omega C} \angle -90^\circ$$ $$X_C = |Z_C| = \frac 1 {\omega C}$$

I wouldn't say either of them is wrong. They're different ways of simplifying to avoid complex numbers. Any simplification will be right at some times and wrong at other times. You need complex numbers to get the full picture, but that's a lot of math for a college freshman or the general public. So introductory books often deal with magnitude and phase effects separately.

Your citations are good examples of this. The first book gives the positive reactance but then tells you to combine inductance and capacitance like this:

$$\text{Resultant reactance} = X_L - X_C = 2 \pi f L - \frac 1 {2 \pi f C}$$

The second book gives the positive formula and describes phase shifts in the next paragraph. The third book (Electronics for Dummies) is a deliberate simplification. The fourth book describes the phase shift in terms of phasor diagrams on the next page. The fifth book mentions phase shifts in the box below the definition, but says that the book omits inductors entirely. The sixth book describes phase shifts on the page after the definition.

Answer 2

I think it is mathematically not correct to say \$j = \sqrt{-1}\$. It is correct to say \$j^2 = -1\$. That is all you need in these calculations. Reason: taking a complex root is multiple valued, but squaring is undoubtful clear. So avoid taking a root if you can do it with squaring.

And yes, I do certainly prefer to consider the reactance of a capacitor \$ C \$ to be negative to express the phase difference between current and voltage, compared to the same things in/on a inductor.

In my opinion it is even better to distinguise between the magnitude and the value of a reactance: use the caret symbol to differentiate between the two, like we already do for a voltage or current: \$ V \$ and \$ \hat V \$ and \$ i \$ and \$ \hat i \$. It is hard to see these special characters in plain text mode, but with this special mathematics friendly format it really looks nice.

I suggest we do the same with the \$ X \$, so for a capacitor \$ C \$ define \$X = -\frac{1}{\omega C}\$ and \$|X| = \hat X = \frac{1}{\omega C}\$ and from now on when you want to address the magnitude of the reactance, use \$ \hat X \$. Problem solved.

And talking about reactance means we should also talk about susceptance, which is not the invers of reactance but the imaginairy part of the admittance.

Example: if complex "impedance" \$Z = R + jX\$ with real \$ R \$ = "resistance" and real X = "reactance", then the complex "admittance" \$ W \$ defined as \$ W = 1/Z \$ can be again written as \$ W = G + jY \$ , with real \$ G \$ = "conductance" and real \$ Y \$ = "susceptance". Note that in these definitions the \$ R, X, G \$ and \$ Y \$ are all real numbers and may carry a sign, even \$ R \$ and \$ G \$ in general.

Working this out gives:

$$ \begin{align} W & = \frac {1}{Z} \\ & = \frac {1}{R+jX} \\ & = \frac {1}{R+jX} \cdot \frac {R-jX}{R-jX} \\ & = \frac {R-jX}{R^2+X^2} \\ & = \frac{R}{(R^2+X^2)} + j \cdot \frac{-X}{R^2+X^2} \\ & = G+jY \end {align} $$

or the imaginary part (the "susceptance") of \$ W \$ is:
$$ Y = - \frac{X}{R^2+X^2} $$ Note that susceptance \$ Y \$ obviously will have a positive value if reactance \$ X<0 \$ .

A special case is the capacitor \$ C \$ of which the resistance \$ R=0 \Omega \$ and rectance \$ X=- \frac{1}{\omega C} \Omega\$ . Note the negative sign: this carries information about the phase difference between voltage over and current through the \$ C \$ .

Filling in these values gives: $$ Y = - \left( \frac{-\frac{1}{\omega C}}{0^2 + \left( - \frac{1}{\omega C} \right) ^2} \right) = \frac{ \frac{1}{ \omega C}}{ \left( \frac{1}{ \omega C} \right) ^2} = \omega C $$ which, as was expected, is a positive number: \$ Y > 0 \$

Note, that for a capacitor \$ C \$ the reactance \$ X = - \frac {1}{Y} \$ , where \$ Y \$ = the susceptance of the \$ C \$ .

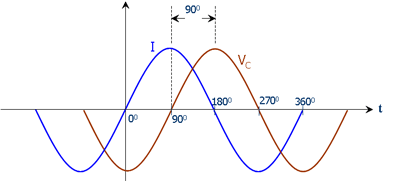
Note also, that the change in sign means the phase has flipped too and that is as it should be: because on a capacitor its voltage over it is 90 degrees lagging behind the current through it.

If you look at the reactance ("AC-resistance") of a capacitor) \$ \frac {V_C}{I_C} = Z_C \$ you should get a negative sign reflecting that the voltage is lagging relative to the current and that makes that the reactance \$ X \$ of a capacitor \$ C \$ should have a negative sign.

Looking at \$ \frac {I_C}{V_C} = Y_C \$, you are looking at the current relative to the voltage and because the current is 90 degrees ahead of the voltage, the susceptance ("AC-conductance") of the capacitor \$ Y_C \$ should be positive.

Answer 3

The minus sign is an indication of the phase relationship to the applied signal. There are cases where one is only interested in the reactance and its effect on simple observations such as current. Just as I=E/R, here I=E/X, and if the current is all you want to know about (think appliances) then you aren't concerned with any phase relationship and can ignore the sign. That's why you often don't see it in introductory material.