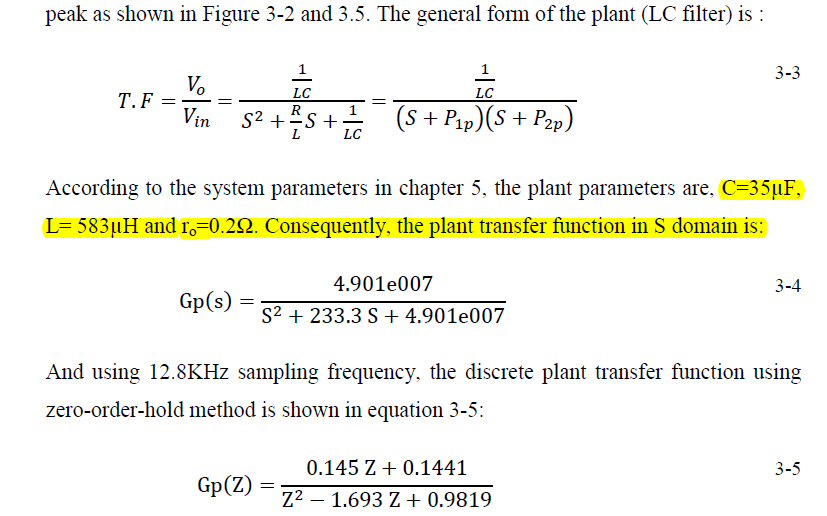
I am just reading a research paper on matrix converter and i came through transfer function conversion from s domain into z domain. which i am unable to understand. how the equation 3.4 is transferred into 3.5 using zero hold method. can anyone explain step by step? attached is the screenshot.
2 Answers
The Laplace TF of a ZOH is usually taken as \$\small \dfrac{1-e^{-sT}}{s}\$, where \$\small T\$ is the sampling increment. Multiply this by \$\small G_p(s)\$, giving $$\small G^*_p(s)=(1-e^{-sT}) \frac{G_p(s)}{s}$$
Since \$\small e^{-sT}\$ and \$\small z^{-1}\$ represent pure time delays of \$\small T\$ in the s and z domains, respectively, we may write:
$$ \small 1-e^{-sT} \rightarrow \dfrac{z-1}{z}$$
The z-transform of the remaining term, \$\small \dfrac{G_p(s)}{s}\$, may be obtained from the tables {http://lpsa.swarthmore.edu/LaplaceZTable/LaplaceZFuncTable.html}
Working (very) approximately from your equations to check the result, the original Laplace TF may be simplified to: $$\small G_p(s)\approx\dfrac{ \omega_n^2}{s^2+\omega_n^2}$$
with \$\small \zeta=0.017\approx0\$; \$\small \omega_n=7\times 10^3rad/s\$; \$\small \omega_n\:T=0.547rad\$ \$\small\approx30^0\$.
Hence, after z-transforming \$\small \dfrac{G_p(s)}{s}\$ and multiplying by \$\small \dfrac{z-1}{z}\$, the approximate z-TF is: $$\small G^*_p(z)\approx\frac{0.13z+0.13}{z^2-1.73z+1}$$
Which compares favourably with the answer you give.
First, there is a typo in the question (or the source). With the given values of \$R\$, \$L\$, and \$C\$, the transfer function is $$H(s)=\frac{4.90076\times 10^7}{s^2+343.053 s+4.90076\times 10^7}$$
Comparing this with $$\frac{w^2}{s^2+2 \zeta w s+w^2}$$ we get $$w=\sqrt{4.90076\times 10^7}=7000.54$$ $$\zeta=\frac{343.053}{2 w}=0.0245$$
The sampling period is $$T = \frac{1}{12800}=0.000078125$$
Now we are ready to compute the zero-order hold equivalent which is $$\frac{z-1}{z}\mathcal{Z}\left(\frac{H(s)}{s}\right)$$
Using the same table used by Chu, we get this to be
$$\frac{z-1}{z}\left(\frac{z}{z-1}-\frac{1}{\sqrt{1-\zeta ^2}} \frac{ z^2 \sqrt{1-\zeta ^2} +z e^{-\zeta T w} \sin \left(\sqrt{1-\zeta ^2} T w-\cos ^{-1}(\zeta )\right)}{z^2-2 z e^{\zeta (-T) w} \cos \left(\sqrt{1-\zeta ^2} T w\right)+e^{-2 \zeta T w}}\right)$$
Plug in the values for \$w\$, \$\zeta\$, and \$T\$ (I used Mathematica) to get the numerical result
$$ \frac{0.14458 z+0.143282}{z^2-1.68569 z+0.973555}$$