Equivalent Circuit for the RLC circuit to find the transfer function

Question

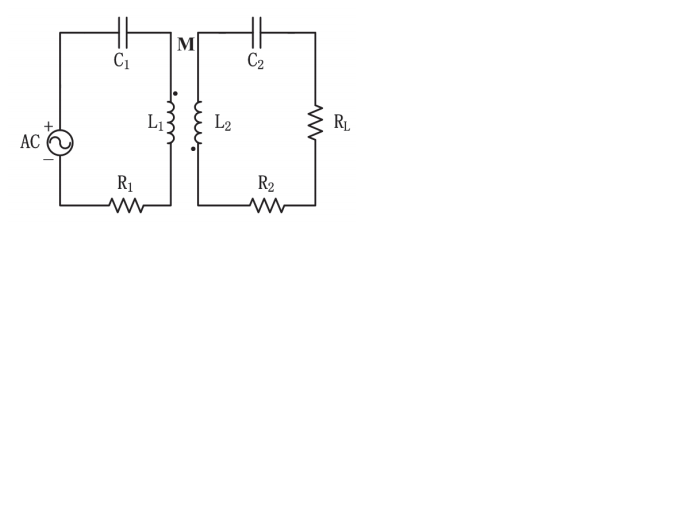
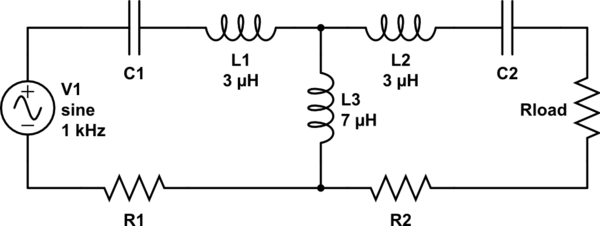
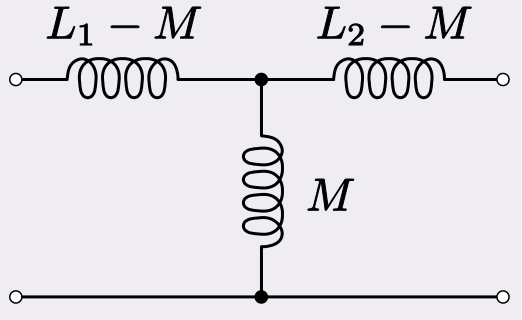
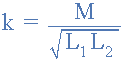I have a series RLC circuit with mutual inductance between the transmitter end and the receiver end.

The circuit is

I am trying to formulate a simple transfer function. I can formulate the transfer function by applying KVL to both transmitter loop and receiver loop and solving them to derive, load voltage/input voltage.

But I am a beginner in circuit theory and till now I have never come across finding the transfer function of a circuit with mutual inductance.

So if somebody can guide me in solving it. or if you know any example then please let me know.

Thanks in advance.

Answer 1

Mutual inductance (M) and coupling factor (k): -

So if you have two inductors of 10uH each coupled at 70% then M = 7uH.

This means that the 10 uH coupled inductor becomes a 100% coupled transformer with leakage inductance of 3 uH in each limb.

Because the transformer is 1:1 (in my example) you can simply electrically connect the secondary components (including the extra series 3 uH leakage inductance) across the "now" 7 uH primary. Here's what it should now look like: -

^{simulate this circuit – Schematic created using CircuitLab}

If the turns ratio wasn't 1:1 (i.e. both coupled inductors were not identical in value) then it becomes a little trickier because you have to impedance transpose the components on the secondary (including the new secondary inductor due to leakage) before you can connect them across the primary inductor.

This wiki page shows how a mutually coupled arrangement of inductors is equivalent to this: -

So if you start with L1=10 uH as per my example and have 7 uH mutual coupling you end up with a common inductor of 7 uH and two teed off inductances of 3 uH as an equivalent model.