I understand that what's diffusion and what's drift current but what I don know is how I identify whether a semiconductor is diffusion or drift based? Let's say bjt MOSFET and diode as example. They all have diffusion and drift property for me which makes me confused
Thanks! That clears my doubts. but from my notes it says bjt is diffusion based which mKes me confuse and MOSFET is drift based. Can I know how we know which will dominant to the other?
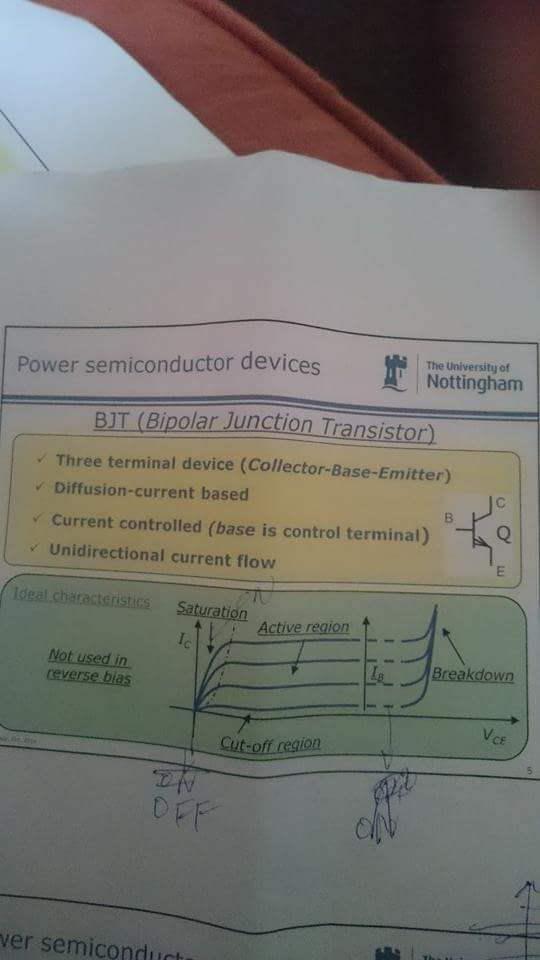
A semiconductor is not diffusion or drift-based, those are two phenomena always taking place in the same semiconductor. Considering electrons as carriers (but the same can be said for holes), the current density in a semiconductor can be expressed by the drift-diffusion transport equation:
$$ \vec{J}_n=q\mu_nn\vec{E} + qD_n\vec{\nabla}n $$ where \$q\$ is the elctron charge, \$\mu_n\$ the electrons mobility, \$n\$ the electron concentration and \$D_n\$ the diffusion coefficient. Thus, according to which term in the sum dominates the other you could say that a certain current is produced by diffusion or drift, but that's a matter of the ՝՝circumstances'', it's not the semiconductor which is diffusion or drift-based.
If you applied a strong electric field, the drift term would dominate; if no field is applied then the current is due only to the \$n\$ concentration gradient.
Hoping that what I said is clear enough, I want to make one final note: don't be fooled into thinking that without an electric field you would obtain a constant current depending only on how you doped your semiconductor; when electrons start to flow into the domain with less \$n\$ concentration, they ՝՝leave behind'' positively ionized atoms, which contributes in generating a potential difference and thus an electric field, until the diffusion term is compensated and there is no net flux of carriers.
The current flowing due to the concentration gradient is called diffusion.During the formation of barrier potential the p holes go to n side whereas the n electrons go to the p side to form immobile ions,this is due to concentration difference.Thus BJT is a device which is current controlled and current is duffusion.Whereas Mosfet as we know works on the variation of Vgs which is voltage controlled and there is no such thing as concentration gradient in this case.Whatever carrier current is due to majority charges which flow under the attraction of potential applied ie the drift current.
