In an op-amp (non-inverting), 
when \$V_s\$ increases by \$\Delta V\$, \$V_n\$ approaches \$V_p\$ until the difference is \$\Delta V/ A\$ (where A is infinity).
Why is there the \$\Delta V/ A\$ difference and where does this difference come from? Why doesn't \$V_n\$ just equal \$V_p\$ (so that the difference is 0)?
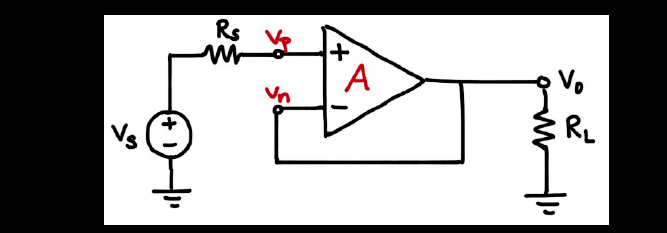
Because the opamp has finite gain. Note that in your equation when A is infinity, Vp and Vn are equal.
Since the opamp has finite gain, it needs some small difference between Vp and Vn to produce a non-zero result. For example, let's say the opamp gain is 100,000 and is producing 7 V out. That means it must see a difference of (7 V)/100,000 = 70 µV to produce that 7 V out.
We can usually consider the two inputs to be the same voltage and ignore a small difference like 70 µV. Usually the input offset voltage swamps that anyway. There isn't much point considering 70 µV difference when the input offset voltage is 2 mV. That means that the actual voltage difference between Vp and Vn can be up to 2 mV off from what it should be ideally. Basically, the input offset voltage is the error inside the opamp when interpreting the difference between its inputs. In this example, the additional offset of 70 µV to make the opamp produce its actual output is only 3.5% of the ambiguity in the difference between the inputs anyway.
It's pretty much by definition. The output is the differential input voltage times the gain (A). Therefore the differential input voltage is the output voltage divided by the gain.
The feedback drives the negative input to very close to the positive input due to the very large gain, again so Vin = Vout/A. If A is infinity as in an ideal op-amp then there's no difference between the 2 inputs. (Or you could say the input voltage is vanishingly small.)
In a non-ideal amplifier the feedback drives the input voltage to a value just large enough to satisfy Vout = A*Vin.
