Determining Voltage gain in a BJT amplifier

Question

I've been trying to solve the ac small signal analysis of this BJT amplifier for a while.

The question asks for the following:

Q.4: For the BJT circuit (Figure 4), the signal source generates ac signal with zero DC. The transistor has \$\beta\$ =100, and ro =20 k ohms.

(a) Find RE to establish a DC current of IE= 0.5 mA. Assume VBE=0.7 V for conduction.

(b) Find RC to obtain VC = 5 V.

(c) Determine the system voltage gain with RL = 10 k ohms.

I was able to solve part a and b. I obtained RE = 28.85 kohms and RC = 20.2 kohms. But, I can't seem to solve part c. I used the t-model to try to solve for the gain. I considered Re to be just internal resistance of the amplifier. That is, re= \$ \frac{V_t}{I_e} \$ and I disregarded the previously found value for RE in part a (because of the bypass capacitor in parallel to Re). I eventually got to the answer \$ A_v \$= -99.2 v/v but the answer given by my teacher was \$ A_v \$=-66.26 v/v.

I've been trying for a couple of hours ( :( ) but I can't seem to be able to solve it. Any ideas?

Answer 1

The only thing you seem to be forgetting is that the BJT gain is not the system gain. System gain is Vout / Vsig. But the BJT gain must be applied to the base voltage, not to Vsig.

You yourself computed the BJT gain as around 99 V/V. I am not double-checking that. Hopefully it is correct. But the base voltage can be computed using the votlage divider rule. First, we need Rpi, the resistance looking into the base. That is given by re * beta. Beta is given as 100, and re can be computed using Vt/Ic = 52. So Rpi is 5200.

The way I was taught to solve these problems is to view them as a series of gains which are multiplied together. The gain of the voltage divider formed by Rsig and Rpi is Rpi / (Rsig + Rpi). That is 2500 / (2500 + 5200) = 0.67. The gain from base to collector is -99.2 (per your calculation).

So the system gain is Vout/Vsig = 0.67 * -99 = -67 V/V.

Hope that helps. Let me know if I am wrong about anything.

Answer 2

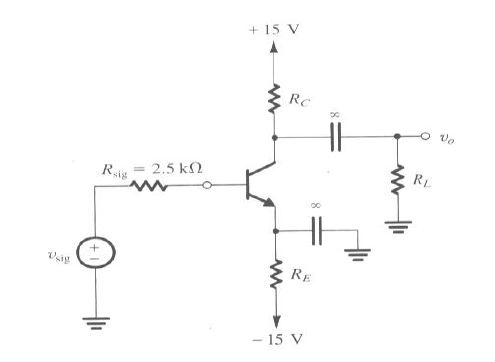
The schematic follows here:

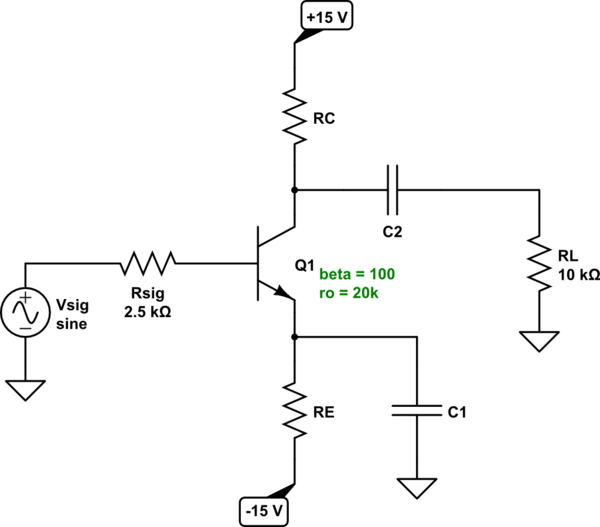
^{simulate this circuit – Schematic created using CircuitLab}

---

For part (a) you know to consider the signal voltage as \$0\:\textrm{V}\$ (quiescent) for the purposes of computing the value of \$R_E\$. I assume you know how to perform the usual KVL loop from the signal source to the \$-15\:\textrm{V}\$ source, as:

$$V_{sig}+I_B\cdot R_{sig}+V_{BE}+I_E\cdot R_E + \left(-15\:\textrm{V}\right)=0\:\textrm{V}$$

From that, and knowing that \$I_E=\left(\beta+1\right)\cdot I_B\$, it's easy to find:

$$\begin{align*} R_E&=\frac{15\:\textrm{V}-V_{sig}-\frac{I_E}{\beta+1}\cdot R_{sig}-V_{BE}}{I_E}\\\\ &=\frac{15\:\textrm{V}-\frac{500\:\mu\textrm{A}}{101}\cdot 2.5\:\textrm{k}\Omega-700\:\textrm{mV}}{500\:\mu\textrm{A}}\\\\ &= 28.5752475\:\textrm{k}\Omega \end{align*}$$

So I believe your teacher is right about this detail. \$R_E\approx 28.575\:\textrm{k}\Omega\$.

---

For part (b), you know the collector current is \$I_C=I_E\cdot\frac{100}{101}\approx 495\:\mu\textrm{A}\$. You also know that this collector current must drop \$10\:\textrm{V}\$ across \$R_C\$, so \$R_C=\frac{10\:\textrm{V}}{495\:\mu\textrm{A}}=20.2\:\textrm{k}\Omega\$, as you already surmised.

---

For part (c), you are now dealing with the AC voltage gain. What first bothered me with your given situation is \$r_o=20\:\textrm{k}\Omega\$. That's a crazy low number. Let's take the DC quiescent point and follow this logic through. We know that \$r_o=\frac{V_A+V_{CE}}{I_C}\$, but this means that \$V_A=I_C\cdot r_o-V_{CE}=495\:\mu\textrm{A}\cdot 20\:\textrm{k}\Omega-5.7\:\textrm{V}=4.2\:\textrm{V}\$. (Which is pretty much insane. I don't buy it. But I suppose it must be accepted as given.)

I have to assume some value for \$V_T\$, so I'll assume \$V_T\approx 25\:\textrm{mV}\$ for the following. Knowing that \$g_m=\frac{I_C}{V_T}\$ and \$r_\pi=\frac{\beta}{g_m}\$ and \$r_e=\frac{1}{g_m}\$ then the total gain should be:

$$\begin{align*} \vert A_V\vert &=\frac{r_\pi}{r_\pi+R_{sig}}\cdot\frac{R_C}{r_e}\cdot\frac{r_o}{r_o+R_C}\cdot\frac{R_L}{R_L+\left(r_o\vert\vert R_C\right)}\\\\ &=\frac{\frac{\beta}{g_m}}{\frac{\beta}{g_m}+R_{sig}}\cdot\frac{20.2\:\textrm{k}\Omega}{\frac{1}{g_m}}\cdot\frac{20\:\textrm{k}\Omega}{20\:\textrm{k}\Omega+20.2\:\textrm{k}\Omega}\cdot\frac{10\:\textrm{k}\Omega}{10\:\textrm{k}\Omega+\left(20\:\textrm{k}\Omega\vert\vert 20.2\:\textrm{k}\Omega\right)}\\\\ &=\frac{100}{100\cdot \frac{V_T}{I_C}+2.5\:\textrm{k}\Omega}\cdot 20.2\:\textrm{k}\Omega\cdot\frac{20\:\textrm{k}\Omega}{20\:\textrm{k}\Omega+20.2\:\textrm{k}\Omega}\cdot\frac{10\:\textrm{k}\Omega}{10\:\textrm{k}\Omega+\left(20\:\textrm{k}\Omega\vert\vert 20.2\:\textrm{k}\Omega\right)}\\\\ &\approx 66.385 \end{align*}$$

Close.

I still can't stomach the \$r_o\$ value you have. But that's what works out in the end, if you accept it as valid.