How do op-amp output add to/substract from the input voltage in postive/negative feedback?

Question

Unlike for current, I don't understand how voltages add up or cancel each other.

^{simulate this circuit – Schematic created using CircuitLab}

With negative feedback, Vout will try to make V- and V+ equal but 'taking away' or 'adding' voltage to the inverting input.

You have this essential equation for op-amp: $$V_{out}=A_0(V_+-V_-)$$ Because the output is connected to the inverting input, you now have: $$V_{out}=A_0(V_+fV_{out})$$

$V_{out}$ can be less of more than $V_{-}$.But my question is how does Vout does take away or add to the V- to make it equal to V+.

Answer 1

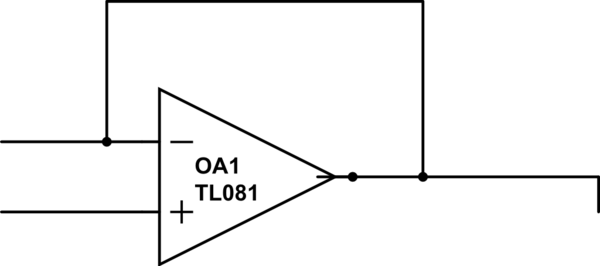
If and only if the op amp's output voltage \$V_{out}\$ is the only voltage source that is driving (connected to) the op amp's inverting input \$V_{-}\$, then via some simple algebraic manipulation of the equation \$V_{out} = A_{0}(V_{+}-V_{-})\$ we have,

$$ V_{out} = A_{0}(V_{+}-V_{-})\\ = A_{0}V_{+}-A_{0}V_{-}\biggr\rvert_{V_{-}=V_{out}} \\ = A_{0}V_{+}-A_{0}V_{out} \\ \Rightarrow V_{out}+A_{0}V_{out}=A_{0}V_{+} \\ \Rightarrow V_{out}(1+A_{0})=A_{0}V_{+} \\ \Rightarrow V_{out}=\frac{A_{0}}{1+A_{0}}V_{+}\biggr\rvert_{A_{0}\gg 1} \\ \Rightarrow V_{out}\approx V_{+} $$

In this case, \$A_{0}\$ is the op amp's open loop voltage gain. At low frequencies (close to DC) and for a "typical" op amp, \$A_{0}\$'s value might be in the range \$10^{5}-10^{6}\, V/V\$, in which case \$A_{0}\gg1\$ and the approximation result \$V_{out}\approx V_{+}\$ is valid.

Note that if two different voltage sources—e.g., \$V_{X}\$ and \$V_{out}\$—are directly connected to the same circuit node—e.g., the op amp's inverting input terminal \$V_{-}\$, the two voltage sources will fight each other for control of the voltage at that node. At the very least the system will be unstable, and it's quite likely one or both of the voltage sources will eventually fail catastrophically.