Confusion about positive feedback condition in gain equation

Question

Closed loop gain A' of the above op amp system is given as:

A' = A / (1 + β*A)

where A is the open loop gain which is a positive huge number.

Here as a side note, my understanding is that β being positive means β doesn't cause any phase shift so this causes subtraction hence negative feedback. And I assume β being negative means β causes 180 degrees phase shift and causes positive feedback(subtraction becomes addition at Σ).

But in a text I encounter the following:

Condition for negative feedback: |1 + β*A| > 1

Condition for positive feedback: |1 + β*A| < 1

So if the above conditions are correct, does that mean that even the β causes 180 degree shift, it is not enough to create positive feedback? I mean |1 + β*A| can still be greater than 1 even β is negative.

Which one is correct?

1-) β being negative causes 180 degree phase shift so there is positive feedback

2-) Condition for positive feedback: |1 + β*A| < 1 . β being negative alone is not enough for positive feedback salutation.

Here is the text where I encountered these conditions:

Answer 1

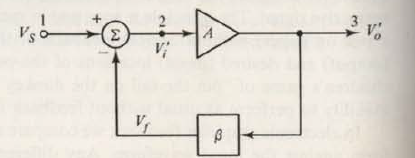
Here's my take on it, those conditions are wrong when it comes to defining if the feedback is positive/negative, so

1-) β being negative causes 180 degree phase shift so there is positive feedback

For this block diagram, if \$A\$ is considered the plant, the feedback is negative if \$\beta>0\$ (doesn't mean it will stabilize the plant/loop or anything). If the plant is something after \$A\$ (an unitary gain block?), then the feedback is negative for this block diagram if \$A\beta>0\$.

2-) Condition for positive feedback: |1 + β*A| < 1 . β being negative alone is not enough for positive feedback saturation.

For the loop to oscillate, you need the meet the Barkhausen stability criterion (and only simple poles), where \$|A\beta|\ = 1\$ and also (in this case due to the negative sum junction) you must have either \$A < 0\$ or \$\beta < 0\$.

Also, the book states three things in this page that are particularly confusing.

In the Eq. 9.3 the feedback voltage \$V_f\$ is presented to the input circuit in subtractive fashion. The denominator \$|1+A\beta| > 1\$ and the feedback is negative. Equation 9.5 then shows that \$|A'|<|A|\$ and the gain of the system with feedback is less than the internal amplifier gain. Thus gain is sacrificed with negative feedback.

I read the paragraph as, if \$|1+A\beta| > 1\$ and feedback is negative (\$\beta > 0\$, since the picture shows it just multiplies the output and goes to a negative sum junction), then \$|A'|<|A|\$. Or logically,

$$|1+A\beta| > 1,~ (\beta>0)^* \Rightarrow |\frac{A}{1+A\beta}| =|A'|<|A|.$$

*Notice this is useless to his proof, removing this premise doesn't break the conclusion. It is probably mentioned as it is common to have negative feedback systems where \$\beta>0, ~A>0\$

If \$A\$ is negative, as is usual in C-E amplifiers, we reverse \$V_f\$ from the \$\beta\$ network, resulting in a positive \$A\beta\$ term in Eq. 9.5 and so retain the negative feedback.

I suppose it mean, if \$A<0\$ we need \$\beta < 0~\$ ("... we reverse Vf from the β network") to keep \$|A'|<|A|\$. Or logically,

$$A<0,~ \beta<0 \rightarrow |1+A\beta| > 1 \Rightarrow |\frac{A}{1+A\beta}|=|A'|<|A|.$$

Finally,

If the phase of \$V_f\$ reverses, as may happen with nonresistive \$\beta\$ networks, the feedback voltage \$V_f\$ becomes additive to \$V_s\$ in Eq. 9.3 and the denominator of Eq. 9.5 shows that \$|1+A\beta|<1\$ and the feedback is positive. The closed-loop gain is \$|A'|>|A|\$ and the gain of the feedback system is greater than the internal gain.

Having \$\beta<0\$ and \$|1+A\beta|<1\$, we have that \$|A'|>|A|\$. Also, logically,

$$ (\beta<0)^*,~ |1+A\beta|<1 \Rightarrow |\frac{A}{1+A\beta}|=|A'|>|A|.$$

*Also useless to the conclusion, but probably used to hint that \$A>0\$.

Answer 2

I'm sure you'll agree intuitively that positive feedback will increase the gain thus |1 + AB| must be < 1 so that |A/(1+AB)| > |A|. Similarly, we can argue that negative feedback will decrease the gain thus requiring |1 + AB| > 1.

The derivations of these formulas assume the systems are Linear (https://en.wikipedia.org/wiki/Linear_time-invariant_theory).The examples you gave that seem to violate this, are cases where the loop gain is so large linearity is no longer satisfied. For example with B = -10 and A = 10000 you find that the closed loop gain is negative, this doesn't make much sense if the open loop gain is positive and if we consider values of AB between 0 and -1 we find the closer to -1 we are the larger the gain gets, yet it is still positive. Then at -1 the gain goes to infinity. Past this point, we can no longer apply those formulas, hence the contradiction.

For more information see, Positive feedback and unstability

Answer 3

I wouldn't say you are missing something, but the book is certainly not giving you the full picture.

"Feedback" is a complicated topic, and it can't be reduce to "\$a\beta > 1\$ + positive feedback = instability" this has been know for almost 100 years, but people operates with oscillators from a lot before control theory was developed, so in order to explain instability they find that \$a\beta = 1\$ means oscillation for a given frequency, so this should be a "boundary" between stability and instability. This is wrong, the only stability criterion is the nyquist one.

But why the book is saying what is confusing you? As I said earlier control theory is not trivial, there are books fully dedicated to explain the basics of it, so a circuit oriented book can't explain everything. nevertheless, for most introductory practical applications the Barkhausen Stability Criterion, while wrong, still applies. This means that the circuit will be unstable if \$a\beta >1\$ and you have positive feedback, so there wouldn't even exist a transfer function since \$V_{out}\$ would go to infinity in no time.

I suggest you to understand what the book tries to tell you, that is how the fundamentals parameters of an amplifiers circuit (input and output impedance, gain, etc..) change with feedback and if you want to really understand how stability works, read some specialised book on the topic.

That being said, i would address your question directly.

Which one is correct?

1-) β being negative causes 180 degree phase shift so there is positive feedback

2-) Condition for positive feedback: |1 + β*A| < 1 . β being negative alone is not enough for positive feedback salutation.

option 1) is the correct in control theory, but as I said for this case, the transfer function wouldn't be defined.