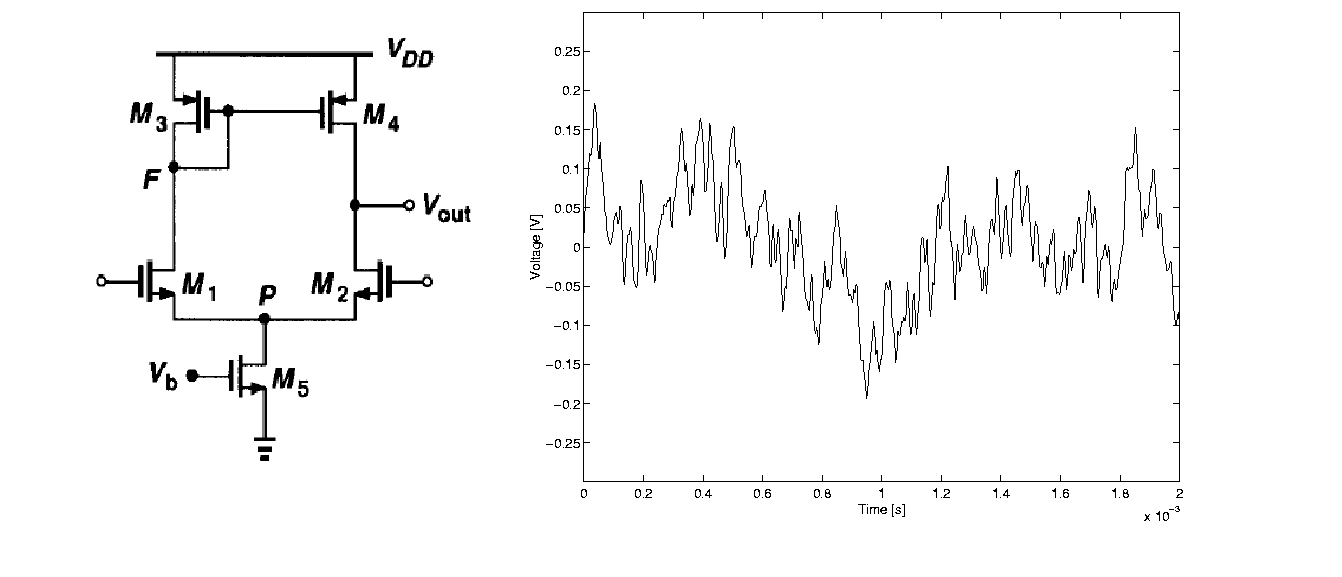
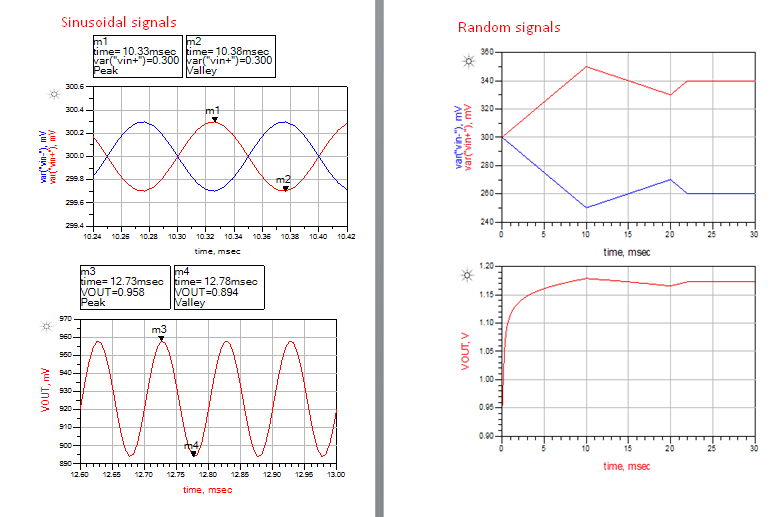
Deferentially amplifying a random signal is identical amplifying a sinusoidal one essentially, but with a complication. Output = gain (in1 - in2) as long as the inputs fall within the amplifier's input range. The output will simply be the difference between them, so just as random.
Therein lies the problem you'll face. Specifically, determining the gain you want. It's very hard if the signal is random such as white noise. If the input signals are 1Vp-p, you will not get 2Vp-p with a gain of 2. The amplifier's gain bandwidth product /slew rate complicates the calculation very much. The amplifier ends up amplifying some of the component frequencies, whilst attenuating others. It's a complicated non linear trade off.
Anecdotally, doubling the amplitude of 1Vp-p noise requires a TL082 op amp to have a gain of ~4. I've never seen a gain formula that applies to random signal amplification, and gain is only determined either by simulation or experimentation.
You will find that even with a unity gain, it is likely that a very broadband random signal will come out with a lower peak to peak than it went in with. This is the effect of frequency attenuation due to gain bandwidth product /slew rate limitation. This may matter or not to you, depending on your use of the signal. But if for example you're looking to maximise range on an analogue to digital converter, the gain calculation becomes important.