Resistance of a heating element

Question

Would a heating element have a very high resistance, or a very low resistance? (All comments in this post are based around the fact that the voltage is the same for each situation) I would have thought that a higher resistance would have resulted in more heat loss, but I've been taught that the higer the current, the more energy is lost to heat. Therefore, a lower resistance would release more heat.

Answer 1

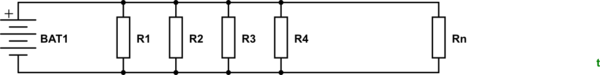
^{simulate this circuit – Schematic created using CircuitLab}

Figure 1. Does adding more resistors increase or decrease the total heat produced?

I would have thought that a higher resistance would have resulted in more heat loss ...

• It should be intuitive that the more parallel resistors we apply to the circuit of Figure 1 the lower the resistance becomes.
• Given a constant voltage as specified in your question it should also be intuitive that the current through each branch will be the same no matter how many branches.*
• We can then see that with n parallel resistors the total power dissipated will be n times the power dissipated with one resistor.

Therefore a lower the resistance value will result in more power dissipation or heat loss.

Mathematically this can be seen from the power equation \$ P = \frac {V^2}{R} \$ that, for a given voltage, power dissipated is inversely proportional to the resistance.

---

* A real power supply will, of course, have a limit to how much current it can produce before the voltage starts to droop.

Answer 2

Heat output is defined by the power \$P\$ which is itself defined by the voltage drop \$V\$ across the element and the current \$I\$ through it: \$P = V*I\$.

If you have a specific heat output you want and a input voltage you can figure out the resistance needed by plugging in Ohm's law.

\$P = V*A = \frac{V* V}{R} \$

So decreasing the resistance increases the heat output.

Answer 3

It depends:

• if it is connected to an ideal constant voltage source: lower load resistance will cause higher load power
• if connected to an ideal constant current source: higher load restance will cause more load power.

Often practical power sources can be treated like an ideal constant voltage source with a (rather low) internal series resistance. In that case most load power is caused by a load resistance that is equal to the the internal series resistance of the power source.
This fact is called the Maximum Power Transfer Theorem.

Answer 4

To further confuse things, perhaps shed more heat than light, if you have a nominally constant voltage source with a fixed source resistance there will be a load resistance that has a maximum power. Note that usually that's way lower resistance than what you would use (say) on the mains.

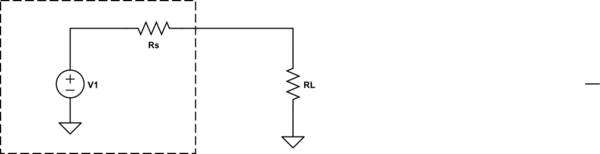
^{simulate this circuit – Schematic created using CircuitLab}

In the above circuit, the current is V1/(Rs+RL), so the power in the load is:

\$P_L = \frac{R_L\cdot V_1^2}{R_S+R_L}\$

You can see intuitively by inspecting the numerator and denominator that if RL is very low or is very high the power approaches zero.

In fact it is a maximum at \$R_L = R_S\$, where the load resistance equals the source resistance. Half the power is lost in the source resistance.

More generally, maximum power transfer is when source impedance equals load impedance.

Answer 5

A heating element has neither "very high" nor "very low" resistance.

The total energy dissipated by the circuit is proportional to current, so the resistance of the heating element has to be low enough to draw sufficient current to generate enough heat.

However, of the total energy dissipated by the circuit, the portion of the energy dissipated by each part is proportional to its resistance, so the resistance of the heating element has to be high enough so that most of the energy is dissipated by the heating element itself instead of, for example, the wiring in the walls.

If you're connecting a heating element to the wall mains, there is a circuit breaker involved that limits the current so that your wiring doesn't get too hot. A heating element designed to deliver maximum heat (in a kettle, for instance) will draw as much current as it can while staying safely below that limit.

Answer 6

It depends on the power source. If that offers reasonably constant voltage, as most do, then lower resistance increases the current, which increases the power dissipation and thus the heat.

As heating usually takes a lot of power (compared to electronics) it usually needs a pretty good power supply, like a large lead acid or Li-Ion battery if it's portable - and those are reasonably good voltage sources.

So if you have some means of control - like PWM, or a thermostatic on-off switch, err slightly on the low side of resistance to get slightly more power than you need, and regulate that power to get the right temperature.

If you had a good constant current source, then increasing resistance would increase the voltage, and that would increase power. But those are pretty rare in practice.

Answer 7

Do you want high or low resistance?

It depends on you power source. If you want heat you want power and power is

$$P = I \cdot V = I^2 \cdot R = \dfrac{V^2}{R}$$

So if you have a constant current source you want high resistance. However, most heaters are supplied with a constant voltage so would require a lower resistance.

If the power source is AC remember to use the RMS figure for the current or voltage as appropriate.

Answer 8

It depends where are your biggest problems in powering that heater.

If you have problems with resistance of the supply (eg. long or thin wires, high internal resistance) then you go for high resistance, high-voltage, low-current option.

If you have problems with insulation (eg. there is not enough room for thick insulation or the heater cannot be well insulated from potential users touching it) then you go for low resistance, low-voltage, high-current setup.

It's a balance between those two. In reality, you go for the voltage that you have at hand (eg. older trams use heaters connected directly to line voltage, be it 600V, 800V, or any other voltage the rest of the tram runs on. More modern ones utilize off-the-shelf 220V heaters, because today it's cheaper to design voltage converter than to design new heater). The pretty much only exception is when you need to safeguard against touching, then you drop the voltage down to safe level and work with that.

Answer 9

Don't know if this helps but I just put my multimeter on a 220-240V 1850-2200W kettle element and got ~27 ohms.

Ps electronics is not my strong point