I want to obtain the inductance value from a coil. To do so i'm using a basic RL circuit as follows. The function generator has a 50 Ω internal resistance and the coil resistance is 87 Ω. The coil has a small sticker with it's value (6,24 mH) supposedly provided by the manufacturer. I would like to prove it experimentally to be sure that it's correct.
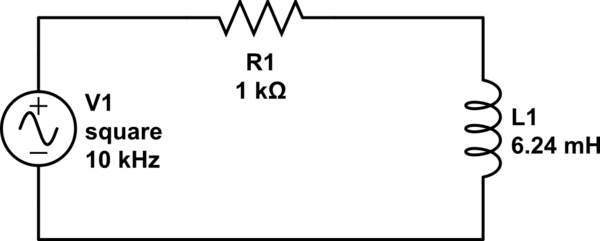
simulate this circuit – Schematic created using CircuitLab
As we know the voltage that goes through the coil tends to 0 following this expression: \$V_{L}(t)\$=V·\$e^{-t/τ}\$, where $$τ=\frac{L_{1}}{R}$$
What I was doing with the oscilloscope was taking the difference of time between the signal rising start and the moment where the exponential is the 36.79% of the initial value. Then considering this number τ and knowing R value I calculated L1 (L1=R·τ). The result is not the expected and I firmly believe that this method is not correct at all.
What I tried next was similar, taking two points but this time the first one is a little bit after the maximum voltage and the second the 36.79% of the first one. Now with the time and voltage difference between the two instants and the expression seen before I calculated $$L_{1}=\frac{-(t_{2}-t_{1})·R}{ln(\frac{V_{L}(t_{2}-t_{1})}{V})}$$ Again the result is not correct.
I would like to know what i'm doing wrong.
Anyway if you know a different and simple way to measure the inductance experimentally i'm open to sugestions.
Thanks!

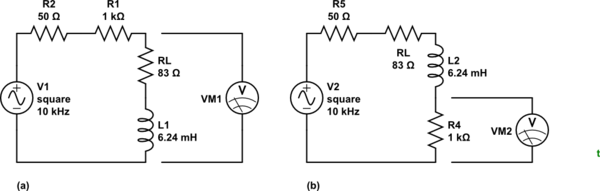
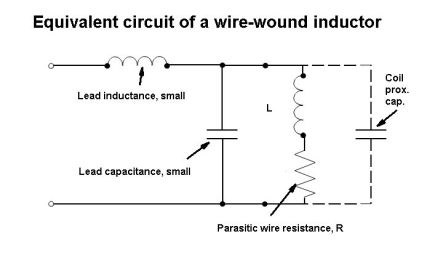
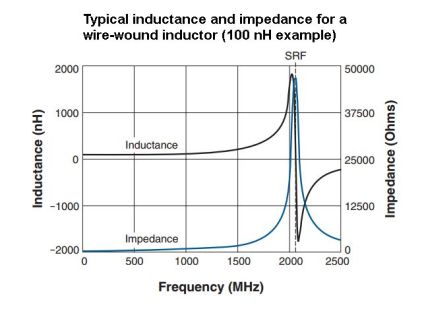
\$to add inline equations and$$for larger "on-their-own-line" equations. See electronics.meta.stackexchange.com/questions/5565/… for a cheat sheet of sorts. \$\endgroup\$