I have the following question:
- drew a vector diagram with the known voltages:
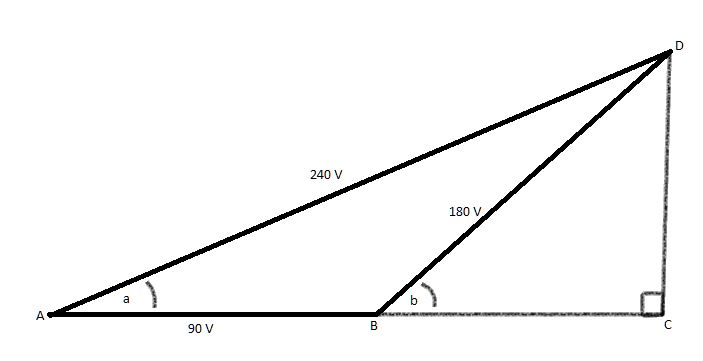
- used the vector diagram to come up with 2 equations using pythagoras and used them to solve for BC and CD
- calculated coil impedance from 180 V and 2.4 A and used that as the "resistance" part
- calculated XL using the value I calculated for CD
- calculated the phase difference using sin (VL/180), where VL is CD
My final answers are BC = 38.3 V; CD = 175.88 V; "R" = 75 ohm; XL = 73.28 ohm; phase difference = 77.72 deg.
My 2 equations for BC and CD:

I'm pretty sure I went wrong somewhere but I can't figure out exactly where I went wrong. I THINK its in the calculation of CD, I can't remember where I got the 125 from. Any help would be appreciated!