Below is a wikipedia section about reflection coefficient in electrical engineering:
source: https://en.wikipedia.org/wiki/Reflection_coefficient
It says that the incident to reflected wave ratio is complex. What does it indicate in practice here being complex?
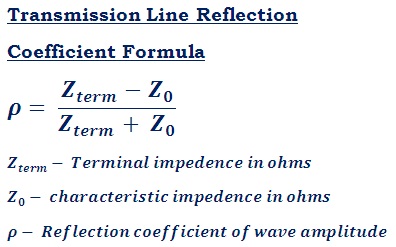
There are no such physical things as complex currents, voltages or electromagnetic fields. Complex reflection factor simply presents the existence of phase shift between incident and reflected sinusoidal waves when they are measured or calculated as complex phasors at the same point and the reflection factor = phasor of reflected wave divided by phasor of incident wave. The observation point can be the end of the line or any other point on the line.
Actually in every case, when the wave reflects due the mismatch, the reflection factor phasor generally is complex . It's real in some rare (see NOTE1) points where the phase difference of the incident and reflected wave is N*180 degrees where N is positive or negative integer or zero.
NOTE1: those points where the reflection coefficient is real are placed at quarter of the wavelength intervals. One of them is at the end of the line if the load is resistive.
Sinusoidal voltages, currents or powers (or voltage, current or power waves) are often denoted in phasor form. In phasor form, they are represented by a complex number where the absolute value of the complex number represents the amplitude of the sinusoid and the angle the complex number makes with the real axis in the complex number plane represents the sinusoid's phase relative to an arbitrarily chosen reference phase.
To calculate what happens when an incident wave is reflected, you multiply the incident wave with the reflection coefficient. If the coefficient is not a real number, it means the reflected wave will have changes in it's phase compared to the incident wave (because a multiplication with a non-real number will change the angle the number makes with the real axis and thus its phase).
What does it indicate in practice here being complex?
It indicates that either the characteristic impedance (Zo) is complex (a la low frequencies in telecom applications) or the load (Zterm) is complex or some combination that makes the answer complex.
Besides meaning complicated (which this is), complex refers to complex numbers and complex algebra, a number system based on the "complex operator", the square root of -1. In the late 1800s, Oliver Heavyside invented both coaxial cable and the math to describe it - complex vector analysis. This is the heavy lifting of electrical engineering math.
The math world uses a lower case i (i for imaginary) as the symbol for the operator. In EE land, i already is used for current, so we use j for the operator.
https://en.wikipedia.org/wiki/Complex_number https://en.wikipedia.org/wiki/Oliver_Heaviside
it just means that the reflection coefficient can be represented as a complex number/quantity in the form :
a +jb or in polar notation using magnitude and angle.
It doesn't have any "physical" significance or so. Its just a mathematical tool to represent the nature of a quantity and simplify calculations. For eg: when we represent an impedance as a complex quantity, Z = a+jw , we can infer that a there is a real part which doesn't vary with input voltage (Resistance), and there is an imaginary part jw ( Reactance part due to inductors and capacitors), which can varies with input voltage frequency.
