How to calculate net force from a Lorentz Force Density in Magnets

Question

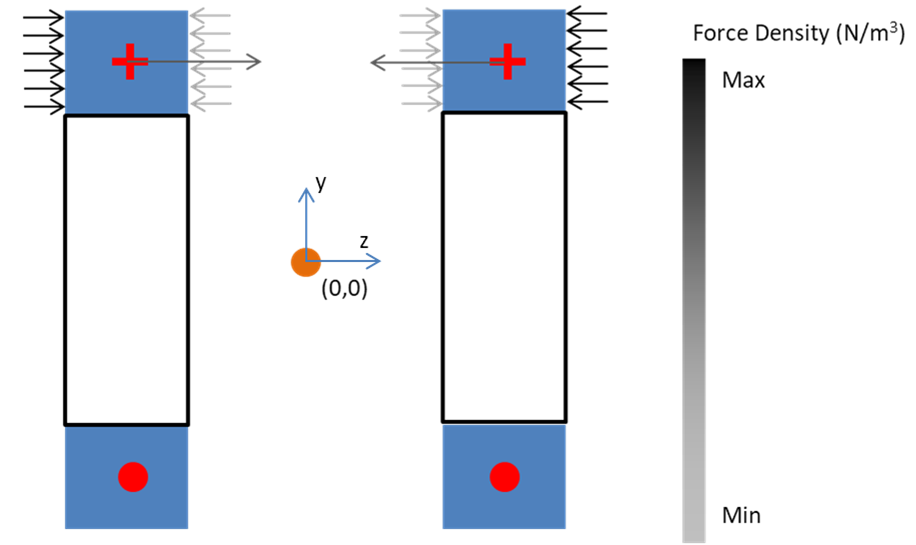
Consider a pair of solenoid magnets as shown in the figure. Based on the magnetic field (B) at each point and corresponding Current Density, (J), I can calculate the Lorentz Force density at each point as F = J x B. Since it is a solenoid (axisymmetric), if I consider, say YZ plane, I can get axial force density as Fz = Jx * By and radial force density as Fy = Jx * Bz for each discrete point in the coil.

From this Force density map( vectors at each point), how can one calculate the net force in each solenoid? My intuition was adding all the values in a face, and multiplying it with the area of the face will give the force in that face. If it is correct, how do I find the net force in the body? multiply it by 2 pi?

Answer 1

I had considered a standard solenoid from literature, where the final value from is known. The force density at different points was calculated and I could find out the method to correctly evaluate the net force.

If you have data at N points, your total area is A, R is the average radius, r is the radius at a given point, the net force can be given as,

\$ F =( \Sigma f ) \cdot 2 \cdot \pi \cdot R \cdot \frac{A}{N} \$

A bit more accuracy can be gained by

\$ F = \Sigma (f \cdot r) \cdot 2 \cdot \pi \cdot \frac{A}{N} \$

Answer 2

My intuition was adding all the values in a face, and multiplying it with the area of the face will give the force in that face

Yes, basically that's what you have to do.
The mathematical term for that is integrating the force densisty over the area and about since Leibniz's time it is written as

\$F = \int_A f da\$

(where \$f\$ is force density [unit force per unit area]; \$da\$ is the infinitesimal area element; \$A\$ is the total area and \$F\$ is the total force)
BTW: the \$\int\$ is to remind of Sum.

Answer 3

If you have calculated all the force densities in discrete points over the face and multiply with the face area, you still need to integrate along the solenoid's radial direction for the required volume integration.

Therefore, multiplying with 2 pi is not suficient, you have to multiply with 2 pi x radius, where radius is an averaged radius.

Assuming you numerically calculated the force densities in the points, and going through the volume integration, you get

radius = (rad_outer + rad_inner) / 2

with outer and inner solenoid radius.