Full wave rectifier math analysis

Question

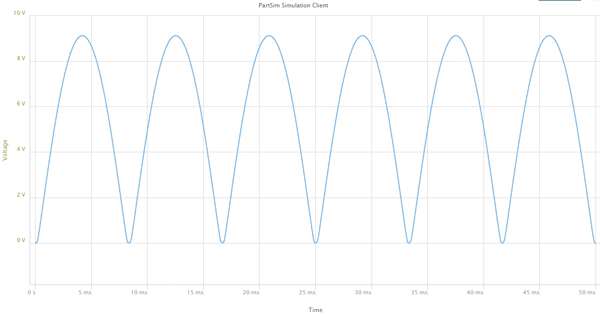
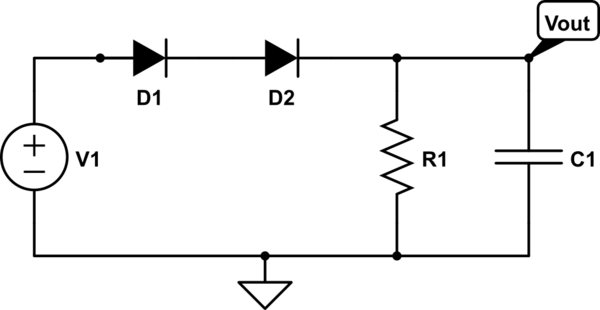
I read some about a full wave rectifier on this website and when it got to the section of smoothing capacitor, and showed this graph and schematics:

It was also said that:

Here the 5uF capacitor is charged to the peak voltage of the output DC pulse, but when it drops from its peak voltage back down to zero volts, the capacitor can not discharge as quickly due to the RC time constant of the circuit.

This got me thinking about the mathematical analysis of this circuit, because I wanted to know what the time constant is. I assume it is not T = RC like in a resistor-capacitor series circuit, but something else.

So I assume the circuit can be shown like this now:

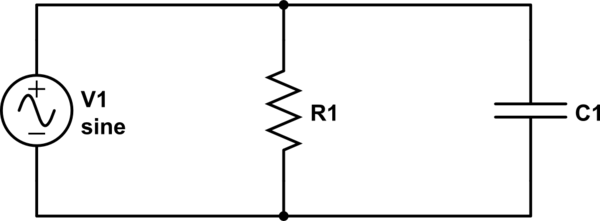
^{simulate this circuit – Schematic created using CircuitLab}

Only now V1 isn't really a sine function but rather this:

I have Googled for a the math here but I couldn't find much, the best thing I found was this, showing how to find the impedance or current in the circuit.

My questions are:

1. How can I describe the source (V1 in my circuit below), the output of the bridge rectifier, as a mathematical function?

2. What is the time constant here?

3. Here is something I'm very confused over. If the voltage is equal in a parallel circuit, aren't the waveforms supposed to be the same? How is the waveform of the voltage on the capacitor different from the one on the source (again, V1 in my circuit).

Thank you.

Answer 1

Your circuit can not be described as you think because a true voltage source would maintain the voltage and sink current as well as supplying it.

A better approximation would be.

^{simulate this circuit – Schematic created using CircuitLab}

This circuit has two diodes since when the bridge rectifier is conducting there are two diode drops.

\$ V_1 = |V_{pk} \cdot \sin(2 \pi f t)|\$ where \$ V_{pk} \$ is the peak voltage of the input AC, \$ \sqrt{2} \cdot V_{rms} \$

The time constant \$ \tau = R_1 \cdot C_1 \$ when the diodes are not conducting. Taking the peak voltage on C1 to be the peak voltage on V1 minus two diode drops and calculating the droop using half the period for the input AC will give you a slightly pessimistic estimate of the output ripple.

You can get a better estimate if you compare the voltage on V1 in more detail but the quick method is usually good enough for design purposes.

Answer 2

The four diodes make this circuit change paths where current flow during V1's cycle: you can roughly divide its operation into two different modes....

• one mode where the diodes connect V1 to the RC load

• the other mode where V1 is disconnected from the RC load.

Viewed this simplified way, diodes operate like switches. When |V1| < Vc, then |V1| is isolated by an open switch. This discontinuous operation means that you cannot apply a voltage source \$ |V1|_{(t)} \$ and use linear circuit analysis to find voltage across C1 and R1 - the diodes make it a non-linear circuit.

In this diagram, the two operating modes are described as C Charges and the other C Discharges. The diodes connect V1 to R,C only during the C Charges period.