Phasor vs Vector vs Space Vector

Question

I wonder what is the difference between a Phasor, a Vector and a Space Vector? A phasor is also a rotating vector in space then it means that a space vector is same as a phasor? Also by definition a vector has magnitude and direction in 2D-space.. so then a vector and space vector both are the same things. Please correct me if I am wrong in this understanding.

Answer 1

A space vector results from a mathematical transform of a three-phase system, which results in a vector in the complex plane. As time progresses, the vector moves around and draws its trajectory in the complex plane.

Example for a three-phase voltage system:

\$\underline{a} = e^{\frac{2 \pi}{3} j}\$

\$\underline{u}(t) = \frac{2}{3} \cdot [u_R(t) + \underline{a} \cdot u_S(t) + \underline{a}^2 \cdot u_T(t)]\$

If \$u_R(t)\$, \$u_S(t)\$ and \$u_T(t)\$ describe a pure sinusoidal system, the resulting space vector \$\underline{u}(t)\$, which is a time-dependent complex number, will rotate in the complex plane, and draw a circle there. Every deviation from the circle (e.g. current ripple if one looks at the space vector of inverter current) is related to a distortion in the time domain.

Space vector calculus allows optimization of PWM switching patterns, and allows visualization of complex modulation patterns.

Answer 2

A vector is a mathematical entity that we all know and love; it's a \$1 \times N\$ matrix of numbers that indicates a position in \$N\$ dimensional space.

A phasor, as @ThePhoton pointed out, isn't really a vector. It's a lot like a vector, but it's really a complex number denoting a sinusoidal current or voltage at some reference frequency that's had the reference frequency component removed. Thus, you can do arithmetic on it using complex number rules, not vector rules.

When you say "space vector" I believe you're talking about "space vector modulation". I'm really not sure where the space vector is in space vector modulation, but I suspect that for the time being, you'll confuse yourself by thinking too hard about the "vector" part.

Answer 3

Key difference: When we multiply two phasors, we get a result that is another phasor in the same 2-dimensional space. When we multiply two vectors we can either do a scalar product and get a scalar result, or a cross product and get a vector that's orthogonal to both the original vectors

A phasor is also a rotating vector

Because we don't use vector operations on a phasor, I think it's misleading to call a phasor a vector.

I don't know how a space vector is defined (and it doesn't seem to be a common enough concept for Wikipedia to have an article on it) so I can't address that part of your question.

Answer 4

a vector has two elements usually a direction and magnitude. Normally it points to a position in an N dimension space from another point, most of the time this other point is the origin so it just points to a position.

a phasor is a convenient way to express an imaginary number pair R+jX where R and X are the real and imaginary components respectively and j is \$ sqrt{-1}\$ as a module and an angle related to the origin.

now space vectors is not like a phasor or a vector even, it is a convenient way of showing an inverter scheme(make AC voltage from DC) for three phase systems. You got three rows of where you have DC voltage so you have you can call them ABC, you can connect each as plus or minus with switches so you got \$A_+ B_+ C_+\$ and \$A_- B_- C_-\$ now the vectors just indicate who is on and who is off as a state, but ultimately you are talking about multiple DC voltages being turned on and off, there are some rules to it.

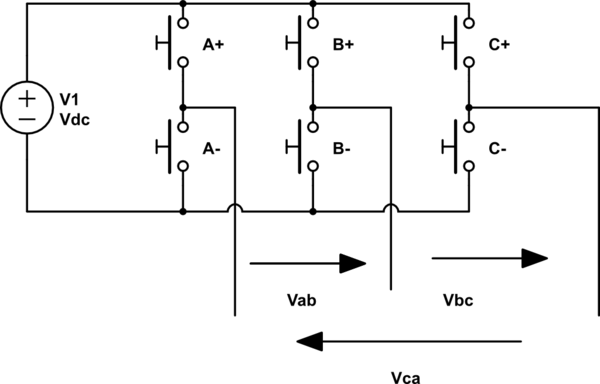
^{simulate this circuit – Schematic created using CircuitLab}

So no, a phasor and space vector(modulation) are not the same thing but they can be closely related.